Please consider this picture of a peptide chain:
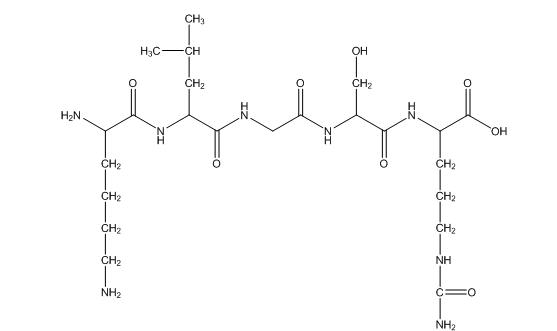
I've done some examining on the different amino acids and concluded that the chain is as follows:
Lys-Leu-Gly-Ser-Citrullin (variant of arginine).
I would now like to calculate the charge of this peptide at neutral pH.
I'm using this table to orient myself: http://www.sigmaaldrich.com/content/dam/sigma-aldrich/life-science/biochemicals/migrationbiochemicals1/common-amino-acids-table.jpg
So here's my train of thought:
As we're at neutral pH the +1 and -1 of the mandatory amino and acid groups will cancel out for all amino acids.
Lysine has a pKx of barely 11 which is some higher than 7 so it is basic in our solution. It means that it will accept a proton so Lysine has a charge of +1 in our respective solution.
Leucine, Glycine and Serine have no charge on their x group so their charge is 0 and does not contribute anything to the total charge.
Citrullin, I read, is uncharged at neutral pH, opposed to Arginine which is, so it does not contribute either.
So my total answer is +1. Is this correct? I am in no way 100% sure of my method but it would be great to get a confirmation that I am doing it correctly or to be corrected on my possibly faulty method.
Thank you!
