Since both compounds are of the form $\ce{AB}$ (and not $\ce{AB2}$ or $\ce{A2B3}$/etc), the coordination numbers will be equal for the ions. Similarly, for $\ce{AB2}$, the coordination number for $\ce{A}$ will be twice that of $\ce{B}$.
What happens is that each ion is connected to more ions of the opposite type in $\ce{CsCl}$. Each $\ce{Cs+}$ ion is connected directly to 8 $\ce{Cl-}$ ions, and vice versa. The net effect is that their ratio stays the same, they just have more connections.
Look at it this way: There are four men and four women in a room. First, each man holds hands with only one woman and vice versa. Now, we tell each man to hold the hands of two women, and we tell each women to do the opposite. Now, we shift it up to 3 hands (with the reasonable approximation that humans have an infinite supply of hands), and finally 4. Try this on a piece of paper -- you'll see that the man:woman ratio doesn't need to change as long as the "handholding number" (coordination number) for each person is the same.
Taking a look at the actual lattices:
For $\ce{CsCl}$ (body-centered cubic):

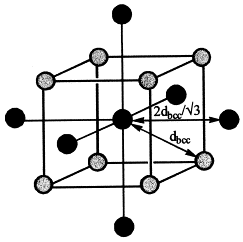
Note that each $\ce{Cs+}$ ion touches 8 $\ce{Cl-}$ ions and vice versa. Also note that the $\ce{Cs:Cl}$ ratio is indeed $1:1$. For each $\ce{Cs+}$ ion, there is a corresponding $\ce{Cl-}$ ion on its NE diagonal tilting into the plane of paper, and for each $\ce{Cl-}$ ion there is a corresponding $\ce{Cs+}$ ion on the SW diagonal tilting out of the plane of the paper.
Here's $\ce{NaCl}$ (face centered cubic, close packing):
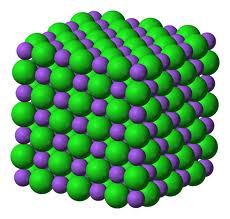
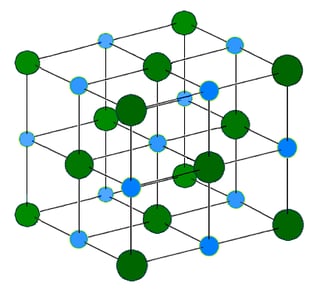
Here each $\ce{Na+}$ ion touches 6 $\ce{Cl-}$ ions and vice versa. We can define a similar pairing as before -- each $\ce{Na+}$ ion has a corresponding $\ce{Cl-}$ ion to its east, and each $\ce{Cl-}$ ion has a corresponding $\ce{Na+}$ ion to its west.




