TL, DR: They do not contradict. Although larger ions decrease the lattice energy by increasing ion distance, it is compensated by another factor that increases the lattice energy. And like Nisarg Bhavsar said, product formation does not solely depend on lattice energy. Other factors such as the electron affinity and ionization energy of the element should be accounted too.
From Ref. 1:
The answer has to do with the crowding of alkali ions around oxygen,
as illustrated for $ \ce{K2O}$ at the right. Because eight large $ \ce{K^+}$
ions surround each $ \ce{O^{2-}}$ ion in the structure, the cations
are essentially in contact. Indeed, the metal-oxygen bonds are
"stretched" in $ \ce{Na2O}$, $ \ce{K2O}$, and $ \ce{Rb2O}$ relative to
$ \ce{M-O}$ bonds with the same bond order in other structures.The
situation is so extreme for $ \ce{Cs2O}$ that it finds an
(electrostatically unfavorable) structure in which the coordination is
lowered to 6:3. This packing problem is relieved somewhat in the
peroxides, where the coordination is still 8:4 but the anion is
larger, and especially in the superoxides where the cation:anion ratio
is 1:1 and the coordination is 6:6. Thus the larger alkali ions ($
\ce{K^+}$, $ \ce{Rb^+}$ and $ \ce{Cs^+}$) tend to form superoxides.
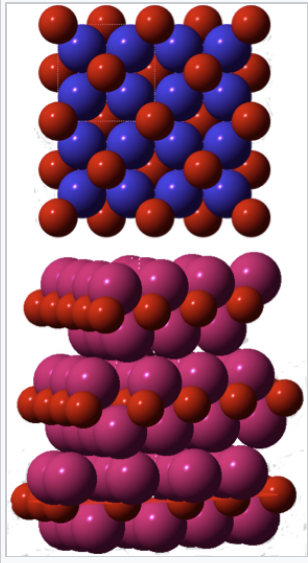
Above: $ \ce{K2O}$ structure, below: $ \ce{Cs2O}$ structure. Oxides are red, potassiums are blue and caesiums are magenta.
Thus, the reason why heavier alkali metals tend to form superoxides is because the stoichiometry of the compound and ionic radius of the anion allows the large cation to arrange so that they won't touch each other as much. Since like-charges repel, having cations in contact with each other is a crime in forming crystal lattices because it would greatly destabilize the crystal.
In case you don't know, the Born-Lande equation to calculate energy is actually derived from Coulomb's formula of electrostatic potential energy, i.e
$$E_\mathrm{pair} = \frac{z_1\cdot z_2\cdot e^2}{4πε_0r} $$
Where $z_i$ is the charges of one ion and $r$ is the distance between the point charges (ions in this case).
Specifically, assume a theoretical compound $\ce{M^{2+}X^{2-}}$ that crystallizes in the rocksalt ($\ce{NaCl}$) structure. The Born-Lande equation is derived from the sum of all electrostatic forces that one ion in the lattice experiences. Let's use the $\ce{M^{2+}}$ cation.
The rocksalt lattice has 6:6 coordination. It means that one $\ce{M^{2+}}$ cations is equally surrounded by six $\ce{X^{2-}}$ ions. Thus the expression for the electrostatic energy between the $\ce{M^{2+}}$ in question with the six $\ce{X^{2-}}$ surrounding it is
$$E_{1} = 6\frac{2\cdot (-2) e^2}{4πε_0 r} $$
The $\ce{M^{2+}}$ is also equally surrounded by twelve $\ce{M^{2+}}$ with a distance of $\sqrt{2}r$. Thus the expression for the electrostatic energy between the $\ce{M^{2+}}$ with the 12 $\ce{M^{2+}}$ surrounding it is:
$$E_{2} = 12 \frac{2\cdot 2 e^2}{4πε_0 \sqrt{2}r} $$
Etc. You can do another $\pu{E_{i}}$s of other sets of further ions surrounding the $\ce{M^{2+}}$ ion.
Adding all of this electrostatic energy equations together we get the sum of all the electrostatic energy on one particular ion:
$$E_\mathrm{all} = \frac{2\cdot (-2) \cdot e^2}{4πε_0 r} \cdot \pu{(6 - \frac{12}{\sqrt{2}}+...)} $$
The sum of $(6 - \frac{12}{\sqrt{2}}+...)$ in the equation is the Madelung constant. Since those numbers derive from the geometry and coordination number of the lattice, it is dependent on the crystal system rather than the nature of the ions, as you might have learned in textbooks.
Finally, combining this with the account for the electron-electron and nucleus-nucleus repulsions between the ions and multiply with the Avogadro constant since we are using $\pu{kJ/mol}$, we arrive at the Born-Lande equation:
$$E_{lattice} = \frac{N_A \cdot z_1 \cdot z_2 \cdot e^2 \cdot M}{4πε_0 r} \cdot ( 1-\frac{1}{d}) $$
As you can see, since the lattice energy involves the interaction forces of the ions, having too much repulsion would decrease it. The benefit of increased lattice energy from this factor is more than enough to compensate for the increased ion distance.
References:
The Strange Case of the Alkali Oxides. (2021, June 7). Retrieved June 11, 2021, from https://chem.libretexts.org/@go/page/189354
Energetics of Crystalline Solids- The Ionic Model. (2021, June 7). Retrieved June 11, 2021, from https://chem.libretexts.org/@go/page/183348

