How many rings does cubane have?

Question

Looking at the structure of cubane $(\ce{C8H8}),$ it appears as if the molecule were composed of six rings. However, numerous authoritative sources call the cubane a pentacyclic hydrocarbon. What is the reason for this?

Answer 1

You can always check the ring count by cutting the rings by imaginary scissors. The number of rings is equal to the number of the needed cuts.

Imagine cuban as two cyclobutane rings with all carbons interconnected by four bonds. Cut three of these connections to have just one remaining bond connected these rings. Plus another two cuts to break both rings.

So five rings in total.

Another approach could be watching the cube and seeing the sixth ring of the sixth side is excessive and rather just imaginary, adding nothing new. The whole cube is already constructed by five rings (the cube floor and four walls). The ceiling ring is not formed physically; there is no extra atom connection to close the sixth ring.

Yet by other words: The number of rings is not equal to the number of rings you can see, but to number of rings you have to make (and can cut) to build the structure.

Another, numerical approach, as suggested by @CuckooBeats for saturated hydrocarbons, is comparing the number of hydrogen atoms, compared with the respective n-alkane ($\ce{C8H8}$ versus $\ce{C8H18}$) and dividing the absolute difference by two ($\frac{18-8}{2} = 5$).

It can be generalized even for cyclic unsaturated hydrocarbons:

$$n_\text{cycles} = (2 \cdot n_\text{C} +2 - n_\text{H} - 2 \cdot n_\ce{=} - 4 \cdot n_\ce{#})/2$$

$$n_\text{cycles} = n_\text{C} + 1 - \frac{n_\text{H}}{2} - n_\ce{=} - 2 \cdot n_\ce{#}$$

or even with $\ce{N, O, S, X}$

$$n_\text{cycles} = n_\text{C} + 1 - \frac{n_\text{H}}{2} + \frac{n_\text{N}}{2} - \frac{n_\text{X}}{2} - n_\ce{=} - 2 \cdot n_\ce{#}$$

$n_\ce{C}$ - carbon atom count
$n_\ce{H}$ - hydrogen atom count
$n_\ce{N}$ - nitrogen atom count
$n_\ce{X}$ - halogen atom count
$n_\ce{=}$ - double bond count
$n_\ce{#}$ - triple bond count

Bivalent atoms $\ce{O}$ and $\ce{S}$ do not contribute to calculation.

General Rule for Counting Double Bonds:

• Include $\ce{C=C}$ and $\ce{C=O}$: These bonds contribute to the degree of unsaturation because they reduce the hydrogen count of the molecule.
• Exclude bonds like $\ce{S=O}$, $\ce{P=O}$: Double bonds involving hypervalent atoms (like sulfur and phosphorus) do not reduce hydrogen count in the molecule and hence do not contribute to unsaturation.
• Nitrogen ($\ce{C=N}$): Include because it behaves like $\ce{C=C}$ in terms of hydrogen count.

Answer 2

From a purely mathematical (more specifically, topological) viewpoint, one way to see that cubane has five rings is to consider the corresponding Schlegel diagram of the cube, which can be intuitively thought of as "flattening" a cube so that all atoms are in a plane, with no bonds crossing each other:

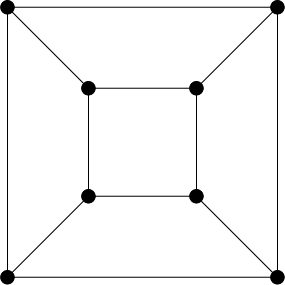(source)

From the Schlegel diagram, you can also easily perform the suggestion in Poutník's answer to count rings by counting how many bonds must be cut to get a ring-free structure. (For those who know graph theory, this is equivalently the number of edges to remove to get a spanning tree.)

The other mathematical way to see it is to use Euler's characteristic, $b-a+1$, where $b$ and $a$ are respectively the number of bonds and atoms. For the cube, we have $12-8+1=5$. (Note that we ignore H atoms and C-H bonds here for counting purposes.)

(See also Derek Johnson's notes, helpfully provided by user uhoh.)

Similar reasoning can be used to show that dodecahedrane has 11 rings, and the buckyball has 31 rings, to use more elaborate examples. ;)