8
$\begingroup$

Commonly known buckminsterfullerene has molecular formula C60 if we calculate it's degree of unsaturation by formulae, it comes out to be 61 and it is also known that this fullerene has 12 pentagon rings and 20 hexagon rings.

So, overall it has 32 rings. Also, degree of unsaturation can be thought of as number of pi bonds + number of rings for hydrocarbons, so the number of pi bonds calculated are 29 according to this concept

However, the number of pi bonds in fullerene have been found out to be 30. Why does degree of unsaturation not work for fullerene, what is it's actual degree of unsaturation?

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ Could you provide links as to where you are getting these "rules" from? My guess is that with fused rings you can forget them, but without seeing exactly how the terms you are using are defined its impossible to say. $\endgroup$
    – Ian Bush
    Commented Nov 30, 2023 at 14:13
  • $\begingroup$ It's not that your useless concept doesn't work, it's just that counting of rings is slightly more complicated. $\endgroup$
    – Mithoron
    Commented Nov 30, 2023 at 14:26
  • $\begingroup$ chem.libretexts.org/Bookshelves/Organic_Chemistry/…. degree of unsaturation is found using similar method for bicyclo compounds and the formula is given too $\endgroup$
    – worksifitdoes
    Commented Nov 30, 2023 at 14:27

1 Answer 1

Reset to default
10
$\begingroup$

An alkane with 60 carbon atoms has the formula $\ce{C60H122}$. To get the degree of unsaturation of fullerene, you have to successively add dihydrogen to it until you have an alkane. For each double bond turned into a single bond, you need one dihydrogen. For each single bond you break (to remove rings), you need one dihydrogen. Looking at the formula of fullerene, you will need 61 dihydrogen molecules to turn it into an alkane, so the degree of unsaturation is 61.

If a ring system has N rings, it does not mean you need to break N bonds to open them all up. Here is an example with 4 rings:

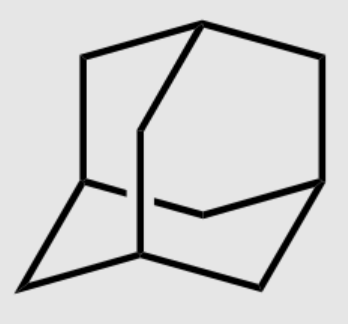
enter image description here

I only need to break 3 bonds to get a non-cyclic alkane:

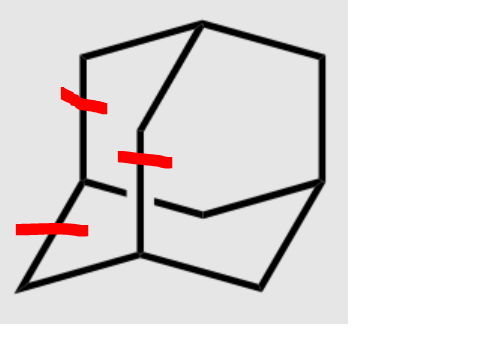
enter image description here

This is because the first cut breaks two rings at the same time.

EDIT:

This insightful comment made me think more about counting rings.

[Nicolau Saker Neto in the comments] Because C60 fullerene is so close to a sphere, I can't help but bring up this curiosity - the reason the formula is slightly off comes down to the fact that a 3D sphere missing even just a single infinitesimal point is topologically homeomorphic to a plane, but a complete 3D sphere is not, so there really is something special about that first cut.

If you contemplate the structure of naphthalene and norbornane, you will see that they are topologically equivalent. They both have two bridging carbons connected by three links. Yet most of us would probably count two rings for naphthalene and three rings for norbornane.

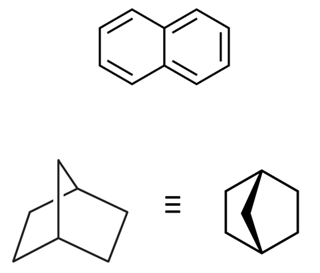
enter image description here

In the "flattened" view, it becomes clear why: In the top structure, we probably will not count the outer 10-membered loop, but in the bottom structure, we will typically count the outer 6-membered loop (and the two 5-membered rings). For the degree of unsaturation, you should only count "inner" rings in the flattened view of the molecule.

For fullerenes, this means counting one ring less, just as the analysis of breaking bonds to remove all the rings shows.

Improve this answer
$\endgroup$
6
  • 6
    $\begingroup$ Because $\ce{C60}$ fullerene is so close to a sphere, I can't help but bring up this curiosity - the reason the formula is slightly off comes down to the fact that a 3D sphere missing even just a single infinitesimal point is topologically homeomorphic to a plane, but a complete 3D sphere is not, so there really is something special about that first cut. $\endgroup$
    – Nicolau Saker Neto
    Commented Nov 30, 2023 at 21:19
  • $\begingroup$ @NicolauSakerNeto - I was trying to figure out how to express that in words. Thank you for putting it so clearly $\endgroup$
    – Andrew
    Commented Nov 30, 2023 at 21:44
  • $\begingroup$ @NicolauSakerNeto You can take a fullerene, grab one of the rings and expand it until the fullerene is flattened. When you do that, the ring you just expanded looks like the outer boundary of the ring system, which you wouldn't count usually. Here is an rough image of this, using a dodecahedron (C20): i.sstatic.net/zIA9j.png $\endgroup$
    – Karsten ♦
    Commented Nov 30, 2023 at 21:51
  • $\begingroup$ @Andrew The same for adamantane: i.sstatic.net/kdDA9.png (3 rings or 4?) $\endgroup$
    – Karsten ♦
    Commented Nov 30, 2023 at 22:13
  • $\begingroup$ Yes I understand that kind of drawing is possible. "Not counting the outer ring" is ultimately equivalent to taking an inflatable fullerene (or adamantane or dodecahedrane, etc.) wrapped in an elastic skin, squishing it against a plane to deform it into that shape, poking a hole in the front, then stretching the skin open and gluing the outer rim onto the plane. So you could say the act of planarizing it (which renders the unsaturation formula valid) "broke the outer ring" and you then decide not to count it. I can't seem to put in it better words. $\endgroup$
    – Nicolau Saker Neto
    Commented Nov 30, 2023 at 22:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.