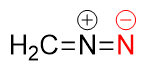I have a few questions about the terminal nitrogen (highlighted in red) in diazomethane, $\ce{CH2N2}$.
Is that nitrogen $\mathrm{sp}$ or $\mathrm{sp^2}$ hybridised?
What type of orbitals do the lone pairs on that nitrogen reside in?
Does the negative charge on nitrogen undergo resonance?
As usual with these small molecules, the bonding situation is a lot more complicated than expected. In this very molecule this is to the extend that not only the Lewis formalism, but also the conventional resonance formalism breaks down.
However, Jan's description is sound, and provides a good starting point for further analysis.
Unfortunately formulating a clear cut valence bond description is difficult, because it involves strongly coupled electrons. This is in part due to the high symmetry of the molecule, $C_\mathrm{2v}$, and the even higher local symmetry of the $\ce{CNN}$ moiety, $C_\infty$. Because of the nature of molecular orbital theory, i.e. being better at describing delocalisation, it is actually easier to understand.
Here are the molecular orbitals computed at the DF-M06L/def2-SVP level of theory:
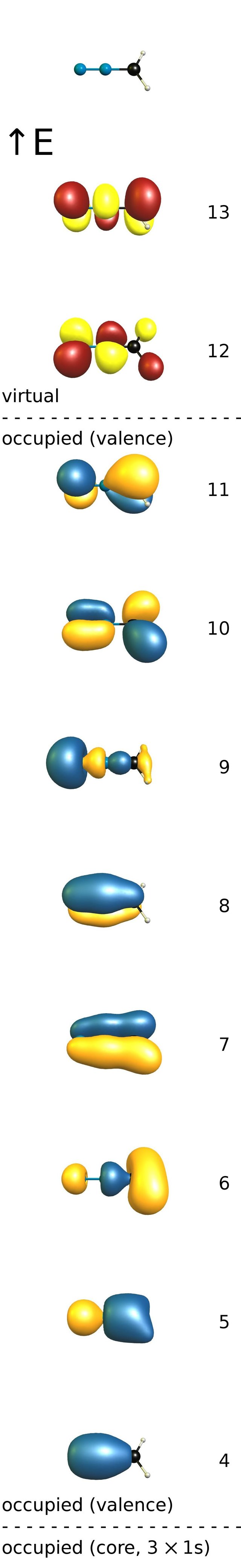
And here is the same thing in numbers
Atomic contributions to Alpha molecular orbitals: Alpha occ 1 OE=-14.323 is N2-s=1.00 Alpha occ 2 OE=-14.252 is N1-s=1.00 Alpha occ 3 OE=-10.099 is C3-s=1.00 Alpha occ 4 OE=-1.055 is N2-s=0.51 N1-s=0.29 N1-p=0.10 Alpha occ 5 OE=-0.802 is N2-p=0.31 C3-s=0.31 N1-s=0.14 N2-s=0.12 C3-p=0.07 Alpha occ 6 OE=-0.518 is C3-p=0.26 C3-s=0.24 H5-s=0.16 H4-s=0.16 N1-s=0.07 N2-s=0.06 Alpha occ 7 OE=-0.487 is N2-p=0.45 C3-p=0.24 N1-p=0.14 H5-s=0.08 H4-s=0.08 Alpha occ 8 OE=-0.450 is N2-p=0.61 N1-p=0.29 C3-p=0.09 Alpha occ 9 OE=-0.407 is N1-s=0.44 N1-p=0.41 N2-p=0.08 Alpha occ 10 OE=-0.366 is N1-p=0.28 C3-p=0.24 N2-p=0.16 H5-s=0.15 H4-s=0.15 Alpha occ 11 OE=-0.206 is C3-p=0.57 N1-p=0.38 Alpha vir 12 OE=-0.082 is N1-p=0.55 N2-p=0.32 H5-s=0.06 H4-s=0.06 Alpha vir 13 OE=-0.005 is C3-p=0.33 N2-p=0.33 N1-p=0.32
From the visual inspection we can see that the terminal nitrogen has only one orbital that could be classified as a lone pair, i.e mo 9; the hybridisation according to this calculation is approximately sp. There are three bonding π orbitals, i.e. mo 7, 8, 10. And there is also one anti-bonding π orbital, i.e. mo 11. The sigma bond is formed by two bonding (mo 4, 5) and one anti-bonding orbital (mo 6).
This is no coincidence, but pretty much always the case for terminal atoms.
Due to symmetry constraints, the hybridisation pattern at terminal atoms is usually:
Localisation of the orbitals is not easy for this compound. I performed a natural bond orbital analysis, which transforms the (delocalised) canonical orbitals into hybridorbital like ones, but it results in a low occupied carbon lone pair orbital and a partially occupied anti-bonding nitrogen-nitrogen π orbital.
(Occupancy) Bond orbital / Coefficients / Hybrids
------------------ Lewis ------------------------------------------------------
1. - 3. core orbitals (omitted)
4. (1.97583) LP ( 1) N 1 s( 67.37%)p 0.48( 32.60%)d 0.00( 0.03%)
5. (1.33940) LP ( 1) C 3 s( 0.00%)p 1.00( 99.92%)d 0.00( 0.08%)
6. (1.99655) BD ( 1) N 1- N 2
( 43.82%) 0.6620* N 1 s( 33.02%)p 2.02( 66.72%)d 0.01( 0.26%)
( 56.18%) 0.7495* N 2 s( 46.00%)p 1.17( 53.92%)d 0.00( 0.08%)
7. (1.99587) BD ( 2) N 1- N 2
( 51.93%) 0.7206* N 1 s( 0.00%)p 1.00( 99.63%)d 0.00( 0.37%)
( 48.07%) 0.6933* N 2 s( 0.00%)p 1.00( 99.80%)d 0.00( 0.20%)
8. (1.98025) BD ( 3) N 1- N 2
( 39.11%) 0.6254* N 1 s( 0.00%)p 1.00( 99.54%)d 0.00( 0.46%)
( 60.89%) 0.7803* N 2 s( 0.00%)p 1.00( 99.89%)d 0.00( 0.11%)
9. (1.99580) BD ( 1) N 2- C 3
( 65.65%) 0.8103* N 2 s( 53.76%)p 0.86( 46.18%)d 0.00( 0.05%)
( 34.35%) 0.5861* C 3 s( 30.11%)p 2.32( 69.75%)d 0.00( 0.14%)
10. (1.95419) BD ( 1) C 3- H 4
( 60.92%) 0.7805* C 3 s( 34.95%)p 1.86( 65.03%)d 0.00( 0.02%)
( 39.08%) 0.6251* H 4 s( 99.91%)p 0.00( 0.09%)
11. (1.95419) BD ( 1) C 3- H 5
( 60.92%) 0.7805* C 3 s( 34.95%)p 1.86( 65.03%)d 0.00( 0.02%)
( 39.08%) 0.6251* H 5 s( 99.91%)p 0.00( 0.09%)
---------------- non-Lewis ----------------------------------------------------
12. (0.01076) BD*( 1) N 1- N 2
( 56.18%) 0.7495* N 1 s( 33.02%)p 2.02( 66.72%)d 0.01( 0.26%)
( 43.82%) -0.6620* N 2 s( 46.00%)p 1.17( 53.92%)d 0.00( 0.08%)
13. (0.64416) BD*( 2) N 1- N 2
( 48.07%) 0.6933* N 1 s( 0.00%)p 1.00( 99.63%)d 0.00( 0.37%)
( 51.93%) -0.7206* N 2 s( 0.00%)p 1.00( 99.80%)d 0.00( 0.20%)
14. (0.07754) BD*( 3) N 1- N 2
( 60.89%) 0.7803* N 1 s( 0.00%)p 1.00( 99.54%)d 0.00( 0.46%)
( 39.11%) -0.6254* N 2 s( 0.00%)p 1.00( 99.89%)d 0.00( 0.11%)
This is usually an indication of a strongly delocalised system such as a three-centre bond. Investigating the molecule with natural resonance theory gives the rather odd contribution of long range N1 - C3 bond.
TOPO matrix for the leading resonance structure:
Atom 1 2 3 4 5
---- --- --- --- --- ---
1. N 2 2 0 0 0
2. N 2 0 2 0 0
3. C 0 2 0 1 1
4. H 0 0 1 0 0
5. H 0 0 1 0 0
Resonance
RS Weight(%) Added(Removed)
---------------------------------------------------------------------------
1* 33.02
2*(2) 32.31 N 1- N 2, ( N 2- C 3), ( N 1), C 3
3*(2) 28.81 N 1- C 3, ( N 2- C 3), ( N 1), N 2
minor contributions omitted
---------------------------------------------------------------------------
100.00 * Total * [* = reference structure]
According to NBO, the terminal nitrogen has an $\mathrm{sp}^{0.5}$ σ-type lone pair orbital, two $\mathrm{p}$ π-type bonding orbitals, and a $\mathrm{sp}^2$ σ-type bonding orbital.
Hybridisation is a mathematical tool to analyse a bonding situation. There is no correct way of applying it. It is also very important to understand an atom is never hybridised. (Even though organic chemists tend to use this jargon - even in textbooks.) The orbitals at an atom can be hybridised.
Until someone steps up and performs calculations, it is best to assume Occam’s razor for determining the hybridisation of the nitrogen atoms. The simplest solution for the outer nitrogen is to assume no hybridisation.
There are two common resonance structures for diazomethane:
$$\begin{gather}\ce{H2C=\overset{+}{N}=\overset{-}{N} <-> H2\overset{-}{C}-\overset{+}{N}#N}\\\ce{(A)\quad<->\quad(B)}\end{gather}\tag{1}$$
Resonance structure $\mathrm{(B)}$ has a single lone pair on the terminal nitrogen, so applying Occam’s razor tells us to put it into an s-type orbital. Such an orbital would also be present in resonance structure $\mathrm{(A)}$ and it would again house a lone pair.
Resonance structure $\mathrm{(B)}$ has no further lone pair but 2 orthogonal p orbitals which each form a π bond to the neighbouring nitrogen. One of these π bonds does not extend any further as it reaches the $\ce{C-H}$ bonds on carbon’s side; the other extends across to interact with the lone pair on carbon. There are three orbitals of this type; π1, π2 and π3; the first fully bonding, the second partially bonding, the third fully antibonding. The lower two are populated giving putting four electrons (one double bond and one lone pair) into that π system. This is important for resonance structure $\mathrm{(A)}$ that we’re about to come back to.
$\mathrm{(A)}$ must feature the same π system as there is no rehybridisation between resonance structures. Thus, there must also be two orthogonal π orbitals on the terminal nitrogen; one again forming a π bond with it’s neighbour (the one in plane with the hydrogens), the other this time housing a lone pair.
Instead of allowing for an unhybridised terminal nitrogen, the same analysis also permits the assumption that the terminal nitrogen is $\mathrm{sp}$ hybridised. In this case, it would be an $\mathrm{sp}$ orbital housing the lone pair present on nitrogen in both $\mathrm{(A)}$ and $\mathrm{(B)}$.
It is not a good idea to say ‘the negative charge participates in resonance’ in the way you seem to be intending it to be understood. The entire molecule is neutral, so any macroscopic partial negative charges must be balanced by a corresponding partial positive charge. Nitrogen being more electronegative than carbon allows us to assume the the partial negative charge is primarily located on the nitrogens while the partial positive charge is on carbon. However, this related question on the dipole moment of carbon monoxide shows that we should never assume partial charges or dipole moments by looking at the Lewis structures alone.
In da wikipedia you can see that the C=N=N fragment is linear AND another resonance structure involves triple N-N bond. So, the answers
Diazomethane typically reacts with its negatively charged carbon, so this is of little consequence though.
