13
$\begingroup$

My textbook (Organic Chemistry, 2nd Ed. Hornback, Joseph) states that if a hydrogen is located near the center of an aromatic ring it will experience an (often quite sizable) upfield shift, as seen in these images (borrowed from aforementioned textbook):

Examples of molecules where a shielded proton is located directly above an aromatic ring

Theoretically it all makes sense - the ring current of the aromatic system shields these protons rather strongly. But is there a way to estimate what the actual upfield shift will be? For example, if provided with structure 1 and asked to estimate the chemical shift of the red hydrogen, how could you do so? Or do you just run the NMR and find out? My book doesn't give any advice on how to figure this out, just that it happens.

Improve this question
$\endgroup$
1
  • $\begingroup$ In principle it is possible to calculate shifts using Density Functional Theory, for example using Gaussian. In practice, such calculations are highly demanding and (due to the necessity to approximate) are prone to substantial errors. $\endgroup$
    – L A
    Commented Mar 2, 2022 at 11:06

2 Answers 2

Reset to default
13
$\begingroup$

Johnson and Bovey first proposed a theory describing the ring current effect,[1] and in 1972 Haigh and Mallion published their often-cited paper on ring current shielding.[2] A later review by these authors in 1979 describes the mathematical prediction of ring currents very well.[3] You may often see this graphic describing ring current contributions to chemical shifts in textbooks.

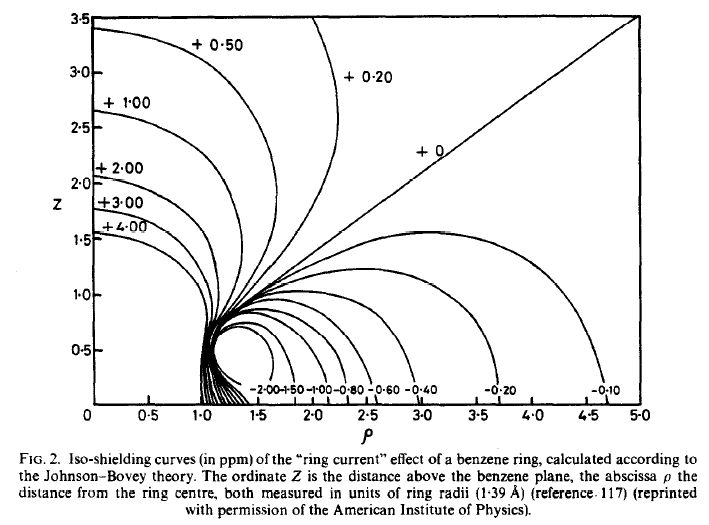
enter image description here

So, in answer to your question, it is possible in theory to make chemical shift predictions for nuclei within the shielded regions of ring currents, however in practice, it is very difficult and prone to large errors. Calculations require a very thorough knowledge of distances and angles between the aromatic plane and the nucleus of interest. And a reasonable computer.

Very few chemical shift prediction tables in texts or literature give examples of upfield-shifted aromatics, and none that I'm aware of give enough tabulated data to extend an even ballpark prediction. This makes it very, very difficult to make a prediction unless you have experience with the particular type of molecules you are dealing with. For example, I work with one research group who makes quite good estimations of chemical shifts for the inner ring proton within porphyrin systems, but this has evolved over many years of experience of dealing with this particular class of molecule. They would really be at a loss trying to predict any of the shifts for the 4 compounds you have shown.

Most desktop chemical shift predictors use an additive model based on combined chemical shift contributions, and do not do a full calculation of ring current properties. In fact, many desktop predictors give very poor estimations for these types of protons for this very reason, as they do not distinguish shielded and deshielded regions arising from ring current. For small organic molecules the variables dictating the ring current contribution are difficult to predict, especially for those signals experiencing increased shielding, such as overhanging methyl groups. They require knowledge of distance from the centre of the aromatic ring and angle from the plane. This information is often used qualitatively in spectral analysis to construct a 3-dimensional structure of a molecule, in explaining upfielded shifts of certain nuclei.

In proteins, NMR shift prediction models are more inclusive, as the molecule conformations are often very highly constrained and well predicted. Many shift predictors for protein NMR includes a ring current contribution.

References

  1. Johnson, C. E.; Bovey, F. A. Calculation of Nuclear Magnetic Resonance Spectra of Aromatic Hydrocarbons. J. Chem. Phys. 1958, 29 (5), 1012–1014. DOI: 10.1063/1.1744645.
  2. Haigh, C. W.; Mallion, R. B. New tables of ‘ring current’ shielding in proton magnetic resonance. Org. Magn. Reson. 1972, 4 (2), 203–228. DOI: 10.1002/mrc.1270040203.
  3. Haigh, C.; Mallion, R. Ring current theories in nuclear magnetic resonance. Prog. Nucl. Magn. Reson. Spectrosc. 1979, 13 (4), 303–344. DOI: 10.1016/0079-6565(79)80010-2.
Improve this answer
$\endgroup$
0
2
$\begingroup$

Magnetic anisotropy effects are very difficult to calculate. As such I don't believe there is a table that help you calculate the value so an experiment or a similar example is required. A look at Pretsch shows only one example of this system (structure 1 in your book). Running one of the structures in a NMR predictor gives results that are quite far from the experimental values and obviously don't take this effect into consideration

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.