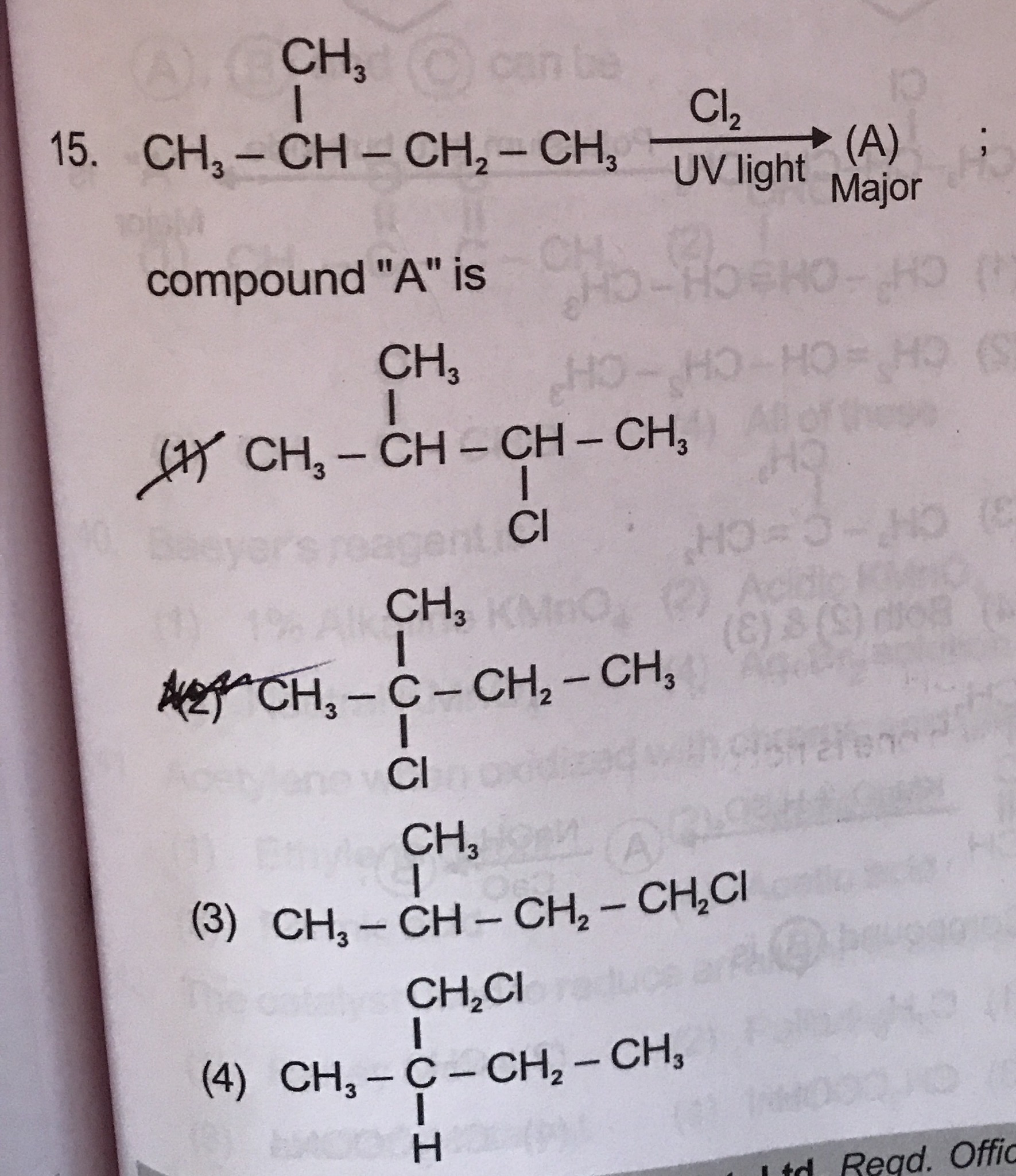
I do not understand how we obtain the product in option one. According to Free radical stability order tertiary carbon radical is more stable than secondary carbon radical which is more stable than primary carbon radical. So hydrogen selectivity should also follow the same order. Then why is it that we get option(1) instead of option (2). I’m only in high school so please try to keep it simple.
