While reading about the structure of an atom, I've encountered (even in some renowned books) the statement that electrons and nuclei are attracted due to electrostatic, or Coulombic, attractions.
However, since the charged particles (electrons and nuclei) are moving, how can the Coulombic force be sufficient to describe their interactions? Surely electrostatics cannot be used in this context. Is it merely an approximation?
If I understand the question correctly, OP is somewhat surprised that Coulomb's law is used to describe the interaction between an electron and a nucleus, although it is usually pictured that electrons are moving and Coulomb's law describes interaction between static particles. Should not then the Lorentz law be used instead Coulomb's one?
First note, that electrons do not move around a nucleus in an atom. At least, they do not do so in the classical sense. Yet, a non-zero orbital angular momentum $\vec{L}$ of an electron in an atom gives rise to the orbital magnetic dipole moment $\vec{\mu}_{L}$ and so the magnetic field is indeed generated by the "moving" electron. Plus, an electron has an intrinsic magnetic moment (spin) which also contributes to this magnetic field. The nucleus possibly possess orbital magnetic momentum as well (if has non-zero angular momentum) that couples with its spin which interacts with that of electron. These interactions splits the energy levels of the atom and the resulting hyperfine structure can be measured using radio-frequency spectroscopy.
And in principle, for a complete description of an atom we could use a model in which we treat the interaction between electron and nucleus taking magnetic interactions into account, i.e. similarly to how it is done in classical electrodynamics with the Lorentz law. There is a problem with the Lorentz law though - it is formulated in terms of forces and velocities and this terms are not even present in the vocabulary of quantum mechanics. So, the magnetic field must appear somehow differently in the Hamiltonian, and to make a long story short the full (non-relativistic) Hamiltonian for one electron in an external electromagnetic field is: $$ \hat{H} = \frac{1}{2m}(\boldsymbol{p} - e\boldsymbol{A})^2 + e\phi \, , $$ where $\phi$ is the scalar potential and $\boldsymbol{A}$ is the vector potential. And even in the absence of an external to the whole atom magnetic field, the nucleus itself gives rise to the vector potential $\boldsymbol{A}$.
So, this is certainly true that electrostatic interaction between electron and nucleus is not the whole story. However, electrostatic interaction dominates magnetic ones by far, so that usually the later are just ignored, unless a very high precision is required.
It is a misconception that one finds in the physical literature too often. The correct Coulomb energy is not static, but varies with time implicitly as
$$V_\mathrm{Coul} = V_\mathrm{Coul}(\boldsymbol{R}(t)) = \frac{ee}{4\pi\epsilon_0 |\boldsymbol{R}(t)|}$$
with $\boldsymbol{R}$ the interparticle distance. The problem is many people confounds this with the field-theoretic quantity
$$V_\mathrm{field} = \frac{1}{2} \int \mathrm{d}^3 \boldsymbol{x} \int \mathrm{d}^3 \boldsymbol{y} \frac{\rho(\boldsymbol{x})\rho(\boldsymbol{y})}{4\pi\epsilon_0 |\boldsymbol{x}-\boldsymbol{y}|}$$
which is a static quantity, besides divergent (and unphysical). This confusion is not a problem exclusive to quantum theory, one already finds this kind of confusion in classical physics. In Ref. [1] you can find a detailed mathematical proof that the Coulomb potential $\phi(\boldsymbol{R}(t))$ is not reducible to the field-theoretic potentials $\phi(\boldsymbol{r},t)$.
If all of the above was not enough, there are additional misconceptions in quantum theory regarding the time dependence of operators. One finds too often the claim that operators in the Heisenberg picture vary with time, but they do not in the Schrödinger picture. This is not true, and in more advanced textbooks one finds expressions such as
$$i\hbar \frac{d}{dt} A_H(t) = [A_H(t), H_H(t)] + i\hbar \left( \frac{d}{dt} A_S(t)\right)_H$$
where the last term (missing in some textbooks) accounts for the time change of the operator in the Schödinger picture (subindex "S" is the operator in the Schrodinger picture and subindex "H" is in the Heisenberg picture). In what follow I work in the Schrödinger picture and avoid the subindex "S".
The point is that electrons and nuclei, all together attract or repel with non-electrostatic interactions, because electrons are never at rest in atoms.
In the nonrelativistic limit, the interaction between charges is given by the Coulomb potential $V_\mathrm{Coul}$. In the phase space formulation of quantum mechanics one simply uses this expression directly. In the Schrödinger wavefunction formulation one has first to replace position variables with Schrödinguer operators $\boldsymbol{x}\rightarrow \hat{\boldsymbol{x}}$; those operators maintain the implicit time dependence of the classical variables $\boldsymbol{x}=\boldsymbol{x}(t)$, and one obtains a Coulomb operator with a implicit time dependence $\hat{V}_\mathrm{Coul}(\hat{\boldsymbol{R}}(t))$.
This implicit time-dependence is not quoted in the literature, but it is needed to make sense of dynamical variables. For instance if we want to compute the velocity of an electron in the Schrödinger picture, we have first to obtain the velocity operator as
$$\hat{\boldsymbol{v}} = \frac{d\hat{\boldsymbol{x}}}{dt} = \frac{i}{\hbar} [\hat{H},\> \hat{\boldsymbol{x}}]$$
and then use this velocity operator in the ordinary Schrödinger-picture expression
$$\langle\boldsymbol{v}\rangle = \int d^3\boldsymbol{x} \>\Psi^{*}(t)\>\hat{\boldsymbol{v}} \>\Psi(t)$$
REFERENCE
[1] https://journals.aps.org/pre/abstract/10.1103/PhysRevE.53.5373
While there are quite a few answers here already, I would like to approach this without going into complicated theories.
You might have heard that Coulomb's law can't be used in the case where charges move. You were thinking that the Coulomb's law is incorrect for moving charges, but the fact is that Coulomb's law is inadequate to completely describe the forces on moving charges.
Coulomb's law deals with electrostatic attraction. These attractions depend only on the magnitude of charges and the separation between them. However, while charges are moving, different types of interactions come into the picture. Those are called magnetic forces. Like electrostatic forces, their magnitude depend on the magnitude of the charges and the separation between them, but in addition, they also depend on the velocity of the charges, in both magnitude and direction.
Before we start to analyze the magnetic forces, we need to know what a magnetic field is. Magnetic fields, like electric fields, exist and are produced by charges. However these fields are produced by, and can interact with only moving charges. Let's see how the fields are produced a bit later, now I'll show you how a moving charge behaves under a magnetic field.
If we represent the magnetic field by a vector $\vec{B}$, and a charge $q$ moving with a velocity $\vec{v}$, the force the charge experiences is:
$$\vec{F} = q(\vec{v}×\vec{B})$$
The force acts perpendicular to both the field and the velocity.
Moving charges can also produce magnetic fields. The field produced is given by the Biot-Savart law.
$$\vec{B} = \frac{\mu_0}{4\pi}\frac{q(\vec{v}×\vec{r})}{r^3}$$
where $\vec{r}$ is the position vector of the point where you are measuring the magnetic field, with respect to the moving charge as origin.
The combination of both the electric and magnetic fields together are needed in the case of moving charges.
Let's see. In the Bohr model, you have a stationary nucleus, and an orbiting electron that follows a circular path. The electron moving in the circular path will be generating a magnetic field, that looks like this:
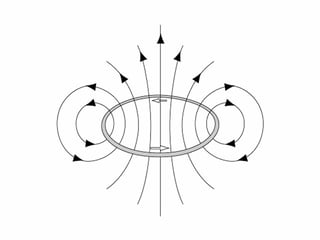
If you tried to use Biot-Savart law, you should be able to arrive at the conclusion that a spinning electron produces a magnetic field at the nucleus of with a magnitude of,
$$B_{\ce{e-}}=\frac{\mu_0ev}{4\pi a_0^2}$$
or
$$B_{\ce{e-}}=\frac{\mu_0he}{8\pi^2 m_{\ce{e-}}a_0^3}$$
What we have derived above is perfectly valid, and has also been verified. Orbiting electrons do produce magnetic fields, and their magnetic moments have been determined in laboratories. The magnetic moment of a hydrogen atom, is called the Bohr magneton, is a very popular unit to measure magnetic moments in transition metal compounds and coordination compounds.
However, the nucleus is still at rest. A magnetic field cannot interact with a particle at rest, as we have seen earlier. So the magnetic fields do not affect the nucleus, and the simple electrostatic model is enough to predict the properties of the hydrogen atom.
As many others have pointed out, the Bohr model is rather outdated, and that we use quantum mechanics to explain the atom. I'm not very familiar with the quantum mechanical model, so I can't tell you much about that. Nevertheless, do not dwell on the Bohr model too much, it can say some stuff, but not everything.
Hopefully I clarified you completely. Leave a comment if you need more clarification.
Electrons and neutrons are not attracted from strong electrostatic interaction.
Electrons and nuclei are because nuclei contain protons. Coulombic physics are therefore entirely relevant for atoms, because protons bear a 1$e$ charge, while electrons bear a -1$e$ charge.
(Don't confuse neutrons with nuclei!)
Neutrons interact with matter in two main ways: first, within the nucleus, through the strong force, reported to be ~137 times stronger than electromagnetic force. This is what binds neutrons to protons in the first place, in the tiny nucleus. The second is through interactions of magnetic fields. This second way is the only way that neutrons interact with matter outside of the nucleus, and it is what makes them useful for things like neutron-powder diffraction (an alternative to X-ray crystallography).
