(Note that this question has been fully edited for clarity, SE help advised not to delete the question and post another).
Background:
I have a computer model in which I feed it input data from various feedstocks usually containing sodium. My calculations input NaOH data (because it's an industry standard and easy to use for the user) but calculate Na2O for output quantities for the sake of consistency as everything else is in oxide form (e.g. SiO2, Al2O3, H2O etc.). Note that the full model incorporates the following metrics from each inputted feedstocks:
- Feedstock Elemental Mol Quantities: (e.g. mols Si, mols Al and mols Na (etc.) from every feedstock, note that mols H instead of mols H2O is used for consistency in using elemental mols instead of changing to an oxide just for water).
- Feedstock Oxide Weight Quantities: (e.g. wt% SiO2, wt% Al2O3, wt% Na2O (etc.) from every feedstock).
- Feedstocks Solid wt%s: (i.e. $Solids_{i,wt\%s} = Feedstock_{i,Total,wt\%} - H_2O_{i,wt\%}$; the summed wt%s of all non-H20wt% quantities in a given feedstock)
- Feedstock Liquids wt%: (i.e. $Liquids_{i,wt\%s} = Feedstock_{i,Total,wt\%} - Solids_{i,wt\%s} = H_2O_{i,wt\%}$
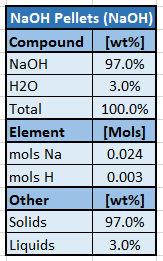
One such feedstock which is commonly used in the model are the standard reagent grade 97 wt% NaOH pellets. Knowing the reaction between NaOH and Na2O (i.e. 2NaOH -> Na2O + H2O), if I wanted to calculate the composition of a 1 g NaOH pellets I get the following (note that I did try to put everything in Chem SE table format but it was too difficult to do, format and the help page didn't provide too much clarity from my POV):
1. First, the pellet's composition with respect to NaOHwt%:
Calculations are as follows:
NaOHwt% = 97 wt% NaOH as per the specification sheet mentioned in the link above.
Note that it has been assumed that $H_2O_{wt} = 100_{wt\%} - Solids_{wt\%} = 100_{wt\%} - NaOH_{wt\%} = 3_{wt\%} H_2O$
$mols Na = \frac{97 wt\% NaOH}{100 wt\% Pellets} \times \frac{100wt\% pellets}{1 g pellets} \times \frac{1 g NaOH}{100 wt\% NaOH} \times \frac{1 mol NaOH}{40 g NaOH} \times \frac{1 mol Na}{1 mol NaOH} = 0.0243 \frac{mols Na}{1 g pellets}$
$mols H = \frac{3 wt\% H2O}{100 wt\% Pellets} \times \frac{100wt\% pellets}{1 g pellets} \times \frac{1 g H_2O}{100 wt\% H_2O} \times \frac{1 mol H_2O}{18.02 g H_2O} \times \frac{2 mols H}{1 mol H_2O} = 0.0033 \frac{mols H}{g pellets}$
$Solids_{i,wt\%s} = 100_{wt\%} - 3_{wt\%,H_2O,i} = 97 \%$
$Liquids_{i,wt\%s} = 3_{wt\%,H_2O,i} = 3 \%$
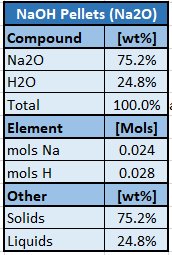
2. Secondly, the pellet's composition with respect to Na2Owt%:
Calculations are as follows:
Note that Na2Owt = $ \frac{97 wt\% NaOH}{100 wt\% Pellets} \times \frac{100wt\% pellets}{1 g pellets} \times \frac{1 g NaOH}{100 wt\% NaOH} \times \frac{61.98 g Na2O / mol Na2O}{40 g NaOH /mol NaOH} \times \frac{1mol Na/ mols NaOH}{2mols Na/mols Na2O} = \frac{0.752gNa2O}{1 g Pellets} = 0.752 \frac{g Na_2O}{1 g pellets} \times 100\% = 75.2 wt\% Na_2O$
The resultant H2Owt% as per the above 3wt%, I presume instead needs to be of $H_2O_{wt} = 100_{wt\%} - Na_2O_{wt\%} = 24.8_{wt\%} H_2O$ which seems ludicrous...
$mols Na = \frac{75.2 wt\% Na_2O}{100 wt\% Pellets} \times \frac{100wt\% pellets}{1 g pellets} \times \frac{1 g Na_2O}{100 wt\% Na_2O} \times \frac{1 mol Na_2O}{62 g Na_2O} \times \frac{2 mols Na}{1 mol Na_2O} = 0.0243 \frac{mols Na}{1 g pellets}$
$mols H = \frac{24.8 wt\% H2O}{100 wt\% Pellets} \times \frac{100wt\% pellets}{1 g pellets} \times \frac{1 g H_2O}{100 wt\% H_2O} \times \frac{1 mol H_2O}{18.02 g H_2O} \times \frac{2 mols H}{1 mol H_2O} = 0.028 \frac{mols H}{g pellets}$
$Solids_{i,wt\%s} = 100_{wt\%} - 24.8_{wt\%,H_2O,i} = 75.2 \%$
$Liquids_{i,wt\%s} = 24.8_{wt\%,H_2O,i} = 24.8 \%$
In can be seen that, although the mols Na are the same, neither the mols H nor the solids or liquids parameter match up. Which doesn't seem to make sense to me?
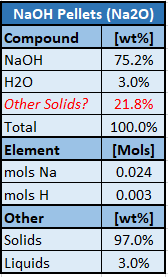
3. Finally, the pellet's nonsensical composition with respect to Na2O:
- The only solution I've managed to balance with the initial NaOH composition with respect to the Na2Owt doesn't make sense as I've had to add a nonsensical "Other solids?" compound which accounts for the missing solids to ensure that the mols of Na, H and solids and liquids quantities remain consistent.
My questions from the above are as following:
What is the missing component (maybe the "other solids" quantity) which resolves the inconsistency between the NaOH pellet's composition with respect to the NaOHwt and Na2Owt?
Is the H2O wt% assumption a good one to make due to the pellet's hygroscopic property?
Is it better to only assume the 2 NaOH <> Na2O + H2O reaction for solids and therefore only use it in aqueous solutions with NaOH or Na2O?
Should NaOH pellets only be quantified by their NaOH composition and not Na2O for better representation and accuracy?