1
$\begingroup$
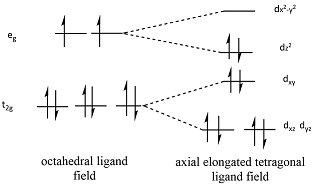
  1. Chemistry LibreTexts stated clearly that (under the section 'Large $\Delta$' and 'Small $\Delta$') octahedral d$^8$ system does not exhibit Jahn-Teller distortions. But then it stated also:

...Some common examples (that exhibit distortions) include Cr$^{3+}$, Co$^{3+}$, and Ni$^{2+}$

Isn't Ni$^{2+}$ d$^8$(Group 10, +2, so 10 - 2 = 8) and should not exhibit distortions?

  1. I believe this question is asking the similar thing. But then when the energy level diagrams drawn out as shown below, I saw that even for d$^8$, if singlet favoured over triplet, the distortion does lower the energy (and so should happen).

enter image description here

So what is wrong with the two arguments above?

Improve this question
$\endgroup$
6
  • 4
    $\begingroup$ Your distortion doesn’t preserve spin. As drawn, you should have one electron in z2 and one in x2-y2. Something is wrong with the sentence that you quoted. Perhaps they meant Ni(III), which does exhibit JT distortions. I also don’t know where they got Co(III) from. Co(III) is nearly always low spin and hence no JT distortion. Likewise, Cr(III) is d3, which should not have JT distortions. $\endgroup$
    – orthocresol
    Commented Dec 10, 2020 at 11:44
  • 2
    $\begingroup$ Having looked at the paragraph in more detail, I think it is just poorly written and these are supposed to be examples of ions which don’t exhibit JT distortions, rather than ions which do. They literally picked out d3, d6, and d8 examples. $\endgroup$
    – orthocresol
    Commented Dec 10, 2020 at 11:48
  • $\begingroup$ @orthocresol I just remember the spin must be preserved! But based on that, does that mean that, all d8 axial elongated tetragonal complexes will be paramagnetic (as total spin cannot be 0)? $\endgroup$
    – TheLearner
    Commented Dec 10, 2020 at 12:03
  • 2
    $\begingroup$ You can put the electron in the lower orbital, thus getting a singlet state, basically what you've drawn. The only question is whether it's more stable than the alternative, which is to have a triplet state with one electron in $z^2$ and $x^2-y^2$. That would depend on the classical considerations of pairing energy and orbital energy gap: for example, in square planar complexes, the $z^2$ and $x^2-y^2$ are so far apart that both electrons sit inside the $z^2$. Thus, for example, $\ce{[Ni(CN)4]^2-}$ is diamagnetic. But that is no longer the original topic of Jahn–Teller distortions. :-) $\endgroup$
    – orthocresol
    Commented Dec 10, 2020 at 12:13
  • $\begingroup$ I know I shouldn't say thank you here, but I still want to thank you to @orthocresol for often answering my question so pertinently. $\endgroup$
    – TheLearner
    Commented Dec 10, 2020 at 23:33

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.