Why does carbon monoxide have a greater affinity for hemoglobin than oxygen?

Question

Hemoglobin is an iron-containing oxygen transport metalloprotein in the red blood cells of most mammals. Simply put, it's a carrier protein. Interestingly it doesn't carry carbon dioxide in the same way it does for oxygen. Oxygen binds to the iron atoms in the protein whereas carbon dioxide is bound to the protein chains of the structure. Carbon dioxide doesn't compete with oxygen in this binding process.

However, carbon monoxide is a very aggressive molecule. It's a colourless, odourless, and tasteless gas that is lighter than air and can be fatal to life. It has a greater affinity for hemoglobin than oxygen does. It displaces oxygen and quickly binds, so very little oxygen is transported through the body cells.

There are two equilibrium reactions of binding oxygen and becoming oxygenated hemoglobin:

$$\ce{Hb (aq) + 4O2 (g) -> Hb(O2)4 (aq)}$$

$$\ce{Hb (aq) + 4O2 (g) <- Hb(O2)4 (aq)}$$

And carbon monoxide binding equation at equilibrium:

$$\ce{Hb (aq) + 4CO (g) ⇌ Hb(CO)4 (aq)}$$

It is said the equation is shifted towards right, generating $\ce{Hb(CO)4(aq)}$, since its bond is much stronger.

I have two main related questions:

1. Why is the carboxyhemoglobin bond stronger relative to that of oxygenated hemoglobin?

2. Why is carbon monoxide highly attracted to hemoglobin?

Does it have anything to do with the oxidation state of oxygen in each molecule? Please show some reaction equations associating $\ce{Fe}$.

Answer 1

Excursion into simple coordination chemistry: Bonding, backbonding and simple orbital schemes

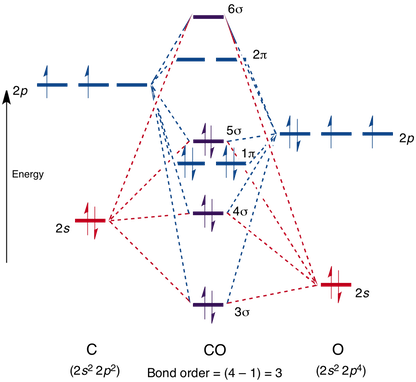
Please refer to Breaking Bioinformatic’s answer for the MO scheme of carbon monoxide, it is very helpful. You might also look at the orbital pictures in this answer by Martin. Carbon monoxide can bind to metal centres via a σ coordinative bond where the HOMO of $\ce{CO}$ interacts with metal orbitals and also by the π backbonding, Breaking Bioinformatics mentioned. I’ll start by touching the σ bond so we can later better understand the π bond. In figure 1 you can see the molecular orbital scheme of a complex composed of a central metal ion and six ligands that donate in a σ manner exclusively.

Figure 1: Molecular orbital scheme of an octahedral complex with six σ donors around a central metal. Copied from this site and first used in this answer of mine. Metal orbitals are 3d, 4s, 4p from bottom to top; ligand orbitals are of s-type.

You will notice that figure 1 contains the irreducible representations of the orbitals beneath them. Orbitals can only interact if they have identical irreducible representations; otherwise, their interactions will sum up to zero. We can see that the metal d orbitals are split up into a $\mathrm{t_{2g}}$ set and and $\mathrm{e_g}^*$ set. This is the effect of σ bonding and why it stabilises the entire entity.

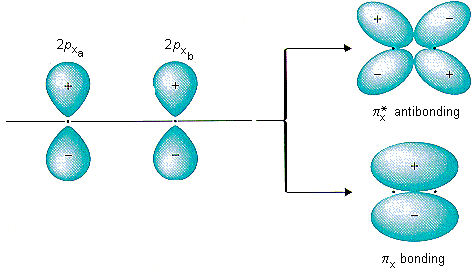
π bonding can only occur if the ligands have available orbitals of π symmetry. The 2π orbitals of $\ce{CO}$ are a nice example, but one could also simply assume a halide with its p-orbitals for the same effect. Further down in the internet scriptum I originally copied the picture from, you can see a set of two pictures that introduce p-orbitals. Twelve ligand p-type orbitals will transform as $\mathrm{t_{1g} + t_{1u} + t_{2g} + t_{2u}}$, thus a further stabilising/destabilising interaction is introduced for the $\mathrm{t_{2g}}$ orbitals. Due to the $2\unicode[Times]{x3c0}^*(\ce{CO})$ orbitals being similar in energy to the metal’s $\mathrm{t_{2g}}$ and empty, they can stabilise the former well, creating a possibly strong stabilisation for the overall system. Since this is an interaction of two orbitals creating both bonding and antibonding orbitals, and since the resulting molecular orbital is filled with two electrons that stem from the metal centre this is termed π-backbonding.

The binding mode of carbon monoxide to haemoglobin

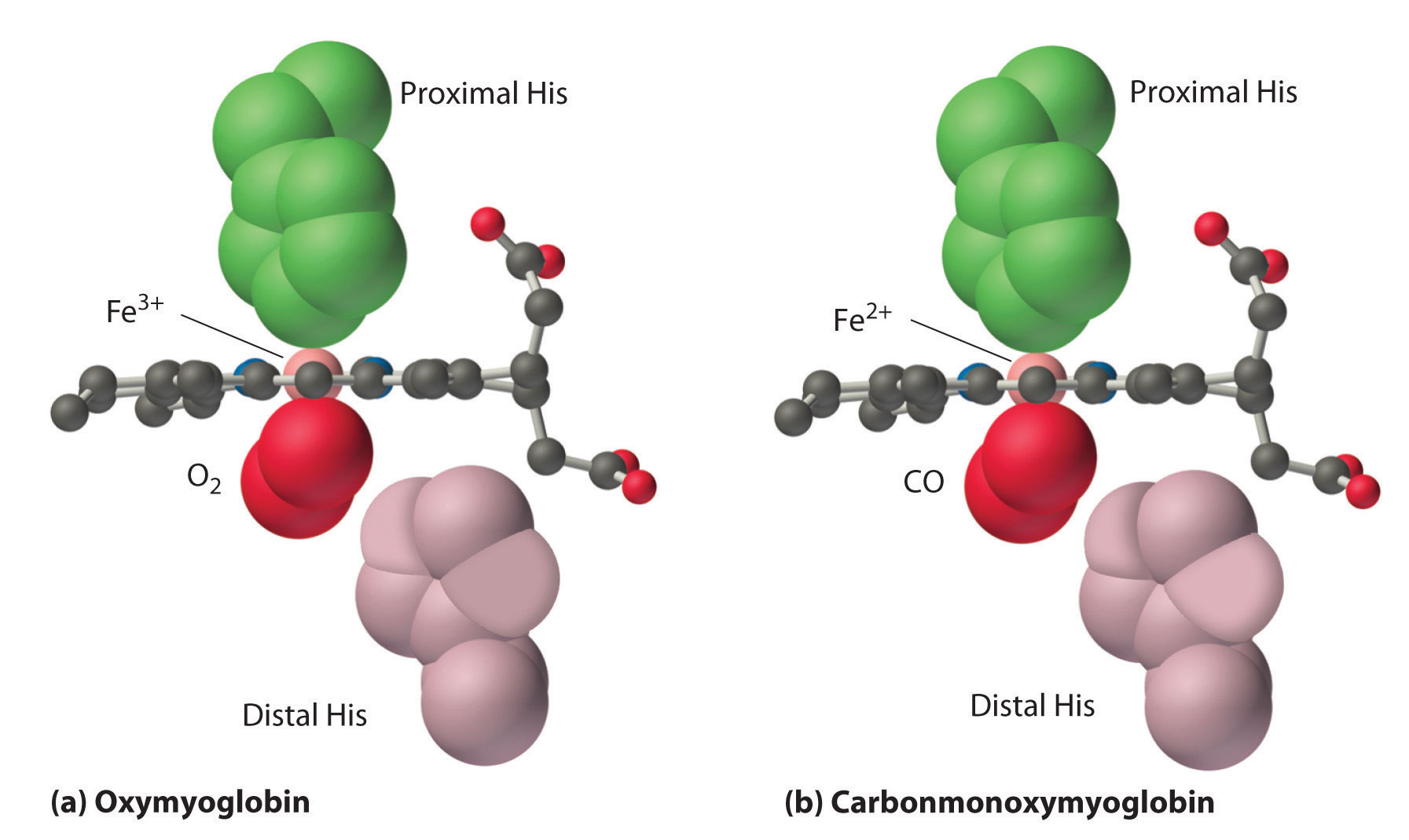
After this extensive background discussion, it may be clear that $\ce{CO}$ is generally a good ligand. For reasons I didn’t go into, a carbonyl complex of metal ions isn’t that stable, but a single carbonyl ligand will almost always be beneficial. Such is the case for the porphyrin-iron(II) system that forms the heart of haemoglobin: The central iron(II) ion is coordinated well from five directions (four from the porphyrin ring and one histidine of haemoglobin) and has a weakly bound water atom in the ground state in its sixth coordination slot, sometimes displaced by a distal histidine. Carbon monoxide can diffuse in and bind very well to this system, displacing the weakly bound water and histidine. In fact, isolated haem can bind carbon monoxide $10^5$ times better that it can oxygen.

This is something that nature could not really inhibit, because it is based on fundamental properties, but also something nature didn’t really care for, because the natural concentration of carbon monoxide is very low, and nature rarely had to deal with it competitively inhibiting oxygen binding to haemoglobin.

The binding mode of oxygen to haemoglobin

Oxygen, the $\ce{O2}$ molecule, is an absolutely lowsy σ donor — especially when compared to $\ce{CO}$. Its molecular orbital scheme is generally that of carbon monoxide except that it is entirely symmetric and two more electrons are included: They populate the 2π orbitals to give a triplet ground state. These orbitals are now the HOMOs and they hardly extend into space in any meaningful way — plus the still existant lone pair on each oxygen atoms is now at a much lower energy and also does not extend into space and thus cannot bind to a metal centre in a σ manner.

What happens here is rather complex, and the last lecture I heard on the topic basically said that final conclusive evidence has not yet been provided. Orthocresol discusses the different viewpoints in detail in this question. The diamagnetic properties of the resulting complex are, however, unquestioned and thus one must assume a singlet ground state or one where antiferromagnetic coupling cancels any spins at molecular levels. Since the ground state of haemoglobin has a high-spin iron(II) centre and the ground state of oxygen is a paramagnetic triplet, it makes sense to assume those two to be the initial competitors.

Professor Klüfers now states the steps to be the following:

1. Iron(II) is in a high-spin state with four unpaired electrons;

2. Paramagnetic triplet oxygen approaches and one of its $\unicode[Times]{x3c0}^*$ orbitals coordinates to the iron(II) centre in a σ fashion.

3. This induces a high-spin low-spin transition on iron and slightly reorganises the ligand sphere (pulling oxygen closer to the iron centre). We now have a low-spin singlet iron(II) centre and a coordinative σ half-bond from oxygen. We can attribute that electron to the iron centre.

4. Through linear combination, we can adjust the spin-carrying σ orbital and the populated iron orbitals so that an orbital is generated which can interact with the other $\unicode[Times]{x3c0}^*$ of oxygen in a π fashion.

5. We thus expect an antiferromagnetic coupling and an overall state that can be described best as $\ce{Fe^{III}-^2O2^{.-}}$ — a formal one-electron oxidation of iron to iron(III), reducing oxygen to superoxide ($\ce{O2-}$).

6. If the spin-carrying orbitals are all treated as being metal-centred, we gain a $\ce{Fe^{II}-^1O2}$ state.

(Content in the link I quoted from are in German; translation mine and shortened from the original.)

Only due to the complexly tuned ligand sphere and also due to the stabilising high-spin low-spin transition (plus reorganising) is oxygen able to bind to iron at all. The distal histidine further stabilises the complex by a hydrogen bond, alleviating the charge slightly. It is to be assumed that nature did a great deal of tuning throughout the evolution since the entire process is rather complex and well-adjusted for collecting oxygen where it is plentiful (in the lungs) and liberating it in tissue where it is scarce.

Comparison of the oxygen and carbon monoxide binding modes

The simpler picture I drew above for carbon monoxide is not correct. Inside haemoglobin, carbon monoxide also binds in an angular fashion as if it were oxygen — see bonCodigo’s answer for space-filling atom models. This is because the entire binding pocket is made to allow oxygen to bind (as I stated) thus attempting everything to make oxygen a comfortable home. Carbon monoxide is rather strained in there, its binding affinity is reduced by a factor of $1000$. However, since we started with a binding affinity difference of $10^5$, carbon monoxide can still bind $100$ times better than oxygen can.

Summary

• Carbon monoxide is generally a good ligand that can bind to metal centres well.

• Oxygen is generally a poor ligand.

• Nature did everything to make oxygen a comfortable home in haemoglobin.

• In doing so, the binding pocket became substantially less comfortable for $\ce{CO}$.

• But since carbon monoxide was so good and oxygen so poor to start with, the former still binds better than the latter.

• Nature didn’t really care, because individuals seldomly come in contact with carbon monoxide so the collateral damage was taken into account.

Answer 2

The answer has to do with pi-backbonding.

In essence, the CO molecule has a negative formal charge on the carbon (it's neutral because of the oxygen having a positive formal charge). However, C is quite electropositive, and would like to relieve the stress caused by the negative formal charge. To relieve the stress caused by the negative charge, the CO molecule will want to bond to the iron.

How it does this is related to CO's molecular orbitals, which look like:

Note that the 4, 5, and 6 sigma orbitals, as well as the 2 pi orbital, are all antibonding. I will denote them with * from now on.

As CO stands right now, it has a bond order of three (the two lower sigmas cancel each other out, since 4* is antibonding while 3 is bonding). None of its orbitals, as they stand, can participate in bonding.

However, because carbon wants to rid itself of the negative charge, CO will do an interesting trick. It will promote an electron from the 5 sigma orbital into 2* pi. This reduces the bond order of CO from three to two, and introduces a separation of an electron pair. The picture of the 2* pi orbital is shown here, on the top:

So how is this more stable? In isolation, it is not. However, remember, we are not operating in isolation: there's an iron nearby. Most saliently, the iron has a d orbital, which looks like:

Now, the iron can put an electron into this d orbital and bond it with the pi* star orbital on the CO on the carbon end. This is hugely stable (note that we were willing to promote an electron into an unoccupied orbital and break up an electron pair to do it), especially since it relieves charge stress to do it (as opposed to molecular oxygen, which does no such thing). This backbonding is also why cyanide will stable itself onto iron.

Why isn't hemoglobin designed to bind oxygen as strongly as possible, then? Surely it would seem this would be an advantage, evolutionarily, if oxygen could bind tightly and never let go? But oxygen needs to let go: hemoglobin is a transport protein, and it needs to release oxygen into the tissues. That's why you have plenty of things that bond hemes more tightly than molecular oxygen: we need $\ce{O_2}$ in our tissues, not locked into hemoglobin forever.

EDIT: The hybridization of the iron in the protein is often (but incorrectly) assigned as $\ce{sp^3d^2}$. This is implied by the octahedral geometry the ligands around the iron take: the heme consists of an iron bound in four coordination sites by a porphyrin ring, one site by a histidine, and the last site by the oxygen the iron needs to bind.

The formula:

$\ce{Fe(II) + CO -> Fe(III)CO}$

Answer 3

I appreciate above answer by BreakingBioinformatics. However I have been looking for the oxidation reactions involving Fe in this case. Found some useful material here. It is based on the textbook, Chemistry: Principles, Patterns, and Applications, 2007, Bruce Averill, Patricia Eldredge.

Note : Following picture only shows the binding of Myoglobin.

Here's the text which explains some relevant and interesting details about the affinity of O2 and CO, although the main focus of the text is on primary function of the distal histidine in preventing self-poisoning of CO.

Oxygen is not unique in its ability to bind to a ferrous heme complex; small molecules such as CO and NO bind to deoxymyoglobin even more tightly than does O2. The interaction of the heme iron with oxygen and other diatomic molecules involves the transfer of electron density from the filled t2g orbitals of the low-spin d6 Fe2+ ion to the empty π* orbitals of the ligand. In the case of the Fe2+–O2 interaction, the transfer of electron density is so great that the Fe–O2 unit can be described as containing low-spin Fe3+ (d5) and O2−. We can therefore represent the binding of O2 to deoxyhemoglobin and its release as a reversible redox reaction:

$\ce{Fe^{2+} + O2 ⇌ Fe^{3+} – O^{2−}}$ (26.8.2)

As shown in Figure 26.8.4 (the figure is not loading), the Fe–O2 unit is bent, with an Fe–O–O angle of about 130°. Because the π* orbitals in CO are empty and those in NO are singly occupied, these ligands interact more strongly with Fe2+ than does O2, in which the π* orbitals of the neutral ligand are doubly occupied.

Although CO has a much greater affinity for a ferrous heme than does O2 (by a factor of about 25,000), the affinity of CO for deoxyhemoglobin is only about 200 times greater than that of O2, which suggests that something in the protein is decreasing its affinity for CO by a factor of about 100. Both CO and NO bind to ferrous hemes in a linear fashion, with an Fe–C(N)–O angle of about 180°, and the difference in the preferred geometry of O2 and CO provides a plausible explanation for the difference in affinities. As shown in Figure 26.8.4 "Binding of O", the imidazole group of the distal histidine is located precisely where the oxygen atom of bound CO would be if the Fe–C–O unit were linear. Consequently, CO cannot bind to the heme in a linear fashion; instead, it is forced to bind in a bent mode that is similar to the preferred structure for the Fe–O2 unit. This results in a significant decrease in the affinity of the heme for CO, while leaving the O2 affinity unchanged, which is important because carbon monoxide is produced continuously in the body by degradation of the porphyrin ligand (even in nonsmokers). Under normal conditions, CO occupies approximately 1% of the heme sites in hemoglobin and myoglobin. If the affinity of hemoglobin and myoglobin for CO were 100 times greater (due to the absence of the distal histidine), essentially 100% of the heme sites would be occupied by CO, and no oxygen could be transported to the tissues. Severe carbon-monoxide poisoning, which is frequently fatal, has exactly the same effect. Thus the primary function of the distal histidine appears to be to decrease the CO affinity of hemoglobin and myoglobin to avoid self-poisoning by CO.

Answer 4

The existing answers explain, chemically, why hemoglobin ($\ce{Hb}$) binds $\ce{CO}$ much more strongly than it does $\ce{O2}$. In reading them, you might get the idea that nature evolved $\ce{Hb}$ to bind $\ce{O2}$ as well as possible, and simply didn't account for $\ce{CO}$. The latter is likely true, but not the former.

In fact, nature didn't evolve $\ce{Hb}$ to bind $\ce{O2}$ as strongly as possible. Think about how $\ce{Hb}$ operates: It doesn't merely need to bind $\ce{O2}$; it also needs to be able to release it. Yes, there is a pH/ $\ce{CO2}$/ $\ce{2,3 BPG}$-induced conformational change in $\ce{Hb}$ that facilitates this, but there are limits to how much the binding affinity can change as a result. Thus $\ce{Hb}$ can't bind $\ce{O2}$ too strongly and still fulfill its biological function. Instead, the affinity of $\ce{Hb}$ for $\ce{O2}$ must be finely balanced between binding and release.

Consequently, it is not surprising that a foreign substance ($\ce{CO}$), not present in appreciable quantitites when $\ce{Hb}$ evolved, can outcompete $\ce{O2}$. The best analogy to $\ce{Hb}$'s affinity for $\ce{O2}$ is Post-It Notes. These use an adhesive that sticks, but sticks weakly, so it can release easily when needed.