Will the bond order of CO be greater in its existence as carbon monoxide or in metal carbonyl?

Question

$$\text{Bond order} = \frac{N(b) - N(a)}{2}$$

Where $N(b)$ is the number of electrons in bonding orbitals and $N(a)$ is the number of electrons in antibonding orbitals.

As in metal carbonyls, there is synergic effect during formation of the π back bond from metal to $\ce{CO}$, so $\ce{CO}$ accepts electrons in its π* anti bonding orbital. As $N(a)$ increases, Bond order decreases. So, $\ce{CO}$ in its free state has greater bond order in comparison and hence is more stable.
Would the above concept be correct?

Answer 1

In figure 1 you can see the molecular orbital scheme of a $\ce{[ML6]}$ complex including σ and π interactions. The class of ligands is not important; however, care should be taken when correctly assigning orbitals.

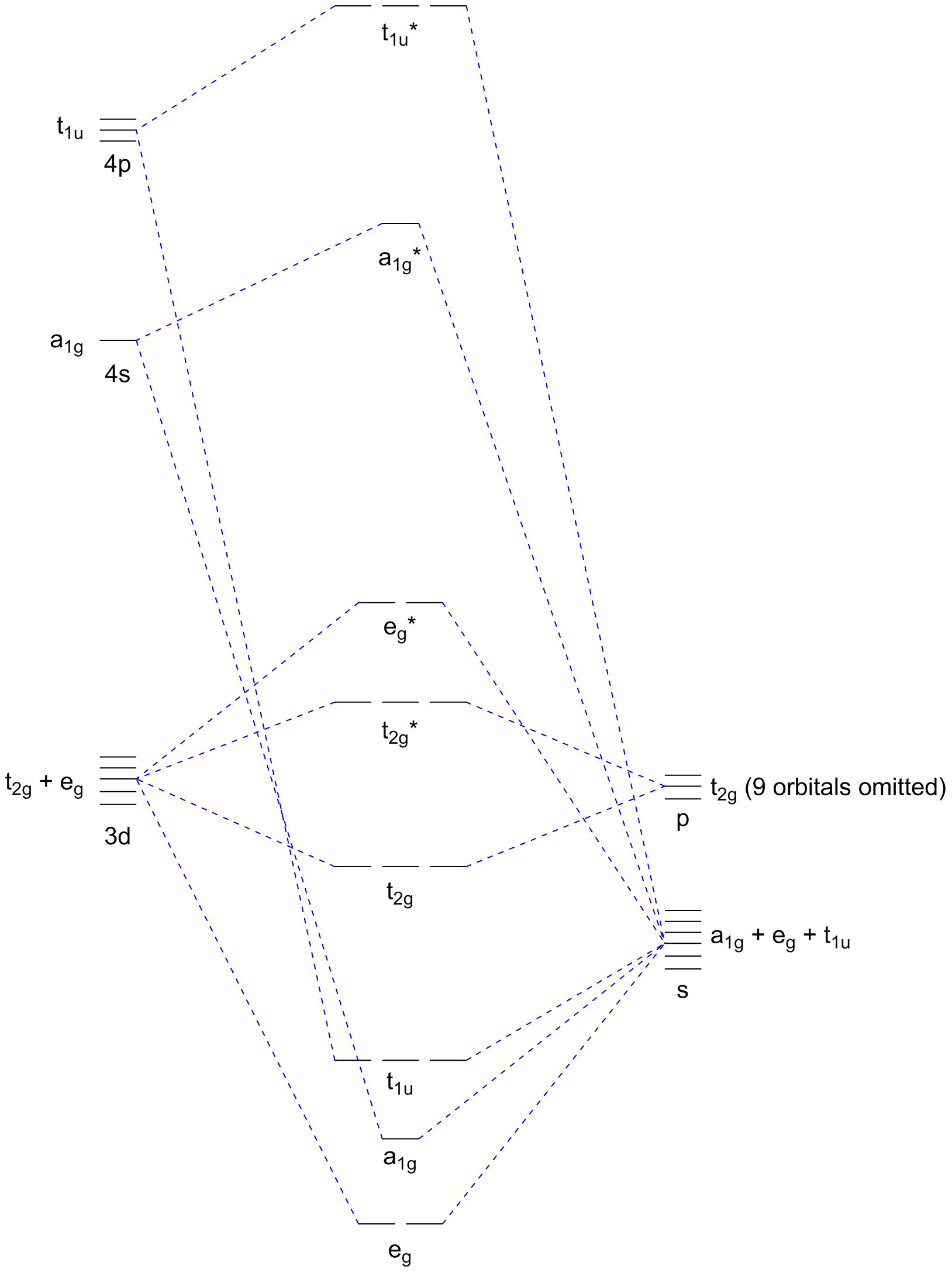
Figure 1: Octahedral $\ce{[ML6]}$ complex including σ and π ligand-metal interactions.

The first thing we should be doing is assigning the original, pre-bonding orbitals. The metal orbitals on the left are obvious. The ligand group orbitals of lower energy, $\mathrm{a_{1g} + e_g + t_{1u}}$ are the six ligand orbitals that interact with the metal in a σ fashion; in carbon monoxide, these represent the lone pair on carbon (the HOMO). The twelve p type orbitals, of which nine are omitted and the remaining transform as $\mathrm{t_{2g}}$ are π symmetric with respect to the $\ce{L\bond{->}M}$ bond and represent the $\pi^*_{\ce{C=O}}$ bonds of carbon monoxide; the LUMOs.

In a metal carbonyl complex, the left-hand side is usually higher in energy due to the metal’s lower oxidation state. Thus, the lower an orbital in the centre of the scheme is, the more ligand-centred it is.

While filling in the final complex’ electrons, we start from the bottom; first the twelve ligand electrons that represent the carbon monoxide lone pairs. Once we are done with this, the next orbitals happen to be $\mathrm{t_{2g}}$ — the bonding ones which have more ligand contribution. These can not only be seen as bonding with respect to the $\ce{M\bond{<-}L}$ bond but also antibonding with respect to the $\ce{C=O}$ bond. The maximum we can fill in here is 6 electrons. These six electrons reduce the $\ce{C#O}$ bond order as can be seen in the formula:

$$\text{B.O.} = \frac{(12 + 2\times12) - 6}{2\times6} = \frac{30}{12} = 2.5$$

The additional factor 6 in the denominator is because I am analysing six $\ce{C#O}$ bonds at the same time.

The result ($2.5$) clearly shows that the bond order is reduced with respect to noncoordinating $\ce{CO}$.

The discussion I did with an octahedral complex can be done with any coordination geometry; octahedra are just a very typical case.