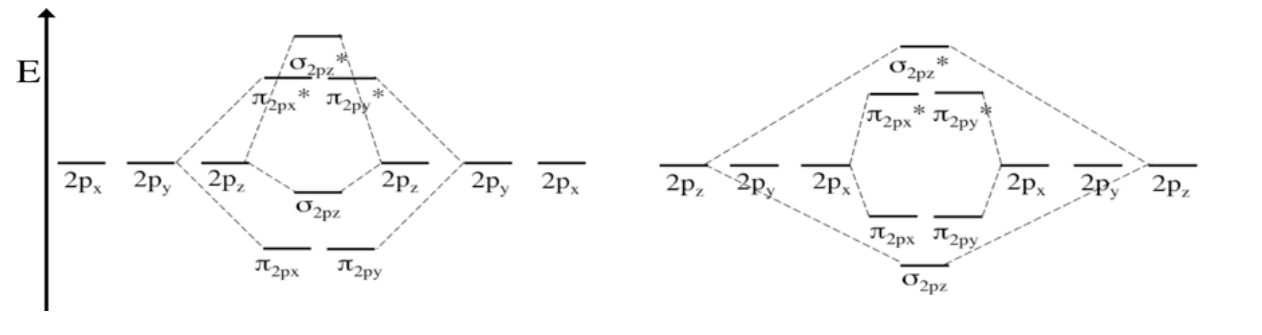
Is there a specific reason why the energy of the $\ce{\sigma_{2pz}}$ orbital varies relative to the $\ce{\pi_{2px}}$ and $\ce{\pi_{2py}}$ orbitals, depending on the atomic number of the atom?
4
-
1$\begingroup$ You should look at diagrams that are not utterly misleading. The should be s-orbitals, too. $\endgroup$– Martin - マーチン ♦Commented Nov 12, 2022 at 13:16
-
$\begingroup$ chem.libretexts.org/Bookshelves/General_Chemistry/… $\endgroup$– Martin - マーチン ♦Commented Nov 12, 2022 at 13:21
-
1$\begingroup$ @Martin-マーチン - unfortunately, the orbital diagram in that link for $\ce{Be2}$ is incorrect. See, for example ch301.cm.utexas.edu/section2.php?target=imfs/mo/… $\endgroup$– Geoff HutchisonCommented Nov 13, 2022 at 0:11
-
1$\begingroup$ See also chemistry.stackexchange.com/a/34834/5017 $\endgroup$– Geoff HutchisonCommented Nov 13, 2022 at 0:12
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
5
Orbital mixing is the general answer. Molecular orbitals with the same symmetry, like $\ce{\sigma_g(2s)}$ and $\ce{\sigma_g(2p)}$ can overlap. The result is that $\ce{\sigma_g(2s)}$ will decrease in energy while $\ce{\sigma_g(2p)}$ will increase in energy. The same thing happens for the antibonding orbitals with $\sigma_u$ symmetry.
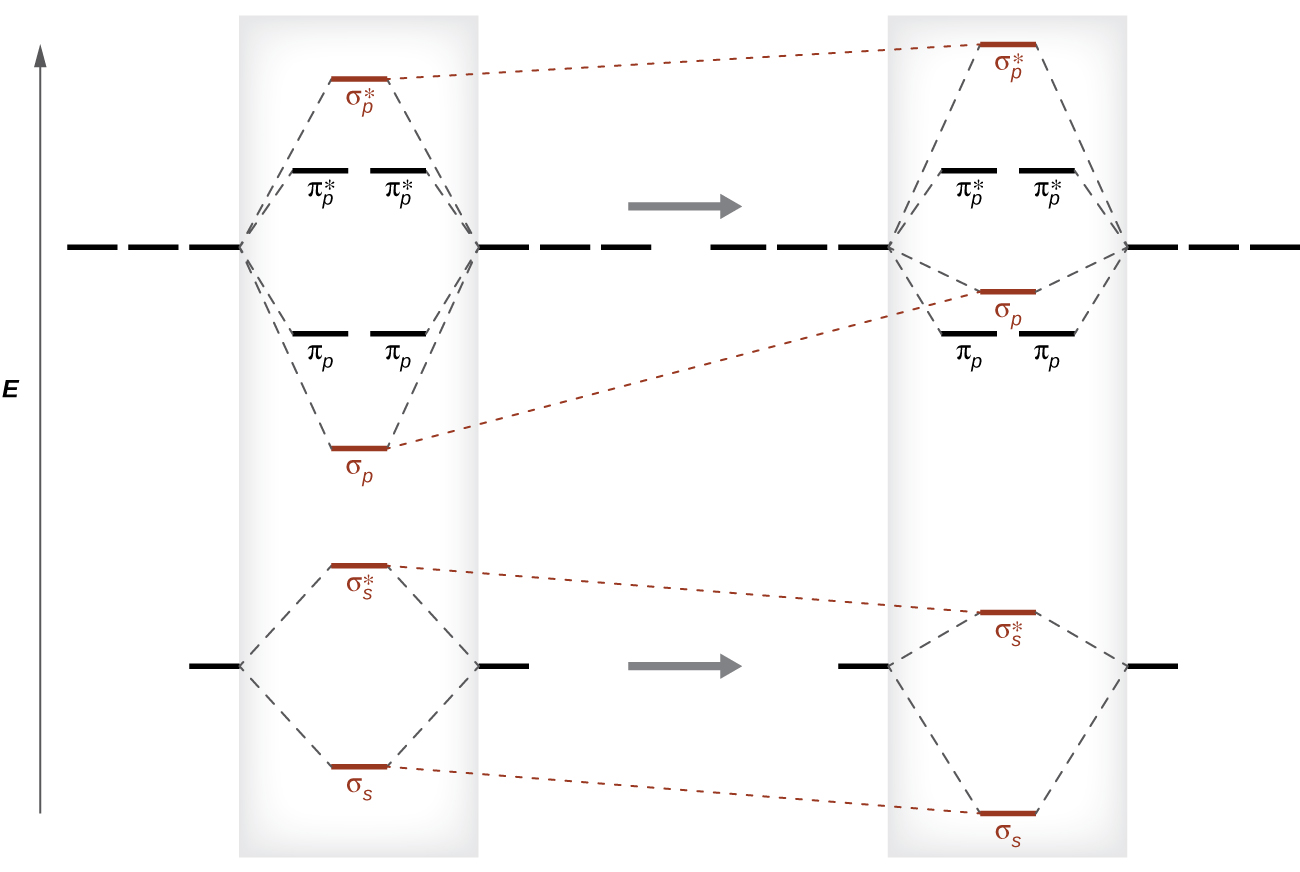sp-mixing: Left diagram shows the MO orbital diagram for s and p orbitals without sp-mixing, while the right diagram shows one possible configuration after considering sp-mixing.
As the effective nuclear charge increases, the sp mixing is smaller due to the larger energy difference between the $\ce{2p}$ and $\ce{2s}$ orbitals. Therefore, for $\ce{O2}$, $\ce{F2}$ and theoretical $\ce{Ne2}$, you see the characteristic molecular orbital diagram shown on the left. However, with diatomic elements like $\ce{N2}$ and theoretical diatomic molecules with lower atomic numbers such as $\ce{C2}$ and $\ce{B2}$, you get more orbital mixing. That means the $\ce{\sigma_u(2p)}$ would increase more in energy, which is why you may see the right molecular orbital diagram representing certain diatomic molecules.
-
$\begingroup$ The left diagram is - if not completely and utterly wrong - at the very least incomplete. $\endgroup$– Martin - マーチン ♦Commented Nov 12, 2022 at 13:14
-
1$\begingroup$ @Martin-マーチン - the left diagram omits the 2s orbitals, but I have seen diagrams like this which focus on the 2p alone because of this unusual orbital energy ordering (e.g., in $\ce{N2}$. This answer is +1 from me. $\endgroup$ Commented Nov 13, 2022 at 0:09
-
$\begingroup$ @GeoffHutchison I was fully aware of this. But how do you explain orbital mixing, if you omit half of the originals that mix? $\endgroup$– Martin - マーチン ♦Commented Nov 13, 2022 at 10:57
-
$\begingroup$ @Martin-マーチン - I'm not justifying the diagram. I think the whole valence picture is necessary. So I completely agree with "incomplete" but I disagree with "completely and utterly wrong" .. I think that goes too far. $\endgroup$ Commented Nov 14, 2022 at 18:14
-
1$\begingroup$ @GeoffHutchison You are right and I have overreacted. I'm too easily annoyed by misleading depictions like the one in the question. However, this shouldn't be the place to comment this and it wasn't helpful. Especially given this being the answer. I'll be more careful in the future. $\endgroup$– Martin - マーチン ♦Commented Nov 14, 2022 at 21:53