Find the added volume of HCl so that the ions separate

Question

Suppose we have a 10mL solution that contains 0.02M $\ce{Ag^+}$ and 0.04M $\ce{Ba^{2+}}$. a) Find the necessary volume to be added of $\ce{HCl}$ to separate all the silver. (Assume to be separated if there is less than 0'1% of remaining silver in the solution of what it was initially)

So here I tried to do it using the constant of solubility that is given to be $Ks(AgCl)=1.8*10^{-10}$ noting that that new concentration of silver has to be 0'1% of the initial one, which means to me $[Ag^+]'=\frac{0.1}{100}[Ag^+]_0$ hence using the constant: $$Ks(AgCl)=[Ag^+]'[Cl^-]'=[Ag^+]'(s+c_{\text{added}})$$ because we have added some quantity to the solubility of $\ce{Cl-}$, and then since the constant is of the order of $10^{-10}$ we can approximate to $s\approx 0$: $$Ks(AgCl)=0.001[Ag^+]_0\cdot c_{\text{added}}\implies c_{\text{added}}=\frac{Ks(AgCl)}{0.001[Ag^+]_0}$$ but I don't think that's right since I don't get the answer that my books suggests.

Answer 1

This is an equilibrium shift problem in which adding $\ce{HCl}$ produces two different effects simultaneously:

(1) Change in total volume of solution.

(2) Change in moles of $\ce{Cl-}$ ions present in solution.

We'll start by defining equations for the equilibrium number of moles of each relevant species in the equilibrium system:

$$\ce{AgCl(s) <=> Ag+(aq) + Cl-(aq)}$$

Let:

$S$ represent $\ce{Ag+}$

$C$ represent $\ce{Cl-}$

$A$ represent $\ce{HCl}$

At equilibrium, (after HCl is added) the moles of silver and chloride ions are, respectively:

$$N_{\ce{S}}=N_{So}-x$$

$$N_{\ce{C}}=N_{Co}-x$$

We're told that at desired separation:

$$N_S=0.001\;N_{So}$$

Substituting above and solving for x:

$$0.001\; N_{So}=N_{So}-x\;\;\implies\;\; x=0.999\;N_{So}$$

Substituting in $N_C$:

$$N_C=N_{Co}-0.999\;N_{So}$$

We then use the relationship between $K_N$ and $K_{sp}$ to form this expression:

$$K_{N}=K_{sp}\;(V_S+V_A)^2=N_S\;N_C=(0.001\;N_{So})(N_{Co}-0.999\;N_{So})$$

Where $V_S$ is the volume of the solution before $\ce{HCl}$ is added, and $V_A$ is the volume of $\ce{HCl}$ added.

Since $\ce{HCl}$ is a strong acid, we can assume that it's dissociated completely and:

$$N_{Ao}=N_{Co}$$

$$V_A=\frac{N_{Ao}}{C_{Ao}}$$

So, we have:

$$\left(\frac{N_{Ao}}{C_{Ao}}+V_S\right)^2=\frac{(0.001\;N_{So})(N_{Ao}-0.999\;N_{So})}{K_{sp}}$$

Plugging in all the known values we're given:

$N_{So}=2*10^{-4}\;mol$

$K_{sp}=1.8*10^{-10}$

$V_S=0.01\;L$

$$\left(\frac{N_{Ao}}{C_{Ao}}+0.01\right)^2=\frac{(2\times10^{-7})(N_{Ao}-1.998\times10^{-4})}{1.8\times10^{-10}}$$

It's important to note that the volume of $\ce{HCl}$ required depends on how concentrated it is, so $C_{Ao}$ should be given.

For example, if we have $\ce{HCl}$ 0.01$\;$M, then we would get when solving for $N_{Ao}$:

$$N_{Ao}=2.006\times10^{-4}\;mol$$

And finally:

$$V_A=\frac{N_{Ao}}{C_{Ao}}=\frac{2.006\times10^{-4}\;mol}{0.01\;mol/L}=0.02006\;L=20.06\;mL$$

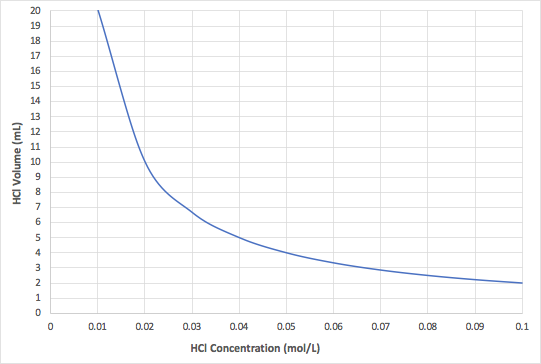
If you would like to observe how $V_A$ varies as a function of $C_{Ao}$, you can plot:

$$V_A=\frac{2000\;C_{Ao}-0.036\;-\sqrt{\left(0.036-2000\;C_{Ao}\right)^2-2.878416}}{3.6}$$

Note that $C_{Ao}$ is in mol/L, and resulting $V_A$ value will be in liters (L).

A plot of $V_A$ (mL) vs $C_{Ao}$ (mol/L) from 0.01M to 0.1M, would look like this:

Answer 2

BaCl2 is water soluble. AgCl is not. Adding HCl to the solution of 0.02M Ag+ and 0.04M Ba+ will produce an AgCl precipitate.

There are 10 ml of original solution.

10 ml × 0.02 mmol/ml Ag+ = 0.20 mmol (millimoles) Ag+

Therefor 0.20 millimoles HCl should be added to get at least 99.9% separation of AgCl.

Interestingly, with AgCl, adding excess chloride will actually increase the solubility of AgCl, so care must be taken to not exceed the proper amount.

1.0 ml of 0.2 M HCl will drop most of the silver as AgCl ppt.