Your example is one of many that have been studied by molecular beam scattering techniques so this is a rather general answer.
Collision theory calculates the rate constant averaged over all geometries and energies at at given temperature. It is the product of cross section, average collision velocity and the Arrhenius factor. Thus it depends on the cross section. The simplest model assumes hard spheres so will work for atom-atom, atom -molecule etc. but less well as the species become more complex. But actually the cross section is very complicated especially as the potential between molecules varies with distance and orientation perhaps as coulomb potential or Lennard Jones or something more complicated. In this case The trajectories of the interaction between species has to be calculated and these averaged to get the collision theory rate constant.
In fact the rate constant is not that important what is important is the potential energy profile between species as this reflects the electronic properties of the molecules and can be calculated from Quantum Theory. The rate constant can then, in principle, be calculated from the potential although experiment is always essential.
Experiments of this sort are studied using atom/molecular beams and numerous reactive scattering reactions have been studied such as H+H2, D+H2, N+O2, F+H2,H+F2, O+CH, O+Cs and many more.
You should look at two excellent books on this topic Levine & Bernstein 'Molecular Reaction Dynamics, and Chemical Reactivity' publ OUP 1987, and Steinfeld, Francisco & Hase, 'Chemical Kinetics and Dynamics', publ Prentice Hall 1999.
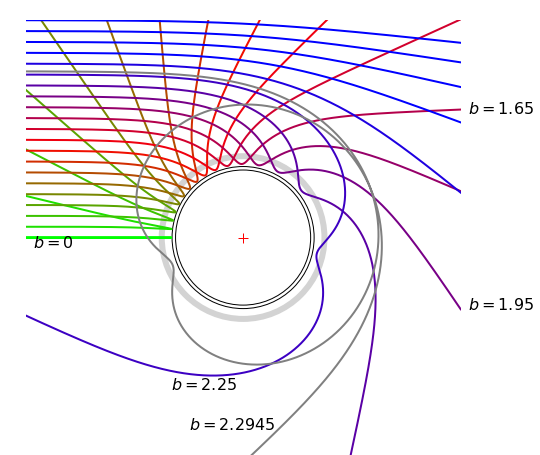
The picture below shows how complicated the trajectories become when there is a Lennard jones potential between two atoms as one approaches the other from the left but at different position vertically. You can see that the species get pulled together at a larger separation than hard sphere (the inner circle) but can orbit and escape if there is enough energy. In this picture no reaction occurs but you can appreciate if the species get close enough with enough energy a condition could be made to make them react, for example being within the wide grey ring.
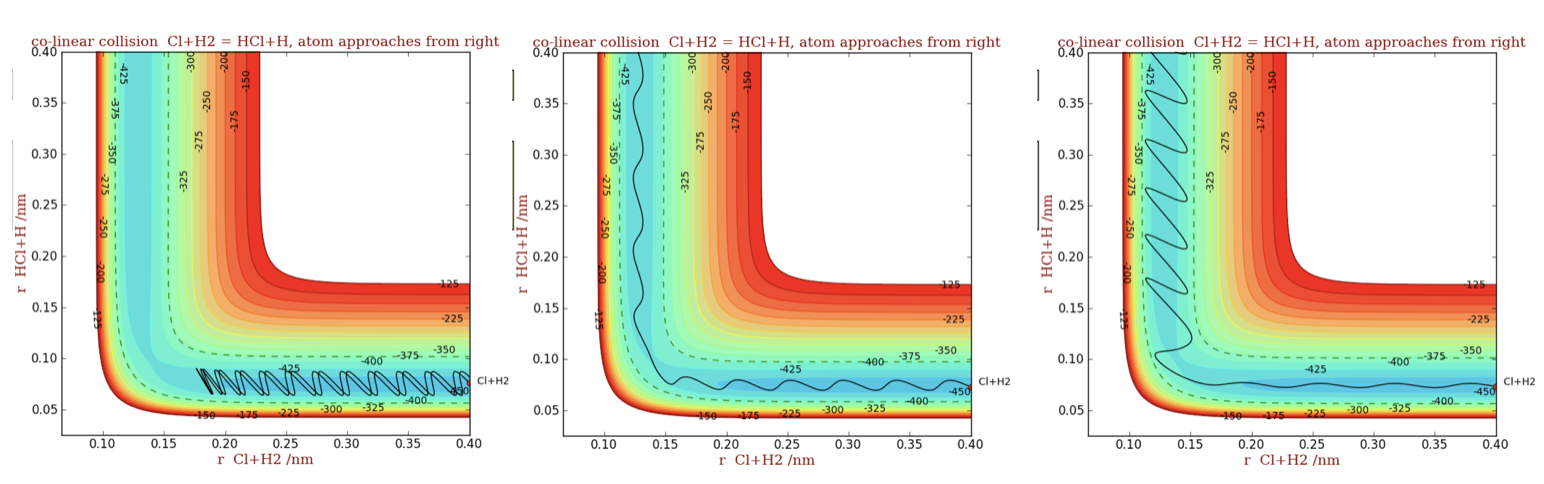
The picture below shows the Cl+H2 $\to$ HCl+H reaction. The species approach with the same energy in each case but collide at different point in the H$_2$ vibration and this leads to different outcomes, i.e no reaction or more of less vibrational excitation in HCL. The blue colour is low energy and the transition state can be seen at the bend. To calculate the rate constant the number of successful transition state crossings vs. total number at a given energy must be calculated. This could then be compared with the collision theory rate calculation and an effective cross section derived.