In a typical covalent bond, such as is found between carbon and hydrogen in
methane, each atom is considered to contribute one electron to the two-electron,
two-centre bond which is formed. The metal-ligand bond is often envisaged as a second type of covalent bond in which we still have a two-centre, two-electron bond, but where
both of the electrons come from one of the atoms or from a molecule. This type of
bond is known variously as a coordination, a dative covalent or a donor-acceptor
bond.
It was recognized early on that the formality of dative covalency in coordination
compounds presents some difficulties. Many inorganic compounds are conveniently
thought of as ionic salts in which there is an essentially complete charge separation
between cationic and anionic species. Compounds of the group 1 and group 2 metals
tend to be readily categorized as ionic. Dissolution of a salt of a group 1 or a
group 2 metal results in the formation of solutions containing solvated cations and
anions - only in concentrated solutions are there significant cation-anion
interactions beyond simple ion-pairing. However, the ionic model does not appear
to be suitable for the description of the properties of many transition-metal
compounds. For example, the compound $\ce{K4[Fe(CN)6]}$ dissolves in water to give
solutions containing solvated potassium ions and the $\ce{[Fe(CN)6]^{4-}}$ ion, rather than
solvated potassium, iron(II) and cyanide ions. The interactions between the cyanide
and the iron(II) centre appear to result in longer lived species than result from
simple electrostatic interactions of the type observed in sodium chloride. This is, of
course, the sort of argument which led to the development of the description of
coordination compounds in terms of donor-acceptor interactions between the
ligands and the metal centre.
Let's have a look at the consequences of the formation of a donor-acceptor
bond in a little more detail. If the donor-acceptor bond is completely covalent,
then there is a net transfer of one unit of charge from the donor to the acceptor as
a direct consequence of the equal sharing of the electron pair between the two
centres. This result leaves a positive charge on the donor atom and a negative
charge on the acceptor atom. The limiting ionic and covalent descriptions of a
complex cation such as $\ce{[Fe(H2O)6]^{3+}}$ are shown below.

As commented above 'ionic' structures are not in accord with the
chemical properties of coordination compounds. However, there are also a number
of objections to the covalent description. The charge distribution is such that the
iron(III) centre bears a three minus charge, whereas the oxygen atoms of the water
ligands each bear a single positive charge. This would be unrealistic in view of the
electronegativities of these elements ($\ce{Fe}$, 1.8; $\ce{O}$, 3.5), which predict that the $\ce{Fe-O}$ bond should be polarized in the sense $\ce{Fe^{\delta +}-O^{\delta -}}$. The problem was addressed by Pauling, who recognized that, in reality, it was not appropriate to describe most bonds as being purely 'ionic' or purely 'covalent'.
He developed a description of the bonding between a metal and its ligands which included considerable ionic character in the metal-ligand bonds within a basically covalent regime. In order to determine the amount of ionic character within a given metal-ligand bond, Pauling framed his electroneutrality principle. In this, he opined that the actual distribution of charges within a molecule is such that charges on any single atom are within the range -1 to +1.
It can be seen how this works by reconsidering the ion $\ce{[Fe(H2O)6]^{3+}}$. An ionic
description results in a +3 charge on the metal centre, whilst a covalent description
gives the metal a -3 charge. Now the electroneutrality principle suggests that the
ideal charge on the metal centre is zero. That would be achieved if the iron centre
gains a total of three electrons from the six oxygen donor atoms; in other words, if
each oxygen loses one half of an electron rather than the whole electron which the
fully covalent model demands. Pauling describes this situation as 50%
covalent (or 50% ionic).
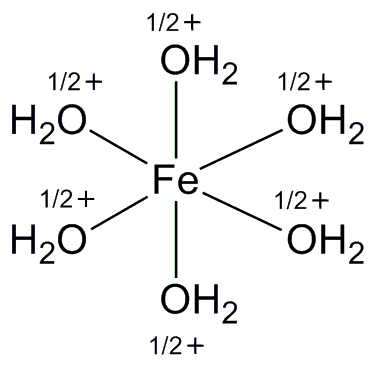
Pauling's model gives a good indication that the bonding situation in transition metal complexes is rather complex since the bonds are highly polarized (An 'accurate' view of the bonding in transition metal complexes can only be gained by more sophisticated methods such as the molecular orbital method).
Thus, Lewis structures are not particularly well suited to depict the bonding situation in coordination compounds because Lewis structures are intended for covalent compounds only.


