20
$\begingroup$

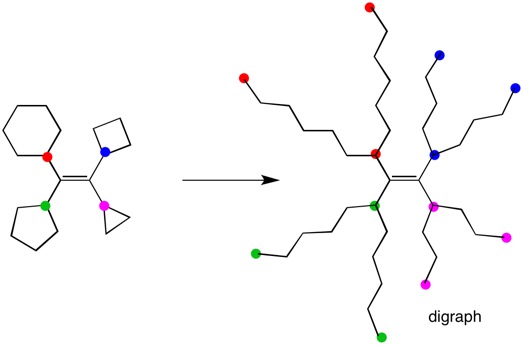
Here's what I read on Wikipedia (section "Cycles"):

To handle a molecule containing one or more cycles, one must first expand it into a tree (called a hierarchical digraph by the authors) by traversing bonds in all possible paths starting at the stereocenter. When the traversal encounters an atom through which the current path has already passed, a ghost atom is generated in order to keep the tree finite. A single atom of the original molecule may appear in many places (some as ghosts, some not) in the tree.

I don't understand how to assign CIP priorities to cyclic systems, for example, consider this:

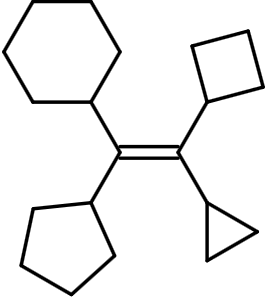
How do we decide the CIP priorities? What does that paragraph I found on Wikipedia really mean? Could someone please give a detailed explanation?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
27
$\begingroup$

I presume you want to know the E/Z geometry of the double bond. You need to determine on each end of the double bond which ring has priority. Intuitively one may argue that cyclohexane is larger than cyclopentane and cyclobutane is larger than cyclopropane. In this simple case, this is true and the double bond has the Z-configuration. However, digraphs are used in more complex cases. Your structure below has four colored dots. Each attached ring bond is "cut" and stretched out until one returns to the original atom. The three red atoms from the cyclohexane ring are identical. The same is true of the other three rings. Now treat the digraph as you would any acyclic alkene. The digraph has the Z-configuration.

Addendum: For two more complex cases, go here and here.

enter image description here

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Is the ghost atom counted or not by that I mean if we were to compare cyclo hexane and meth-Di(pentane)yl which would have a higher priority? $\endgroup$
    – Avyansh Katiyar
    Commented Jan 19, 2018 at 5:57
  • 4
    $\begingroup$ @AvatarShiny The ghost atom is counted as an atom of the same atomic number as its non-ghost counterpart but connected to nothing. Taking the fourth atom of one of the green chains and comparing it to the fourth atom of one of the red ones, both have C, H, H. We need to dive down another layer to find a difference. The red C (fifth in the chain) is connected to C, H, H. The green C is a ghost connected to nothing. Since $\ce{C}>\text{nothing}$, the red chain wins. $\endgroup$
    – Jan
    Commented Jan 19, 2018 at 8:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.