Why does ice melt slower at higher altitudes and how does Le Chatelier's principle play a role in this?
This question appeared right after this chapter in my text book and I've also read a very rough explanation from another source which cited this principle.
For a simplified answer that attempts at a solution using Le Chatelier's principle, you'll need a constraint: Ice and water are at an equilibrium at $\pu{0^\circ C}$.
$$\ce{Ice <=> Water}$$
Simple enough, now pull up the values of densities of ice and water, $\rho_{ice}= 0.9167~\pu{g/cm3}, \rho_{water}= 0.9998 ~\pu{g/cm3}$. Mass being the same, you can conclude that volume of ice is greater than that of water, so in accordance with Le Chatelier's principle, when you move at higher altitudes, the atmospheric pressure falls, thus the equilibrium is favored towards a side where the species occupy a higher volume, i.e. towards the formation of ice. This works antagonistically for $\ce{Ice->Water}$.
Alternatively, have you heard of phase diagrams? This might help you to understand things a bit more clearly after you go through its definition from other sources: https://chemistry.stackexchange.com/a/72732/43942
The phase diagrams for water are different though.
https://d2gne97vdumgn3.cloudfront.net/api/file/m1GFJVbQSBmwkDXRvglV
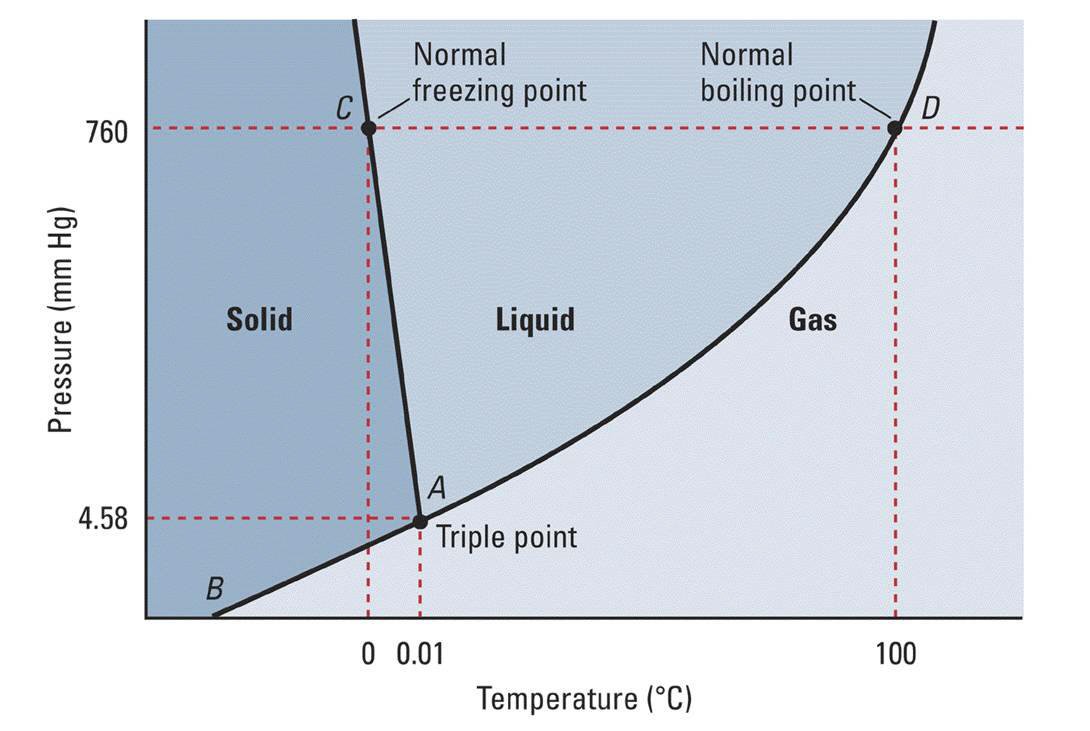
This, too indicates that for water, at lower pressures, the formation of solid (i.e. ice) is more favored.
To be more realistic, as MaxW pointed in the comments, the pressure difference is rather small. The summit pressure at Mt. Everest is $\pu{33.7 kPa}$, while at the sea level it is $101.3$, which gives us a difference of $\pu{67.6 kPa}$ which converts to a mere $\pu{0.676}$ Bar.
Although this is quite a significant pressure (see Jon's comment) for our perception, it doesn't affect freezing that much. For a comparison, this answer https://chemistry.stackexchange.com/a/8883/43942 states that the pressure required to solidify $\ce{CO2}$ to make it into dry-ice at room temperature, you'll need a pressure of about $\pu{56 bar}$.
Freezing at higher latitudes, requires us to also ponder on the ambient temperature. You probably wouldn't want to talk about latitudes as high as the Everest, because then the temperatures will be extremely low (averaging about $\pu{-19^\circ C}$ in summers!) and you'll have to apply an external pressure to adjust the melting point. Latitudes where the ambient temperature is (say) $\pu{3^\circ C}$ have a less temperature gradient when compared to a place (probably at the sea level) with an ambient temperature of (say) $\pu{25^\circ C}$. A higher magnitude of a gradient means a high rate of heat transfer, which hints at a slower melting of ice at higher latitudes.
Also, airhuff suggested that thermal conductivity of thin air at high latitudes may have a role to play in this. I searched this up, you might want to look at this paper: http://nist.gov/data/PDFfiles/jpcrd269.pdf
Easy. As you get higher, there is less air, making the area colder. So, the ice is surrounded by colder and colder tempatures.
