Correlation Diagram Doesn't Match Selection Rules?

Question

From Miessler's Inorganic Chemistry, 5th edition, there is this correlation diagram for $d^{2}$ in a octahedral field.

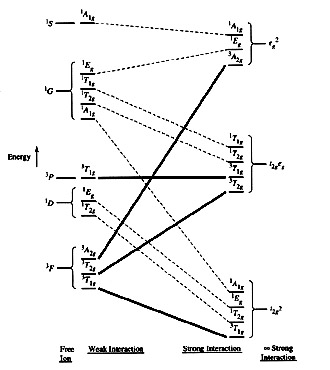
On the left hand side, if it's not clear, it goes (from lowest to highest):

$^{3}F, ^{1}D, ^{3}P, ^{1}G, ^{1}S$.

But by Hund's rules mentioned even in the same chapter, one would expect the $^{3}P$ to show up right after the $^{3}F$ because of their higher spin multiplicities. And even after that, G should come before D. (the ordering for that being s,p,d,f,g in reverse for same spin multiplicities). I don't know of any reason for why the $^1D$ term is just above the $^3F$ term in this graph though. Is this an error or does anyone have an explanation?

Answer 1

No, it's not an error. Take a look at the Tanabe Sugano diagram for $d^2$ (from Wikipedia):

You can see that again, the $^1D$ state is below the $^3P$ state. Why? If we were considering a $p^2$ configuration, the $^3P$ is indeed lower in energy than $^1D$. And again anyone might predict that $^3P$ is lower for $d^2$ too, for the exact reasons you give.

We know this result from spectroscopy. The difference is because of the Racah parameters for the different terms (i.e., the electrostatic repulsion between electrons in the same orbital. The Wikipedia article had a nice link to how you'd compute the difference in this case.

Now of course a $^3F \rightarrow ^1D$ transition is not an allowed transition, which is shown in the textbook on the next page.

The moral of the story is that Hunds' rules and the basics will always get you the lowest energy term symbols for multi-electron atoms, but the details can be tricky for higher-level states.