26
$\begingroup$

How are the number of tetrahedral voids twice the number of octahedral voids in a CCP structure? Is there some kind of mathematical proof for it? Is there some way I can understand this intuitively?

I have been trying to think about it using this picture but I cannot seem to get anywhere.

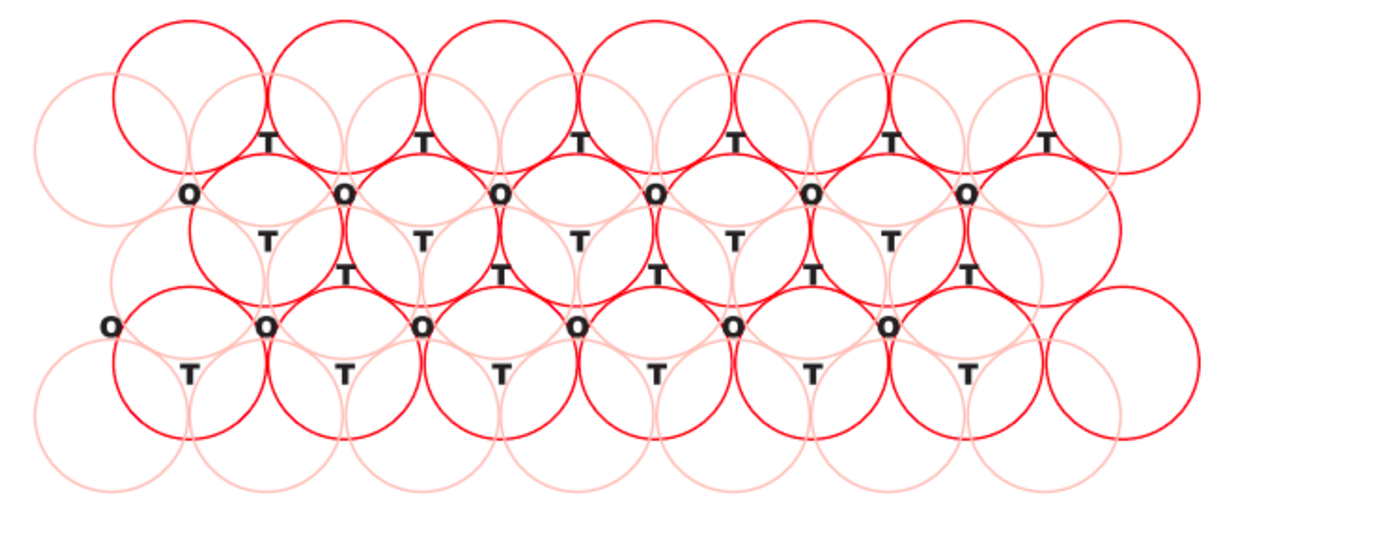
enter image description here

Improve this question
$\endgroup$
7
  • $\begingroup$ I'd start with counting how many neighboring T and O each sphere has. $\endgroup$
    – Ivan Neretin
    Commented Feb 19, 2017 at 16:48
  • $\begingroup$ So, each sphere has three T and three O voids. Then? $\endgroup$
    – Arishta
    Commented Feb 19, 2017 at 16:50
  • $\begingroup$ Should be much more than that. Don't you miss the voids formed by the next layer (the one on top of this)? $\endgroup$
    – Ivan Neretin
    Commented Feb 19, 2017 at 16:52
  • $\begingroup$ If we place the next layer on the T's, then all the T's will get filled, and we will only be left with O's. So, any sphere in the second layer will have 3O's. $\endgroup$
    – Arishta
    Commented Feb 19, 2017 at 16:59
  • $\begingroup$ No, the T's won't get filled. If anything, they will get completed. $\endgroup$
    – Ivan Neretin
    Commented Feb 19, 2017 at 17:12

2 Answers 2

Reset to default
16
$\begingroup$

To be precise, if in a close packed structure (ccp or fcc) there are $n$ atoms or ions then the number of octahedral voids and tetrahedral voids will be $n$ and $2n$ respectively.

For example, there are 8 tetrahedral voids per unit cell of fcc structure $(Z_{\text{eff}}=4)$. If you divide the FCC unit cell into 8 small cubes then each small cube has 1 tetrahedral void located at its own body center. Thus, total number of TVs in a unit cell $= 8 = 2 \times Z_\text{eff}$

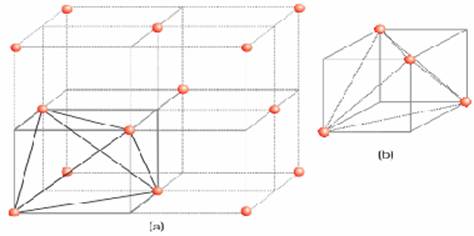
diagram of fcc unit cell

Now, again consider a CCP or FCC unit cell. The body centre of the cube is not occupied but it is surrounded by 6 atoms (4 in same plane, 1 above and 1 below). On joining these face centers, an octahedral void is formed. Thus, number of OV at body centre of the cube is 1.

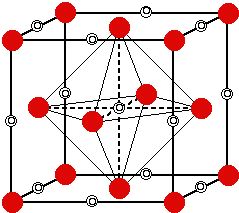
illustration of above paragraph

Besides the body center, there is one OV at the center of each of the 12 edges. It is surrounded by 6 atoms, three belonging to the same cell unit (two on the corners and once on face center), and the three belonging to two adjacent unit cells.

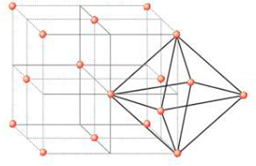
illustration of above paragraph

Since, each edge of the cube is shared between four adjacent unit cells only one fourth of each void belongs to a particular unit.

Therefore, OV at body centre = 1

OV at the 12 edges of the cube and shared between four unit cells $= 12\times\frac{1}{4}=3$

Total number of OVs $= 1 + 3 = 4 = Z_\text{eff}$

Improve this answer
$\endgroup$
5
  • $\begingroup$ How many other octahedral voids surround a n octahedral void ...I tried to do this but I am getting confused $\endgroup$
    – Hydrous Caperilla
    Commented Jul 4, 2018 at 13:28
  • $\begingroup$ Try to count the voids around the central octahedral void. Its much easier to visualize. $\endgroup$
    – Mitchell
    Commented Jul 4, 2018 at 20:22
  • $\begingroup$ Well the octahedral void is surrounded by 12 other octahedral voids according to the diagram in it's own unit cell $\endgroup$
    – Hydrous Caperilla
    Commented Jul 5, 2018 at 0:02
  • $\begingroup$ One more thing.Considering the spheres at one face centre which forms a square with the other face centre in it's plane,will only adjacent face centres touch it and the face centre at "a" distance from it won't touch the face centre opposite to it? $\endgroup$
    – Hydrous Caperilla
    Commented Jul 5, 2018 at 0:36
  • $\begingroup$ Can you please answer my question as I am confused here.......will help me explain the number of neatest neighbours in FCC....... $\endgroup$
    – Hydrous Caperilla
    Commented Jul 6, 2018 at 10:27
5
$\begingroup$

Let $E$ and $F$ be the planes that contain the centers of the spheres of the first and second layer, respectively. We notice that neither the centers of tetrahedral or octahedral voids lie in these planes.

Thus, when counting the tetrahedral and octahedral voids in a cubic or hexagonal close packaging of equal spheres, it is sufficient to count the voids that are centered in the space between $E$ and $F$.

If we regard a fixed sphere, we find four tetrahedral and three octahedral voids next to it and between $E$ and $F$.

Since each tetrahedral void is adjacent to four spheres, while each octahedral void is adjacent to six spheres, we get $4 \cdot \frac{1}{4} = 1$ tetrahedral and $3 \cdot \frac{1}{6} = \frac{1}{2}$ octahedreal void per sphere.

Improve this answer
$\endgroup$
0

Not the answer you're looking for? Browse other questions tagged or ask your own question.