No 6-centre-10-electron bond.
The bonding situation in $\ce{IF7}$ is explained by Christe et. al. (see ssavec's answer) as a 6-centre-10-electron bond. In the article itself I found no real evidence that supports this statement and I believe it to be completely wrong. The following section is taken from Karl O. Christe, E. C. Curtis, David A. Dixon, J. Am. Chem. Soc. 1993, 115 (4), 1520–1526. and might as well be the culprit of this monstrosity.
The bonding in the square-planar $\ce{XeF4}$, can be described by two semi-ionic, 3-center 4-electron (3c-4e) bonds for the four $\ce{Xe-F}$ bonds and two lone valence electron pairs on $\ce{Xe}$ ($\mathrm{s^2p}_z^2$ hybrids). The 3c-4e bonds involve the $\mathrm{p}_x^2$ and $\mathrm{p}_y^2$ orbitals of xenon. Addition of an $\ce{F-}$ ion to the equatorial plane in $\ce{XeF4}$, results in pentagonal-planar $\ce{XeF5-}$ and the formation of a semi-ionic, 6-center 10-electron (6c-10e) bond involving the delocalized $\mathrm{p}_x^2\mathrm{p}_y^2$ hybrid orbitals of $\ce{Xe}$ and six electrons on the five $\ce{F}$ ligands. The two lone valence electron pairs on $\ce{Xe}$ in $\ce{XeF5-}$ are analogous to those in $\ce{XeF}$.
The planar $\ce{IF5}$ fragment of $\ce{IF7}$, has essentially the same bonding as $\ce{XeF5-}$, as shown by the atomic population calculations given in Table VII. [...]
They continue to compare the populations of the valence orbitals in question, without stating the what methods they used to determine that.
The electronic structure calculations were done at the ab initio molecular orbital (MO) and local density functional (LDF) levels. [...]
A more accurate description of the bonding in in $\ce{IF7}$
Iodine heptafluoride is one of the classic examples when it comes to describing hypervalency and octet-expansion. Early attempts included d-Orbitals into describing that phenomenon (VSEPR), resulting in a description that yields the correct geometry. We now know that this theory is obsolete and more often wrong than correct.
A very principle problem in describing the bonding in this molecule is assuming that it is completely covalent bound. Christe et. al. acknowledge that this is wrong by stating "semi-ionic" bonds. This is - as we will see - already a stretch.
A more accurate terminology for these kind of molecules is hyper-coordinated; i.e. the central atom has a higher coordination number than common.
The following analysis utilises a calculation on the local density approximation level of theory. The used basis set is of triple zeta quality and employs a small effective core potential for iodine. The density fitting approximation was used to somewhat speed up the calculations. This is often denoted as DF-BP86/def2-TZVPP. The program used is Gaussian 09 rev. D.
Geometry
The performance of the method is not overwhelming. The bond lengths are overestimated by quite a bit. As cited by Christe, observed is $r_\mathrm{obs}(\ce{I-F_{ax}})=178.1~\mathrm{pm}$ and $r_\mathrm{obs}(\ce{I-F_{eq}})=185.7~\mathrm{pm}$. I calculated $r_\mathrm{BP86}(\ce{I-F_{ax}})=190.4~\mathrm{pm}$ and $r_\mathrm{BP86}(\ce{I-F_{eq}})=195.6~\mathrm{pm}$. Other methods, that I could still reasonably perform did not do much better. The MP2 result is $r_\mathrm{MP2}(\ce{I-F_{ax}})=186.6~\mathrm{pm}$ and $r_\mathrm{MP2}(\ce{I-F_{eq}})=191.9~\mathrm{pm}$.
For the purpose of the analysis the DFT method should be enough. (I had a look at the other results, too; they don't differ significantly.)
Quantum theory of atoms in molecules (QTAIM)
I would usually start with looking at the orbitals, but in this case it is more appropriate to look at the electron density itself. Better yet, we look at the Laplacian of the electron density. The depicted QTAIM plots are the Laplacian distribution within the molecule. Solid blue lines indicate charge depletion $\nabla^2\rho>0$, dashed red lines indicate charge accumulation $\nabla^2\rho<0$, red spheres are bond critical points, solid black lines are bond paths, dark red lines are zero flux surfaces (these separate the atoms). See an earlier answer of me for some more information on QTAIM.
We have two bond critical points to consider, one for the axial and one for the equatorial bond. We find low values of electron density at both; $\rho(\ce{I-F_{eq}})=0.125~\mathrm{a.\!u.}$; $\rho(\ce{I-F_{ax}})=0.137~\mathrm{a.\!u.}$. The Laplacian at both points is positive; $\nabla^2\rho(\ce{I-F_{eq}})=0.33~\mathrm{a.\!u.}$; $\nabla^2\rho(\ce{I-F_{ax}})=0.42~\mathrm{a.\!u.}$.
These values indicate predominantly ionic bonding. The graphs nicely show that the atoms appear as separate entities.
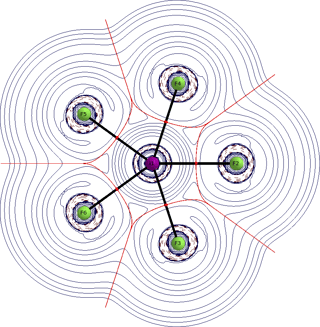
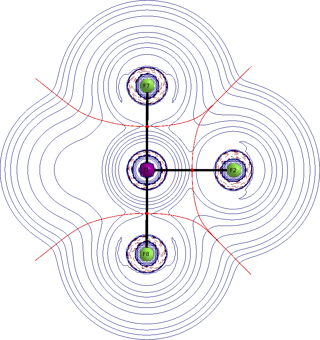
With that background it seems wrong arguing the bonding situation by forming covalent bonds, like they are assumed in MO or VB theory.
However, as wrong as it is to neglect ionic bonding, it is equally wrong to neglect covalent bonds.
The Orbitals of $\ce{IF7}$
There are a total of four orbitals with contributions from iodine and fluorine. There are not more than eight bonding electrons. I cannot imagine that Christe et. al. found a different solution, as his level of theory was not better (maybe worse). They either did not know how to interpret these results or simply overlooked it.
Here are the relevant lines from the output.
Alpha occ 17 OE=-1.196 is I1-s=0.23 F7-s=0.18 F8-s=0.18
Alpha occ 25 OE=-0.587 is I1-p=0.30 F7-p=0.28 F8-p=0.28
Alpha occ 26 OE=-0.568 is I1-p=0.31 F4-p=0.19 F3-p=0.19
Alpha occ 27 OE=-0.568 is I1-p=0.31 F2-p=0.21 F6-p=0.14 F5-p=0.14
(OE is the orbital eigenvalue in Hartree, the contributions missing from 1.00 are at the other atoms, but to small to trigger printing.)
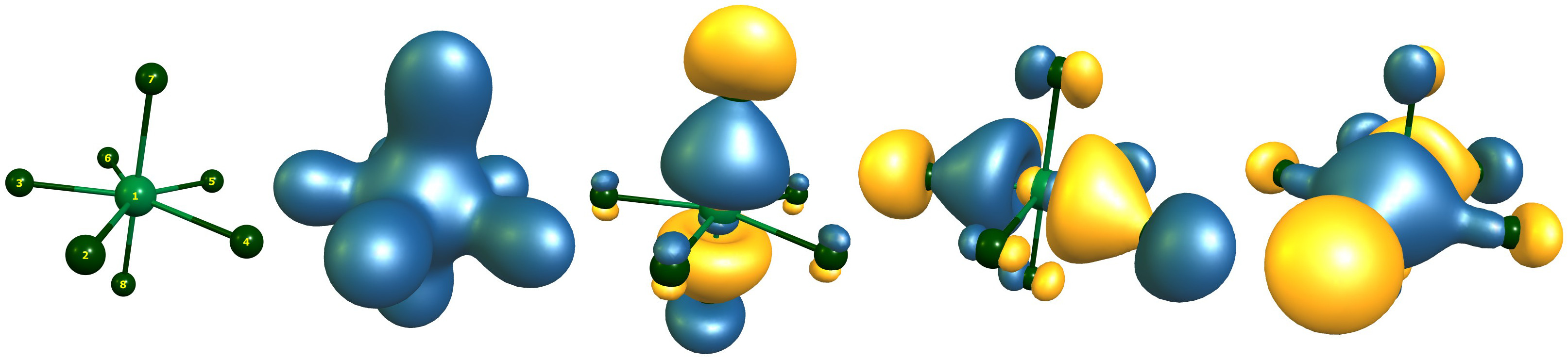
In the LCAO bonding picture we have a totally symmetric bonding orbital from all atoms, an orbital referring to the axial bonds, and two degenerate orbitals for the equatorial plane.
Charges and Wiberg Bond indices
The ionic character of the bonds can also be seen in the charge distribution. While the iodine has a high positive charge $q(\ce{I})=3.24$, the fluorines have a negative charge $q(\ce{F})=-0.46$. These are AIM charges calculated with the MultiWFN program package.
The Analysis with natural bond orbitals give a similar result, $q(\ce{I})=3.20$, $q(\ce{F})=-0.46$.
Looking at the Wiberg bond indices matrix formed from natural atomic orbitals, we find a bond index of $0.54$ for the equatorial bonds and a slightly higher value of $0.56$ for the axial bonds.
Conclusions
It is not possible to determine a number for how many sigma bonds are in the molecule, since this is strictly only possible for covalent bonds. The bonds are here predominantly ionic, with small covalent contributions. The number of bonding electrons does not exceed eight and therefore obeys the octet rule.
VSEPR fails, as it was expected. The term hyper-valency should be avoided and replaced by the term hyper-coordination.



