9
$\begingroup$

I don't know where they go the electrons of the extended-valence in molecules like $\ce{SF6}$ or $\ce{PCl5}$ for instance because my teacher said that the d orbitals can't interact with s and p (or hybridised $\ce{sp^3}$) as they are far away energetically. So if the hybridisations $\ce{sp^3d}$ and $\ce{sp^3d^2}$ don't exist, how does this work?

I've also read about it in "Petrucci Ed.11" and this is presented more or as an unsolved mystery that will continue creating controversy.

Do you know any paper that disapproves the $\ce{sp^3d^2}$ model?

Furthermore, there's a picture that shows the molecular orbitals of $\ce{SF6}$. I can't understand the process to deduce them but I hope this is a more accurate version of the molecule (rather than using hybridisation). It seems to demystify the octet rule and just focus on electron pairing. All this gives me a different vision of chemistry, like for example, what if we had diatomic alkaline metals? (I know that there are some calculations about them, but I'm not pretty sure if they can be made at the lab.)

https://en.wikipedia.org/wiki/Hypervalent_molecule

Improve this question
$\endgroup$
11
  • $\begingroup$ The easiest way around this is to invoke 3 center - 2 electron bonds. Alternatively, you can also have resonance with two ionic bonds in $\ce{SF6}$ or one in $\ce{PCl5}$, with appropriate formal charges on sulfur and phosphorus. $\endgroup$
    – Karsten ♦
    Commented Aug 31, 2022 at 10:28
  • 1
    $\begingroup$ @karsten 3-center 2-electron bobds? I thought it was 3-center 4-electron bonds. $\endgroup$
    – Oscar Lanzi
    Commented Aug 31, 2022 at 10:39
  • 1
    $\begingroup$ Wikipedia says about 3c-4e, yes $\endgroup$
    – Jorge Bonifaz
    Commented Aug 31, 2022 at 10:42
  • 1
    $\begingroup$ @JorgeBonifaz The common $\ce{PCl5}$ Lewis structure shows 10 electrons around the central atom. If two of the chlorine atoms and phosphorus are in a 3 center 4 electron bond, you only "need" 8 electrons from the central atom and stay within the octet. Describing it as $\ce{PCl4+ + Cl-}$ has the same effect. $\endgroup$
    – Karsten ♦
    Commented Aug 31, 2022 at 10:45
  • 1
    $\begingroup$ @OscarLanzi I read up on the nomenclature. Everyone says 3c-4e bonds, so sorry for the (my, mostly) confusion. I did not count the electrons in the non-bonding MO, but everyone else does, so I stand corrected. As Jorge points out, 3c-2e should be reserved to hypovalent molecules. $\endgroup$
    – Karsten ♦
    Commented Aug 31, 2022 at 13:14

2 Answers 2

Reset to default
11
$\begingroup$

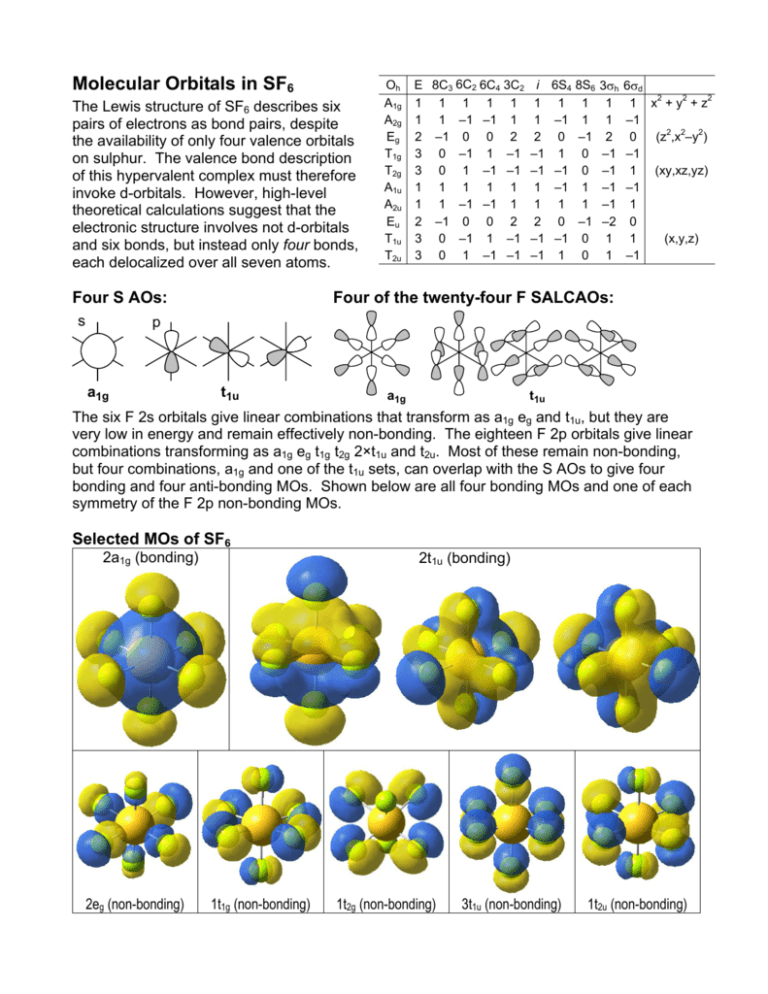
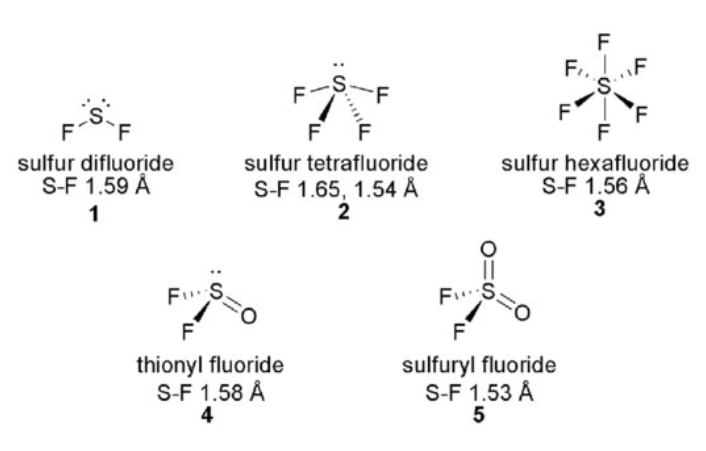
The starting point for a discussion should be the observed structures. $\ce{SF6}$ has a regular octahedral geometry with bond lengths of 1.56 Å. Below is a depiction compared with some other compounds showing fluorine sulfur bonds:

enter image description here Source: https://www.researchgate.net/publication/318478861_Sulfur_-_fluorine_bond_in_PET_radiochemistry/figures?lo=1

The structure of $\ce{PCl5}$ in a trigonal bipyramid:

enter image description here

Source: https://commons.wikimedia.org/wiki/File:Phosphorus-pentachloride-2D-dimensions.png

Trigonal bipyramids have distinct axial and equatorial ligands, but they can swap using the pseudorotation mechanism. While this is sometimes faster than the methods to characterize the molecules (e.g. the NMR spectrum of $\ce{PF5}$ suggest 5 identical ligands), the electrons are even faster "figuring out bonding", so we can ignore pseudorotation (Born-Oppenheimer approximation).

So those are the structures we would like to rationalize, with the simplest model possible (so it still fits in our head and we can be convinced by it - "just" solving the problem ab initio doesn't convince most folks).

[OP] Do you know any paper that disapproves the sp3d2 model?

  1. Chemical bonding in hypervalent molecules. The dominance of ionic bonding and negative hyperconjugation over d-orbital participation, Alan E. Reed and Paul v. R. Schleyer, Journal of the American Chemical Society 1990 112 (4), 1434-1445 DOI: 10.1021/ja00160a022
  2. Hypercoordinate molecules of second-row elements: d functions or d orbitals? Eric Magnusson, Journal of the American Chemical Society 1990 112 (22), 7940-7951 DOI: 10.1021/ja00178a014

[OP] So if the hybridisations sp3d and sp3d2 don't exist, how does this work?

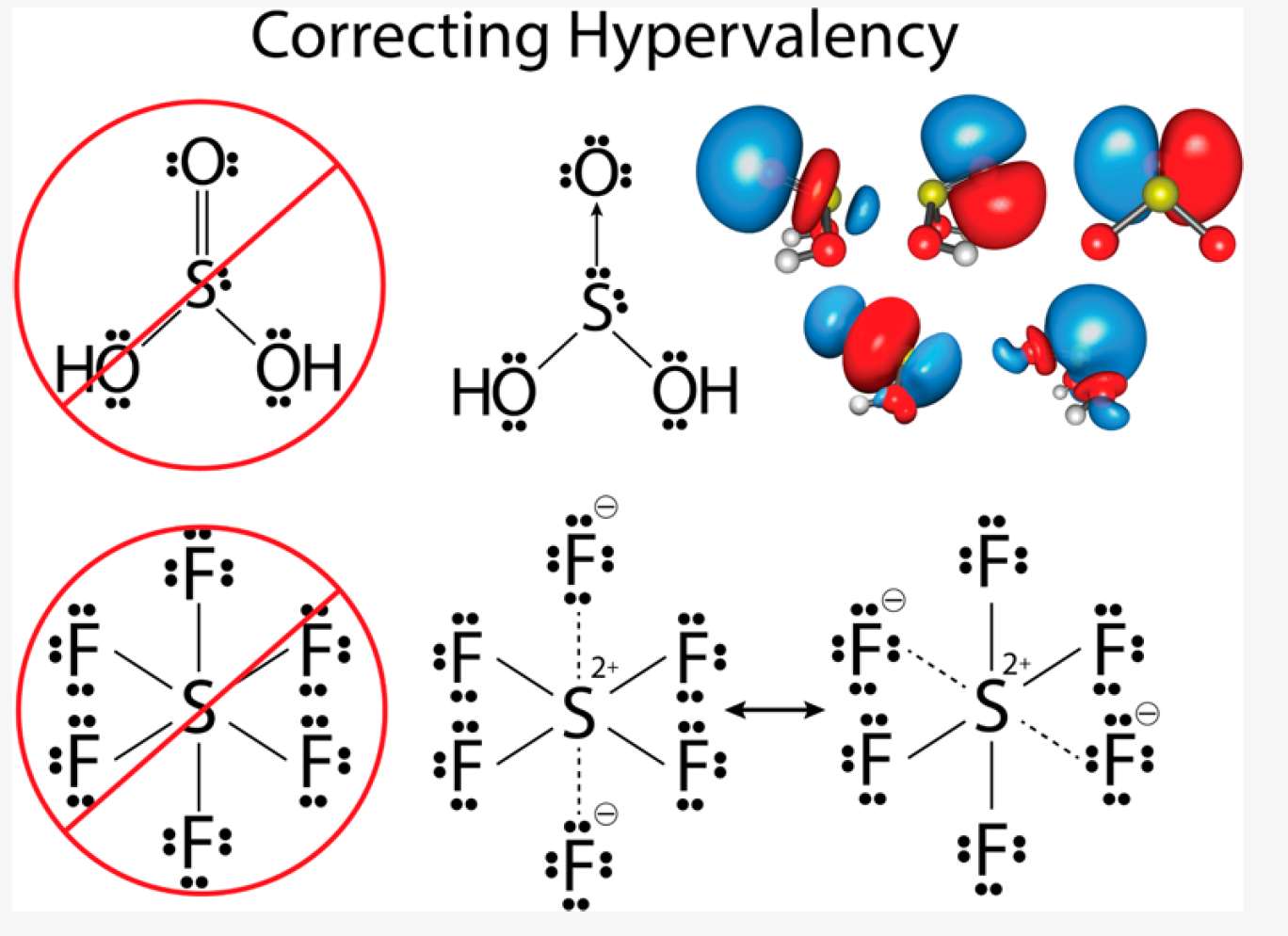
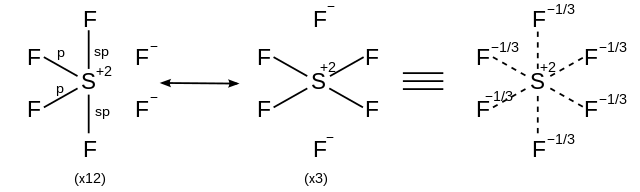
The logic is that if the only atomic orbitals on the central atoms you consider for bonding are s-orbitals and p-orbitals, you can't make more than 4 bonds (this also goes for sulfate and phosphate). If you want to stay close to Lewis structures, with a line representing two electrons, you have to invoke ionic bonds and resonance. For $\ce{SF6}$, you write $\ce{SF4^2+ + 2F-}$. This gives

enter image description here Source: Wikimedia via Wikipedia article on hypervalent molecule

The bonds are a combination of a covalent bond (bond order 2/3) and an ionic bond (attraction of 1/3 charge on fluoride with +2 charge on sulfur).

If you want to use MO-theory, you match the fluoride p-orbitals (the s-electrons don't participate) with the symmetry of the central atom's s-orbitals and p-orbitals (see illustration in the question). The four lowest combinations are shown below. If you use subtraction instead of addition, you get anti-bonding MOs (with more nodes; just like we learn for atomic orbitals, more nodes mean higher energy of electrons in that state).

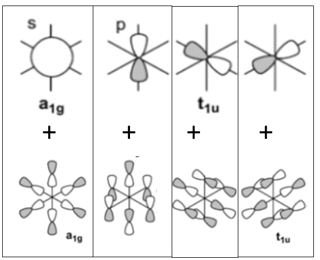
enter image description here

These get filled with the 8 electrons available. 8 electrons would make 4 standard localized bonds, but here you connect 6 ligands, so the bond order is 4/6 = 2/3). This matches the bond order of 2/3 for the VB model above (localized bonds plus ionic interaction).

If you compare the S-F single bond lengths in other molecules to the bond length in $\ce{SF6}$, they are all very similar, even though e.g. the bonds in $\ce{SF2}$ are "real" single bonds and we just said the bonds in $\ce{SF6}$ have a bond order of 2/3. This is rationalized by the partially ionic character of the latter.

The $\ce{PCl5}$ molecule can be explained in a similar manner. The bond order is 4/5.

[Oscar Lanzi in comments] PCl5: bond orders may be different for equatorial and axial atoms, I would use 1 and 1/2 respectively.

Improve this answer
$\endgroup$
8
  • $\begingroup$ Ok, thanks for the answer. I still have some questions. You said in a comment that the bond order was 5/6 but then 2/3. How can I calculate it by my own? $\endgroup$
    – Jorge Bonifaz
    Commented Aug 31, 2022 at 15:23
  • $\begingroup$ Sorry but I can't clearly understand where the electrons go when you use MO theory. $\endgroup$
    – Jorge Bonifaz
    Commented Aug 31, 2022 at 15:27
  • 1
    $\begingroup$ @JorgeBonifaz I fixed my comment above. Bond order for $\ce{PCl5}$ is 4/5 and for $\ce{SF6}$ 4/6. The 4 is always from having available one s and three p orbital on the central atom, and using all of them to make bonding MOs. $\endgroup$
    – Karsten ♦
    Commented Aug 31, 2022 at 16:04
  • 1
    $\begingroup$ I edited the answer, adding some more information. The MO theory will make sense when you study it from the ground up (this is more at the hand-waving level). The idea is that you can take N orbitals and mix and match them (linear combination) to get N combined orbitals, sometimes with different symmetry. $\endgroup$
    – Karsten ♦
    Commented Aug 31, 2022 at 16:07
  • 1
    $\begingroup$ And you said that there are 8 e- participating, from "s" and 3 "p" atomic orbitals (from sulphur), but it has only 6 valency e-, so does this mean that the other 2 e- are just picked from any fluorine atom so it's delocalized? $\endgroup$
    – Jorge Bonifaz
    Commented Sep 1, 2022 at 7:35
3
$\begingroup$

Hypervalency was discounted for sulfur and row 2 elements at least 20 years ago (see the references cited in an earlier answer and related citing works.) Such sulfur compounds have ionic character with the sulfur atom +2 and the negative balancing charge distributed over the more electronegative atoms with delocalized resonant bonds, or similar.

enter image description here

See "Addressing the Hypervalent Model: A Straightforward Explanation of Traditionally Hypervalent Molecules" J. Chem. Educ. 2020, 97, 3638−3646, https://dx.doi.org/10.1021/acs.jchemed.0c00368

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.