0
$\begingroup$

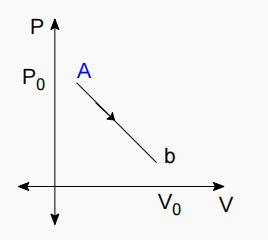
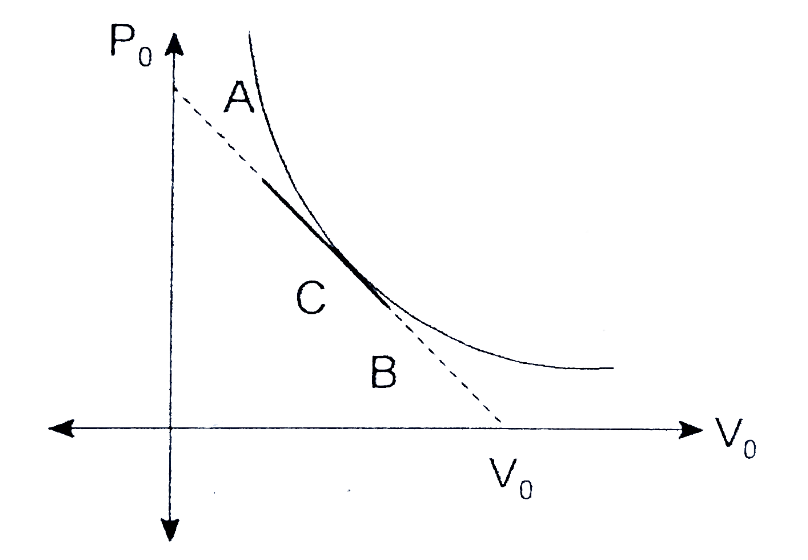
One mole of monoatomic gas undergoes a linear process A to B shown in P-V diagram. Volume of gas from where process turn from an endothermic to an exothermic is?

enter image description here

I tried to calculate the point where the rate of change of temperature wrt volume would become 0 but my answer didn't match. The solution given was: Process will turn from endothermic to exothermic at point where slope of P-V graph satisfy the slope of adiabatic curve for same gas. I couldn't understand why this is true. Could anyone please explain. Thank you.

Improve this question
$\endgroup$
1
  • $\begingroup$ dT/dV <> 0 for not reacting ideal gas during adiabatic processes. $\endgroup$
    – Poutnik
    Commented May 20 at 10:29

2 Answers 2

Reset to default
0
$\begingroup$

From the first law of thermodynamics, $$dU=dQ-dW$$ The process goes form endothermic to exothermic when dQ=0. This is also tangent to some adiabatic reversible curve for the gas.

Improve this answer
$\endgroup$
-2
$\begingroup$

Basically the slope of the adiabatic curve would satisfy the slope of the PV diagram, at a point where the curve and the PV line intersect (The PV line becomes tangent to the adiabatic curve).

pV diagram

Based on this graph,

$ -P0/V0 = - γP/V $

$ -m = - (γ(-mv + P0))m/V = + P0/V0 $

$ mv(1+γ) = γP0 $

$ V = γP0/m((1+γ) = 5V0/8 $

Hence the volume at which it will switch would be $ 5V0/8 $.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.