6
$\begingroup$

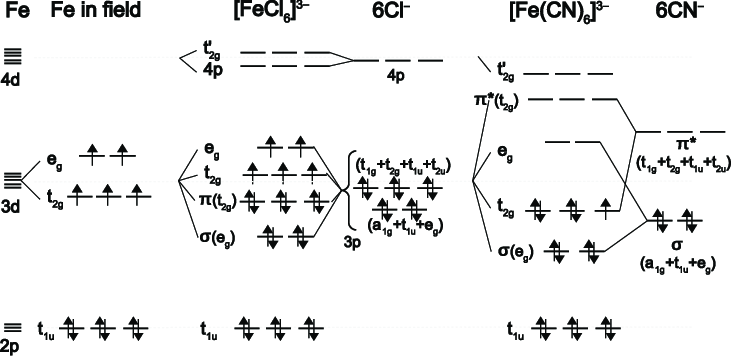
I am trying to explain the oxidizing property of $\ce{Fe^3+}$ in $\ce{H2O}$ and $\ce{CN^-}$ which is $\ce{[Fe(H2O)6]^3+}$ and $\ce{[Fe(CN)6]^3-}$.

The electrochemical reactions along with their standard reduction potentials are: \begin{align} \ce{Fe^3+(aq) + e- &<=> Fe^2+(aq)} \qquad E^\circ = \pu{0.77 V} \tag1 \\ \ce{[Fe(CN)6]^3-(aq) + e- &<=> [Fe(CN)6]^4-(aq)} \qquad E^\circ = \pu{0.36 V} \tag2 \\ \end{align} and the higher the standard reduction potential, the higher the equilibrium constant. I deduce this from \begin{equation} \Delta_\mathrm{r}G^\circ = -nFE^\circ = -RT \ln K \tag3 \end{equation}

Which could also give the result that $\ce{Fe^3+}$ in water oxidizes stronger than when the ion is in cyanide medium. I want to ask whether we can deduce the same way by using the molecular orbital of two complexes mentioned above?

enter image description here

In this picture, instead of water, chloride ion could be used. So I am thinking of predicting oxidizing strength using the energy of HOMO and LUMO. Could I do this?

My thought was that the $t_\mathrm{2g}$ in cyanide complex is lowered in energy than that of the chloride complex. So, if the electron was to be accepted, then the cyanide complex is prone to accept better. However, the reduction potential speaks otherwise.

Improve this question
$\endgroup$
4
  • $\begingroup$ @ananta-This one is correct, and it can also be said that if you are looking at standard oxidation potentials, higher values mean lower reducing power (from the mirror image view) $\endgroup$
    – user144302
    Commented Mar 10 at 7:42
  • 2
    $\begingroup$ I am a bit confused right now. If I read correctly, then wikipedia is wrong, is it not? en.wikipedia.org/wiki/Standard_electrode_potential It is stated "The larger the value of the standard reduction potential, the easier it is for the element to be reduced (gain electrons); in other words, they are better oxidizing agents." $\endgroup$
    – Shira
    Commented Mar 10 at 8:18
  • $\begingroup$ I reconsidered my comment, and the standard reduction potentials are indeed an indicator of the oxidizing power. Higher potential means the moiety gets reduced easily which means it some other moiety gets oxidized easily. $\endgroup$
    – ananta
    Commented Mar 10 at 19:05
  • $\begingroup$ In this context @ananta, we can also say that if you are looking at standard reduction potentials, higher values mean lower oxidizing power just as the larger the value of the standard oxidation potential, the easier it is for the element to be oxidized (lose electrons); in other words, they are better reducing agents." $\endgroup$
    – user145056
    Commented Apr 7 at 1:55

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.