Which make HCO3- to show two pH values at two scenarios?

Question

According to the below two titrations,

Image Reference

If we consider the reactions, at the first reaction (in first figure after adding 1.0), there is $\ce{HCO3-, NaCl,}$ and $\ce{H2O}$ at the first equivalence point. At the second one (after adding 1.0), there is $\ce{HCO3-,}$ and $\ce{H2O, Cl-}$ at the first equivalence point. $\ce{HCO3-}$ only affect to the $\mathrm{pH}$ of the medium. But,

• According to the first figure, $\ce{HA- + H2O → H2A + OH-}$ it acts as a base
• According to the second figure, $\ce{HA– + H2O → A2– + H3O+}$ it acts as a acid

I am doubtful on why do $\ce{HCO3-}$ perform as two types at these different situations? Do anything in the medium manipulate $\ce{HCO3-}$ to work as a base or an acid? How to describe these two scenarios? Generally I can't see a theoretical method for describe it.

I searched this in so many resources, but I couldn't find a satisfied answer. So, I hope a theoretical answer, for this problem, not a mathematical description.

Answer 1

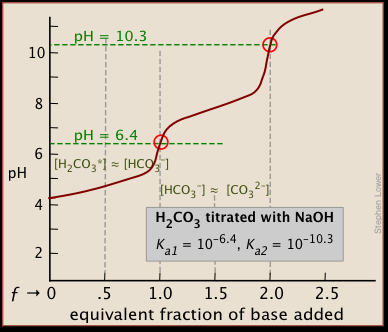
The problem is that both titration curves are wrong: at the first equivalence points, the $\mathrm{pH}$ does not equal $\mathrm{p}K_\mathrm{a1}$ and the $\mathrm{pOH}$ does not equal $\mathrm{p}K_\mathrm{b1}$. As well, the two provided dissociation constants for carbonate ion, in the OP's upper figure, are also wrong.

The figure below shows two titration curves: 1) for $\pu{10 mL}$ of $\pu{0.1 M}$ "carbonic acid", with $\pu{0.1 M}$ $\ce{NaOH}$ titrant (black rising curve) and 2) for $\pu{10 mL}$ of $\pu{0.1 M}$ sodium carbonate solution, with $\pu{0.1 M}$ $\ce{HCl}$ titrant (red falling curve):

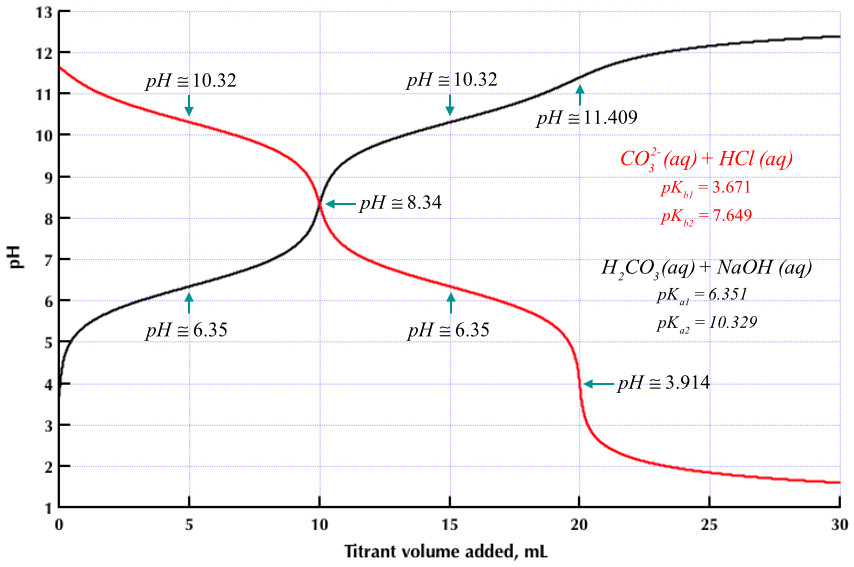
The $\mathrm{pH}$ of the starting $\pu{0.1 M}$ carbonic acid is 3.67 and the $\mathrm{pH}$ of the starting $\pu{0.1 M}$ sodium carbonate is 11.65. At the first equivalence point, for both titration curves, the $\mathrm{pH}$ is approximately 8.34. The $\mathrm{p}K_\mathrm{a}$ values are as shown in the figure and are from (or derived from) the reference at the bottom.

The next two figures show Excel spreadsheet $\mathrm{pH}$ calculations. The first one shows that $\mathrm{pH} = 8.338$ at the first equivalence point, for the neutralization of sodium carbonate solution with $\ce{HCl}$:

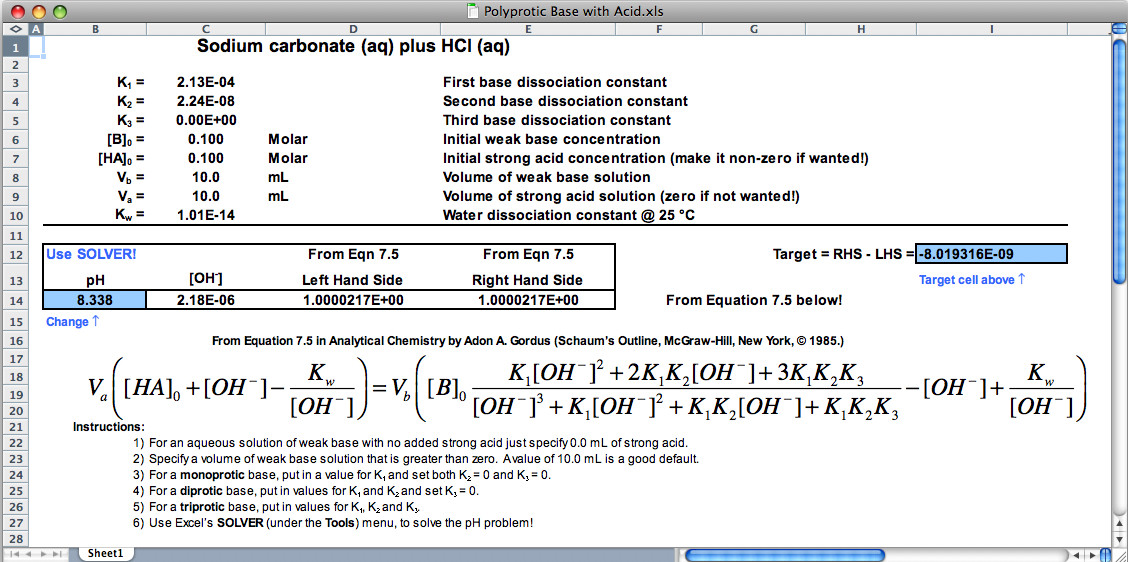
At the first equivalence point, the solution is $\pu{0.05 M}$ sodium bicarbonate plus $\pu{0.05 M}$ $\ce{NaCl}$. Ignoring ionic strength effects, the $\ce{NaCl}$ does nothing to the $\mathrm{pH}$.

The next one shows that $\mathrm{pH} = 8.339$ at the first equivalence point, for the neutralization of carbonic acid with $\ce{NaOH}$:

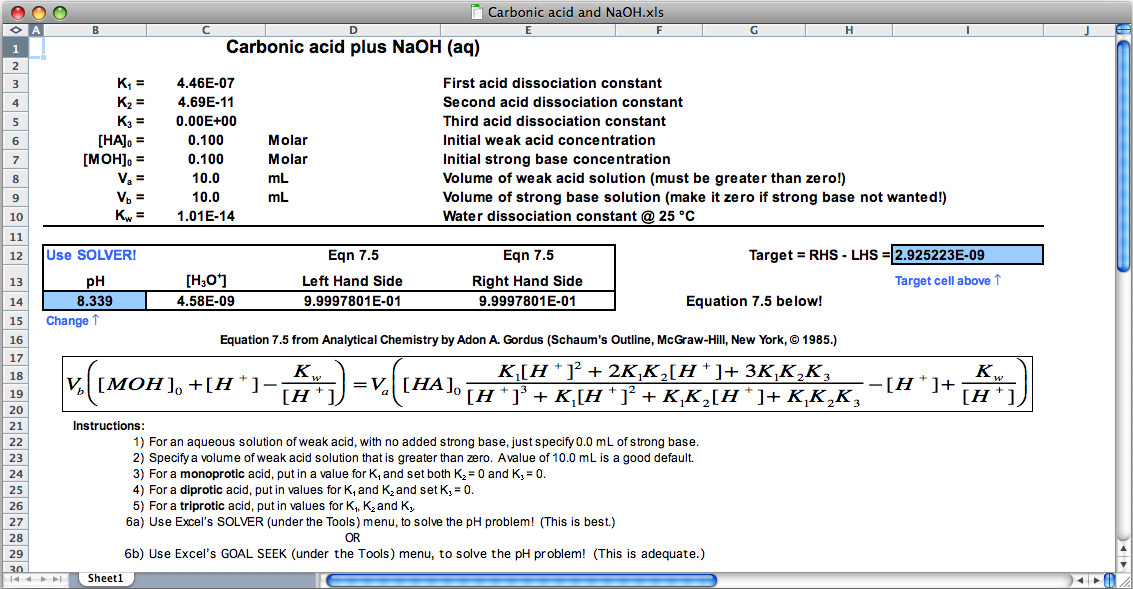
At the first equivalence point, the solution is $\pu{0.05 M}$ sodium bicarbonate. In both cases, the $\mathrm{pH}$ is approximately 8.34 and is consistent with this answer.

The two $\mathrm{p}K_\mathrm{a}$ values for carbonic acid are from D.G. Harris, In Quantitative Chemical Analysis, 7th Edition; Appendix G; W. H. Freeman and Company, ©2007. The two $\mathrm{p}K_\mathrm{b}$ values for carbonate ion are obtained from the two $\mathrm{p}K_\mathrm{a}$ values for carbonic acid via $\left(\mathrm{p}K_\mathrm{b1} = 14.00 - \mathrm{p}K_\mathrm{a2}\right)$ and $\left(\mathrm{p}K_\mathrm{b2} = 14.00 - \mathrm{p}K_\mathrm{a1}\right)$.

Answer 2

The (equivalence)points, which you have marked in the graphs, are where all of the acid present in the sample has been neutralized by the base added with the titrant –and vice versa.

But, pKa equals pH at the point where only half of the acid is neutralized. In other words: [HA] = [A-] -the concentration of acid equals the concentration of the corresponding base.

At these points, according to the Henderson-Hasselebalch equation, pH = pKa. They are called inflection points, and is where the titration curve is horizontal. See e.g. Wikipedia – titration curves