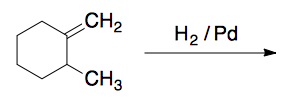The question is to predict the nature of the product(s) of the above reaction, that is if the product(s) form a racemic mixture, meso, diasteromers, or are structural isomers.
I am aware of the fact that addition of $\ce{H2}$ is a syn addition. So it should form a meso compound. However the answer key gives it as a mixture of diastereomers. I am not quite sure if the reaction is strictly selective and some anti products are also formed.
To answer and understand this, let’s first consider the products of the hydrogenation ($\ce{H2; Pd/C}$) of 1-methylidene-4-methylcyclohexane (the same reactant except the methyl group is in para-position). This compound is symmetric and therefore achiral. We can draw the methyl group pointing upwards iwthout loss of generalisation. The hydrogenation is syn-selective and it can occur from the top or the bottom. We expect a $1:1$ mixture of the former methylidene group pointing upwards (the syn-isomer) and pointing downwards (the anti-isomer). Both are achiral (the plane of symmetry is retained in the reaction) but not identical; therefore, they must be diastereomers.
Now let’s move the methyl group back into the 2-position. By moving the methyl group, the plane of symmetry is lost; it suddenly makes a difference whether the methyl group points upwards (S) or downwards (R). For each of the two isomers, we can again hydrogenate from both above and below; we can expect two anti and two syn isomers at first approximation: we enter one stereocentre into the reaction and we form an additional one making two stereocentres in total and thus 4 theoretically possible isomers.
In explicit forms, starting from the (2S) isomer the syn-product could be called (1R,2S) and the anti-isomer would be (1S,2S). From the (2R) isomer, another syn (1S,2R) and anti (1R,2R) isomer is expected. Only when taking a closer look at the products, it becomes obvious that the two syn isomers are in fact identical because they can be transformed into each other by rotation; and that they are achiral because they generated a new plane of symmetry. Thus, the syn isomer is a meso isomer.
Putting this together, the hydrogenation products are two enantiomeric anti products and a meso (syn) product. These two classes are diastereomers of each other, thus ‘a mixture of diastereomers’ is the only correct answers.
