The vibrational modes of $\ce{XeF2}$ are at $555$, $515$, and $213~\mathrm{cm^{-1}}$ but only two are IR active. Explain why this is consistent with $\ce{XeF2}$ having a linear structure.
From what I know, since it has $3$ atoms it should have $3n - 5$ vibrational modes, thus $3(3) - 5 = 4$ modes. Yet the problem tells me it only has $3$, which would suggest a non-linear shape. How to explain this?
Let's start with the rule of mutual exclusion, it will come in handy. It states that, for centrosymmetric molecules (molecules with a center of symmetry), vibrations that are IR active are Raman inactive, and vice versa.
A linear $\ce{AB2}$ molecule will have $(3n-5) = 4$ vibrational modes, but because it is linear 2 of the modes are degenerate. $\ce{CO2}$ is an example of a linear $\ce{AB2}$ molecule, look at the following diagram showing the 4 vibrations for $\ce{CO2}$ and notice that the bending vibration ($v_2$) is degenerate. That is, there is no difference between those 2 bending modes, they are identical.
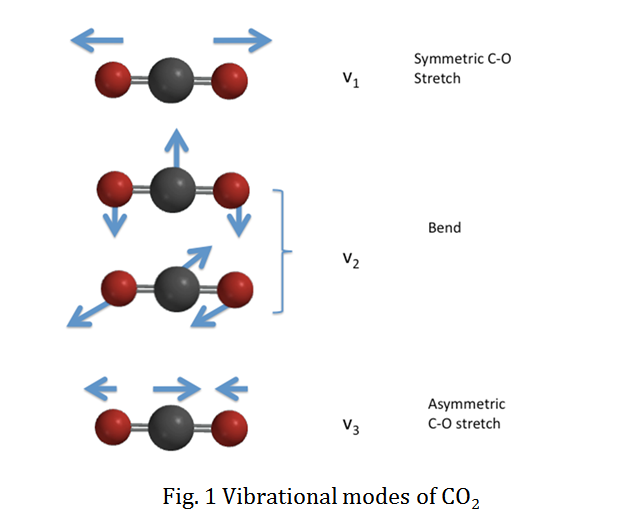
Notice too that $v_1$ is the only vibrational mode that does not change the dipole moment of the molecule, therefore it is the Raman active mode. Since the linear molecule is centrosymmetric, the Raman band does not appear in the IR, nor do any of the IR bands appear in the Raman spectrum. Hence, for a linear $\ce{AB2}$ molecule we would expect 2 IR active bands and 1 Raman band. This is consistent with the spectral data you reported.
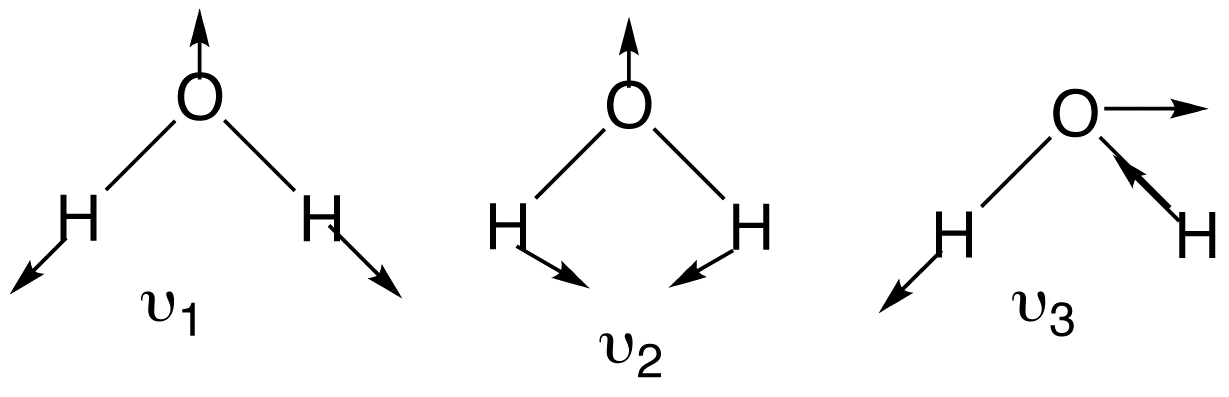
If the $\ce{AB2}$ molecule is bent rather than linear (water for example), then there are $(3n-6) = 3$ vibrational modes as pictured below. All of these vibrations result in a change in the dipole moment. Therefore, for a non-linear $\ce{AB2}$ molecule, all 3 of the bands will be IR active. Since the molecule is not centrosymmetric all 3 bands may also appear in the Raman spectrum (note: while a change in dipole moment is required for an IR-active band to appear, a change in polarizability is required for a Raman band to appear; looking at the water vibrations pictured, they should alter both the dipole moment and the polarizability of the molecule). Hence, for a non-linear $\ce{AB2}$ molecule we would expect 3 bands in the IR and 3 in the Raman spectrum. This is not consistent with the spectral data you reported above.
In summary, your spectral data is consistent with $\ce{XeF2}$ having a linear geometry.
Note: Thanks to @porphyrin for pointing out that bent $\ce{AB2}$ molecules are indeed not centrosymmetric.
The most reliable way to do this is to use point group tables.
A non-linear molecule such as type $\ce{AB2}$ e.g. water has $C_\mathrm{2v}$ symmetry. (The normal modes are given by symmetry species $\mathrm{a}_1$ ($v_1$ & $v_2$) and $v_3$, $\mathrm{b}_2$.) From the point group, IR transitions only belonging to $\mathrm{A}_1$, $\mathrm{B}_1$ and $\mathrm{B}_2$ symmetry species occur. (These are symmetry species in same rows as $x, y, z$ in the third main column of the point group table)
Both $\mathrm{A}_1$ and $\mathrm{B}_2$ are also Raman active as is $\mathrm{B}_1$. (The Raman active species are the squared terms in column 4). The symmetry species $\mathrm{A}_2$ is also Raman active but is not present as a normal vibrational mode. Thus all symmetry species are Raman active in $C_\mathrm{2v}$. Thus as only one species is Raman active in your example, the molecule is not bent so must be linear.
As a check any molecule with a centre of inversion (such as linear $\ce{FXeF}$) has no common transition in the IR and Raman spectrum. All the frequencies are unique so there is no overlap, and the molecule is linear. In fact this is all you need to know to determine that it is linear.
