9
$\begingroup$

In order for a molecule to be Raman active, there must be a change in the polarizability, meaning that there must be change in the size, shape or orientation of the electron cloud that surrounds the molecule. And this change said to occur in symmetric stretching, but not asymmetric stretching.

Please explain using plain english (without any rules or tables) why is that in asymmetric stretching like the following, there is no change in the size, shape or orientation of the electron cloud that surrounds the molecule? Specially considering each side independent has its own electron cloud.

enter image description here

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ Is possible the polarizability change requirement applies not to particular bonds, but to the whole molecule, with the opposite bond effects nullifying each other ? $\endgroup$
    – Poutnik
    Commented Dec 9, 2020 at 8:24
  • 3
    $\begingroup$ The effect on both sides levels out, mostly. Remember that some selection rules are stronger than others: Spin must be retained, and if you just have no dipole moment, you get no IR interaction. Oh, remember you have to look at this from the view of an electromagnetic wavelenght that is a thousand times as large. $\endgroup$
    – Karl
    Commented Dec 9, 2020 at 8:27
  • 1
    $\begingroup$ In the asymmetric stretch the molecule retains the same shape because as one extends the other contracts and vice versa. $\endgroup$
    – porphyrin
    Commented Dec 9, 2020 at 16:38
  • $\begingroup$ the shape oscillates in left and right, how can they be same shape? And why would same shape not cause change in polarizability, hope someone can Answer. $\endgroup$
    – Jtl
    Commented Dec 10, 2020 at 5:58
  • 3
    $\begingroup$ @Karl You don't need a dipole moment, you just need a change in the dipole moment. $\endgroup$
    – ron
    Commented Jan 9, 2021 at 23:03

1 Answer 1

Reset to default
4
+50
$\begingroup$

Please explain using plain english (without any rules or tables) why is that in asymmetric stretching like the following, there is no change in the size, shape or orientation of the electron cloud that surrounds the molecule?

There is a change. The size and shape of the electron cloud does change for the individual bonds affected by the asymmetric stretch. Polarizability relates to the ease with which electrons can be moved from their equilibrium position. Electrons in weaker (longer) bonds are more easily displaced than electrons in stronger (shorter) bonds.

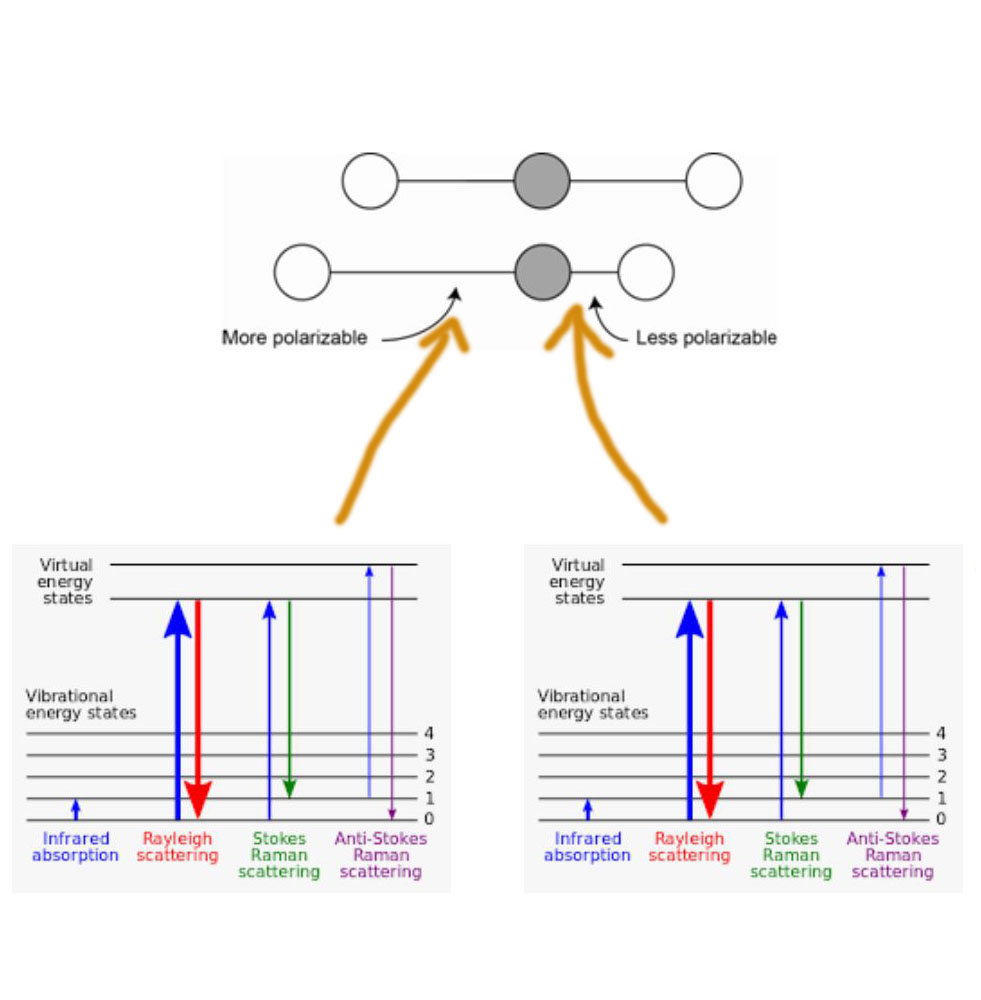
From your diagram, I believe that you are discussing the case of a linear $\ce{AB2}$ molecule. Picture the asymmetric stretch in our $\ce{AB2}$ molecule with the two $\ce{B}$ atoms remaining stationary and the $\ce{A}$ atom oscillating back and forth. Simultaneously one $\ce{A-B}$ bond becomes longer and the other shorter. One becomes more polarizable, the other less polarizable. In the linear $\ce{AB2}$case these changes in polarizability are equal and opposite, hence for the molecule overall they cancel out and there is no change in overall polarizability (for example, see here).

In order for a molecule to be Raman active, there must be a change in the polarizability

Yes, but it must be a change in polarizability for the entire molecule, not just an individual bond. Further, keep in mind the Principle of Mutual Exclusion. It states that, for centrosymmetric molecules (molecules with a center of symmetry, your linear $\ce{AB2}$ is an example), vibrations that are IR active are Raman inactive, and vice versa.

The asymmetric stretch in such a molecule changes the dipole moment in the molecule from zero to non-zero; hence the asymmetric stretch is IR active. Therefor by the Principle of Mutual Exclusion, the asymmetric stretch must be Raman inactive. Even though the asymmetric stretch changes the polarizabilty of some bonds in the molecule, the polarizability of the whole molecule does not change (as explained above) and the asymmetric stretch is Raman inactive as predicted.

The links I posted in the comments show how these concepts can be applied in the linear $\ce{AB2}$ examples of $\ce{CO2}$ and $\ce{XeF2}$.

Improve this answer
$\endgroup$
3
  • $\begingroup$ You just stated the rule about Principle of Mutual Exclusion which you quoted " It states that, for centrosymmetric molecules (molecules with a center of symmetry, your linear AB2 is an example), vibrations that are IR active are Raman inactive, and vice versa." But you didnt state the technical reasons why. Specifically, considering each side independent has its own electron cloud. So why is the polarizability in each side cancelled just because the molecules is bouncing back and forth in for example asymmetric stretching. $\endgroup$
    – Jtl
    Commented Jan 11, 2021 at 3:20
  • $\begingroup$ @Jtl Edited the answer to better address your points. $\endgroup$
    – ron
    Commented Jan 11, 2021 at 20:02
  • $\begingroup$ @Jtl, I think one should not imagine the polarizability as simply as you are imagining. Unfortunately, polarizability is a tensor. I don't know the answer to your query but I am trying to show polarizability is very hard picturize. See this physics query physics.stackexchange.com/questions/274794/…. $\endgroup$
    – ACR
    Commented Jan 12, 2021 at 3:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.