The space group $P4_222$ has some inherent symmetry on special projections beyond its eight symmetry equivalents. The International Tables for Crystallography, Volume A (DOI: 10.1107/97809553602060000114) notes that planes normal to each of the three projections for tetragonal space groups, namely $[100]$, $[001]$ and $[110]$, having their own symmetry in fact. Here's the relevant section for $P4_222$ from the International Tables:

It looks like you are referring to the planes associated with the projection $[110]$. The planes normal to [110] have symmetry $p2mm$ of their own as well as their own origin at $x,x,\frac{1}{4}$, although only one of these planes has its origin at $0,0,\frac{1}{4}$, and of course none of these planes has its origin at $0,0,0$. Here is how the collection of origins at $x,x,\frac{1}{4}$ looks like:
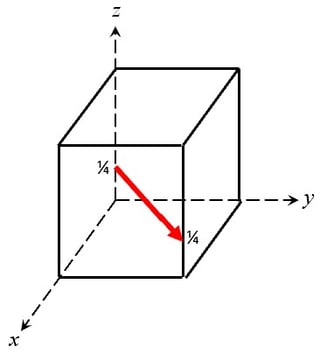
Lets take an example of one such plane, say $(110)$ normal to the projection $[110]$. Defining that plane is $x+y=1$. Here is how this plane looks like:
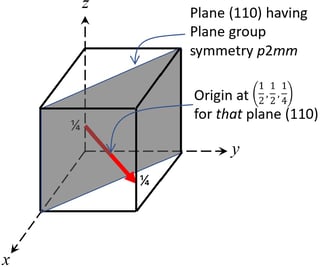
Applying the relationship $y=1-x$ to the $P4_222$ symmetry equivalents, the planar symmetry $p2mm$ reveals itself in the following:
$$(x,\bar x,\tfrac{1}{4}), (\bar x,x,\tfrac{1}{4}), (x,x,\tfrac{3}{4}), (\bar x,\bar x,\tfrac{3}{4}),$$ $$(\bar x,\bar x,\tfrac{3}{4}), (x,x,\tfrac{3}{4}), (\bar x,x,\tfrac{1}{4}), (x,\bar x,\tfrac{1}{4})$$
For this one plane $(110)$ the origin at $(\tfrac{1}{2},\tfrac{1}{2},\tfrac{1}{4})$.
The analysis may be generalized for the rest of the infinite collection of planes normal to the projection $[110]$, each having its origin at $x,x,\tfrac{1}{4}$ as noted above.
As for the origin for the space group $P4_222$ it is at $0,0,0$.



