How would a pH curve look like for titration of diluted weak base compared to concentrated one?

Question

Let's say I take $\pu{80 g}$ of a weak base, dilute it with $\pu{50 ml}$ of water and titrate it with a strong acid. I get a titration curve. Now I take again $\pu{80 g}$ of the same weak base but this time I dilute it with $\pu{200 ml}$ of water and titrate it with the strong acid. What will be the new titration curve compared to the first one?

And here is what confuses me:

On one hand, the diluted base should have lower $\mathrm{pH}$. But on the other hand, at half-equivalence point, $K_\mathrm{b}=\mathrm{pOH}$, and since it's the same base, $K_\mathrm{b}$ is the same, and thus $\mathrm{pOH}$ and $\mathrm{pH}$ at half equivalence point should be the same. I am also not sure about the endpoint.

Answer 1

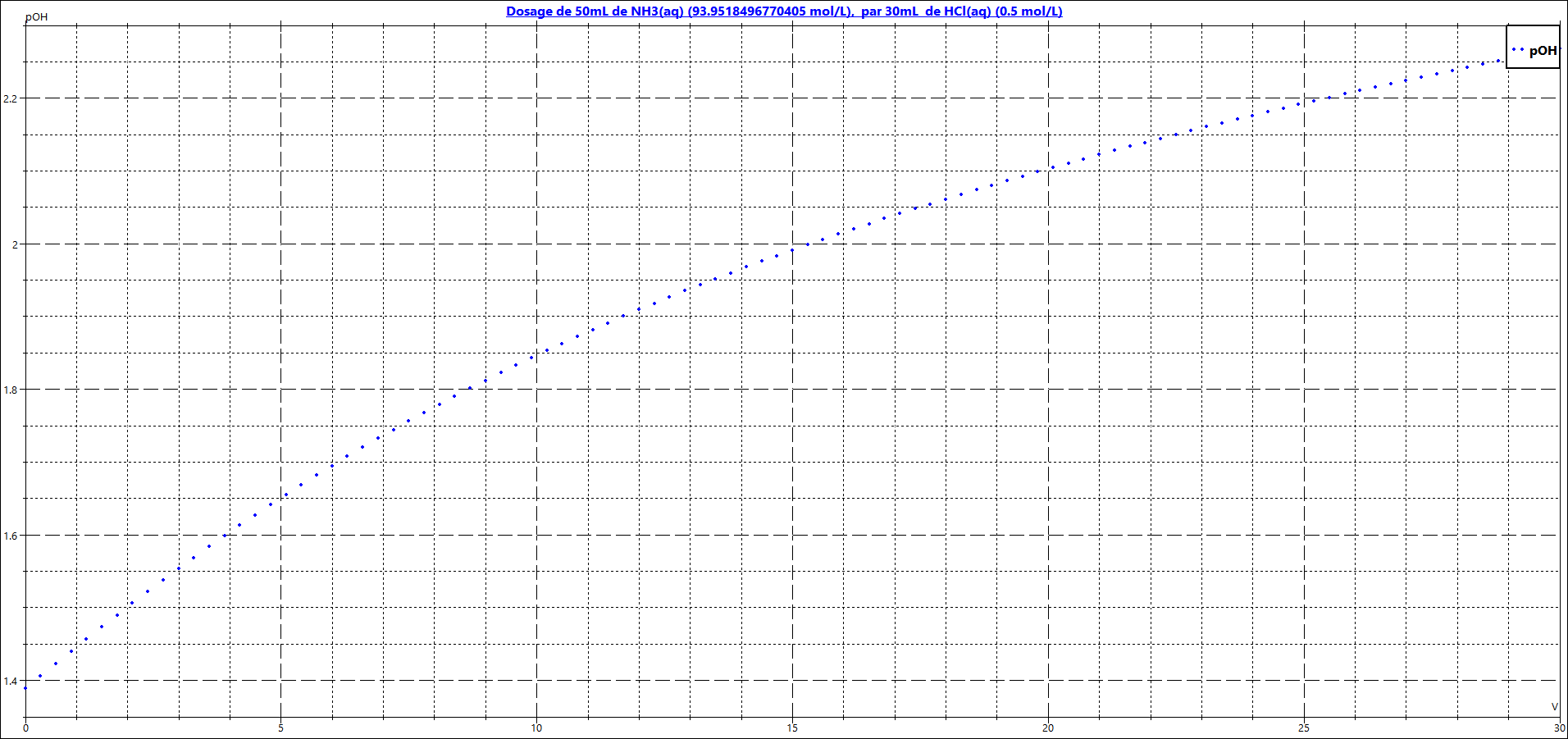
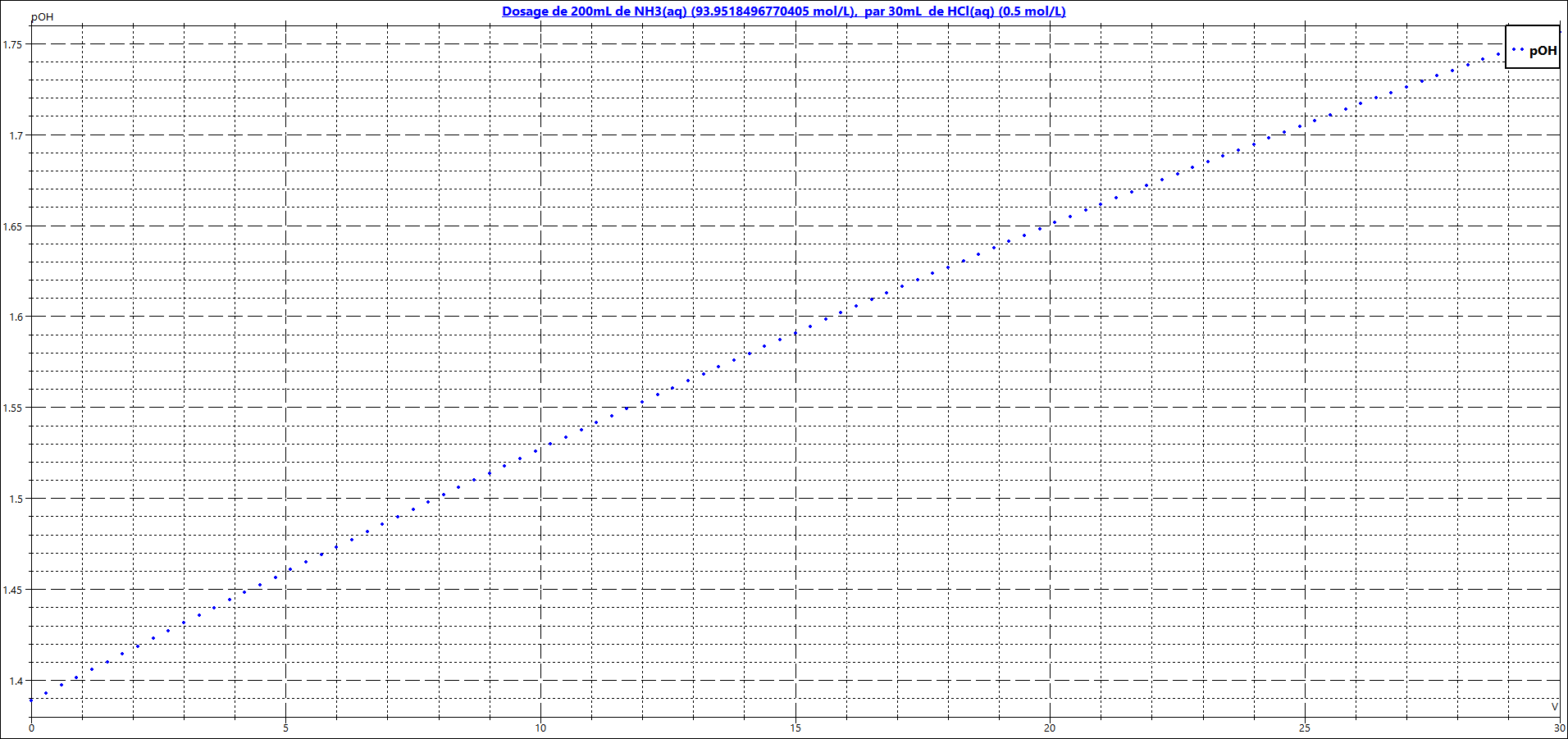
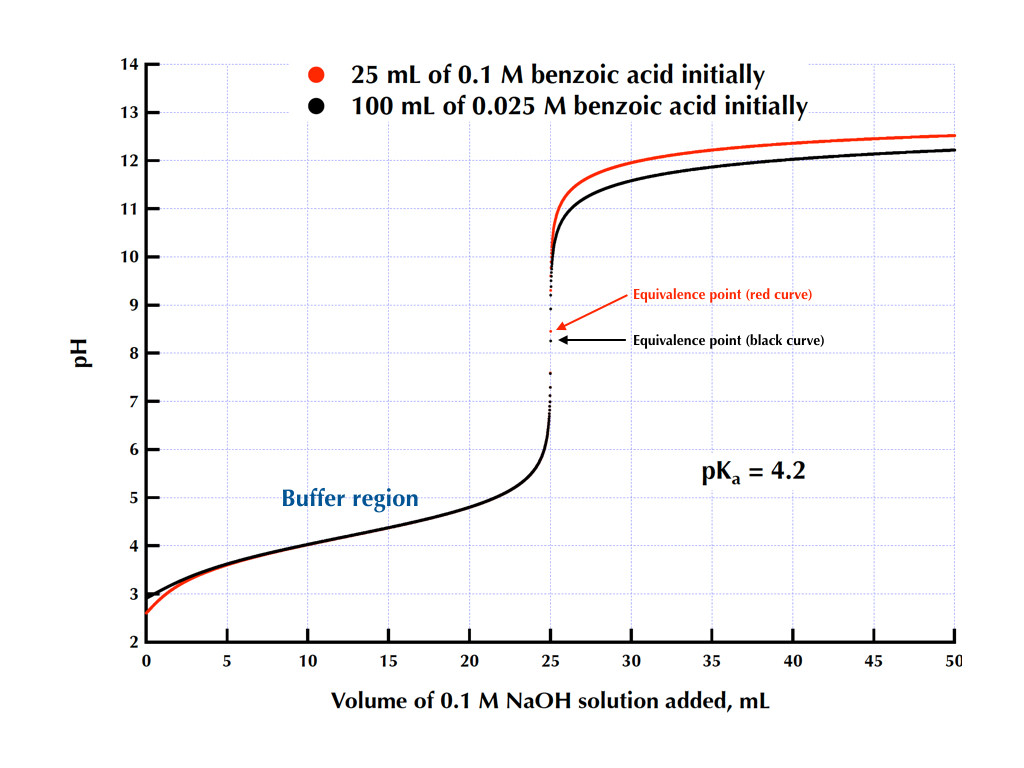
I am first going to do the ‘flip side’ of the OP’s scenario and then basically flip it back. So we start with a $\pu{0.1 M}$ aqueous solution of benzoic acid and the initial solution volume is $\pu{25 mL}$. Benzoic acid is a monoprotic weak acid with $\mathrm{p}K_\mathrm{a} = 4.20$. The initial $\mathrm{pH}$ is $2.606$. The strong base used for the titration is $\pu{0.1 M} \ \ce{NaOH}$. At the equivalence point, the $\mathrm{pH = 8.454}$ and the solution volume is $\pu{50 mL}$ ($=\pu{25 mL}$ benzoic acid solution $+ \pu{25 mL} \ \ce{NaOH}$ solution). Thus, the $\mathrm{pH}$ is simply the $\mathrm{pH}$ of $\pu{0.05 M}$ sodium benzoate solution. After all $\pu{50 mL}$ of $\ce{NaOH}$ has been added, the $\mathrm{pH} = 12.523$ and the final solution volume is $\pu{75 mL}$. At the half-equivalence point, $\pu{12.5 mL}$ of $\ce{NaOH}$ has been added and $\mathrm{pH}= 4.185$.

Now assume the initial benzoic acid solution is only $\pu{0.025 M}$, but the initial volume is $\pu{100 mL}$. The total number of moles of benzoic acid is the same as before. The initial $\mathrm{pH}$ is $2.912$: the benzoic acid solution is only 25% as concentrated. At the equivalence point, the $\mathrm{pH} = 8.254$ and the solution volume is $\pu{125 mL}$ ($=\pu{100 mL}$ benzoic acid solution $+ \pu{25 mL} \ \ce{NaOH}$ solution). The equivalence point $\mathrm{pH}$ is closer to $\mathrm{pH}=7$ because the solution is simply a $\pu{0.02 M}$ sodium benzoate solution, i.e., the number of moles of sodium benzoate is now in a volume that is 2.5 times larger, so the concentration is 40% of $\pu{0.05 M}$. After all $\pu{50 mL}$ of $\ce{NaOH}$ has been added, the $\mathrm{pH}= 12.222$ and the final solution volume is $\pu{150 mL}$. At the half-equivalence point, $\pu{12.5 mL}$ of $\ce{NaOH}$ has been added and $\mathrm{pH} = 4.188$, moving slightly up toward $\mathrm{pH}=7$. See the titration curves below:

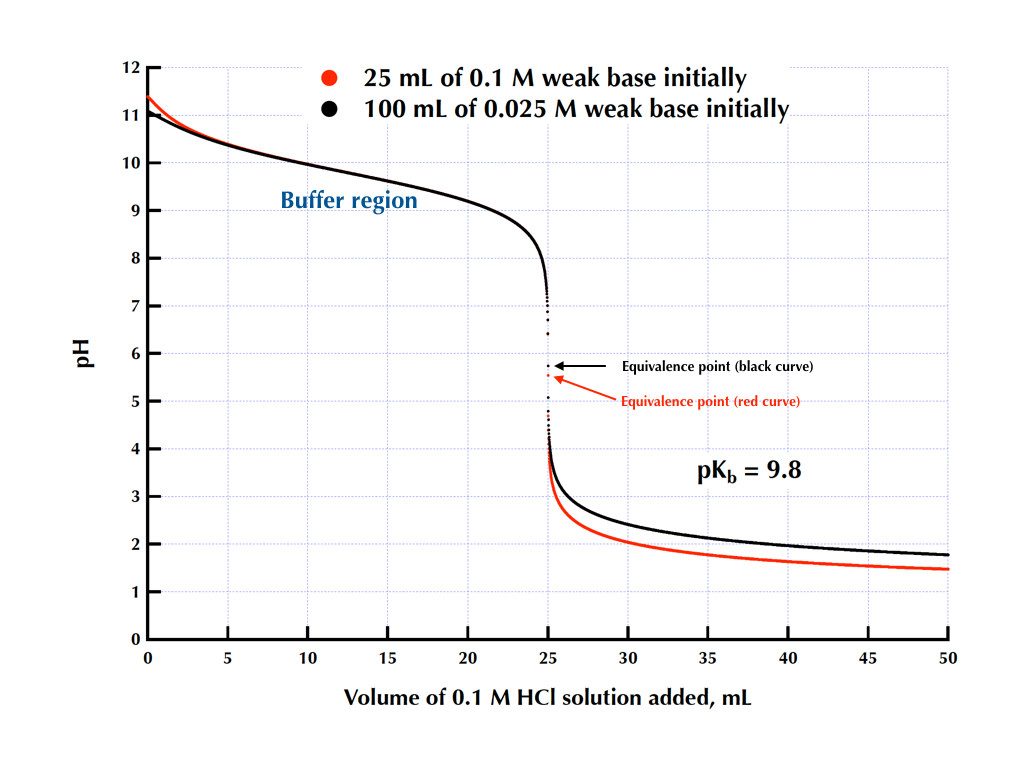
Now flipping back: the OP’s scenario involving a weak base titrated with strong acid. For reasons that will become clear, assume the hypothetical weak base has $\mathrm{p}K_\mathrm{b} = 9.80$, and that the strong acid is $\pu{0.1 M} \ \ce{HCl}$ solution. Note that the $\mathrm{p}K_\mathrm{b}$ is simply $14$ (treated as exact throughout) $- 4.20$.

As before, start by assuming $\pu{25 mL}$ of $\pu{0.1 M}$ weak base. The initial $\mathrm{pH} = 14 – 2.606 = 11.394$. At the equivalence point, the $\mathrm{pH} = 5.546$ and the solution volume is $\pu{50 mL}$ ($=\pu{25 mL}$ weak base solution $+ \pu{25 mL} \ \ce{HCl}$ solution). Thus, the $\mathrm{pH}$ is simply the $\mathrm{pH}$ of a $\pu{0.05 M}$ weak base salt solution. After all $\pu{50 mL}$ of $\ce{HCl}$ has been added, the $\mathrm{pH} = 1.477$ and the final solution volume is $\pu{75 mL}$. At the half-equivalence point, $\pu{12.5 mL}$ of $\ce{HCl}$ has been added and $\mathrm{pH} = 9.815$.

Now assume the initial weak base solution is only $\pu{0.025 M}$, but the initial volume is $\pu{100 mL}$. The total number of moles of weak base is the same as before. The initial $\mathrm{pH}$ is $11.088$: the weak base solution is only 25% as concentrated. At the equivalence point, the $\mathrm{pH} = 5.746$ and the solution volume is $\pu{125 mL}$ ($=\pu{100 mL}$ weak base solution $+ \pu{25 mL} \ \ce{HCl}$ solution). The equivalence point $\mathrm{pH}$ is closer to $\mathrm{pH}= 7$ because the solution is simply $\pu{0.02 M}$ weak base salt solution, i.e., the number of moles of weak base salt is now in a volume that is 2.5 times larger, so the concentration is 40% of $\pu{0.05 M}$. After all $\pu{50 mL}$ of HCl has been added, the $\mathrm{pH} = 1.778$ and the final solution volume is $\pu{150 mL}$. At the half-equivalence point, $\pu{12.5 mL}$ of $\ce{HCl}$ has been added and $\mathrm{pH} = 9.812$, moving slightly down toward $\mathrm{pH}= 7$. For the titration curves, simply imagine vertically flipping the titration curves, in the figure above, about a horizontal line at $\mathrm{pH} = 7$. Or just look at the plot:

One last thing: The $\mathrm{pH}$ values at half-equivalences were close to the respective $\mathrm{p}K$ values, as expected, but any buffer can be broken by dumping it into swimming pool full of water. So dilution may matter a great deal, or relatively little, depending on the specifics.

Answer 2

The equivalence is, by definition, the moment when $n_{base}=n_{acid}$. As you titrate the same mass of base (consequently the same quantity), your equivalence will be reached at the same point. The only difference, to me, between the two curves will be the fact that you will less see the equivalence when the solution is diluted. First curve is 80g in 50mL, second one is 80g in 200mL.

Answer 3

At the beginning of the titration, the solution is a weak base. At the equivalence point, the solution is a weak acid (with some more spectator ions). The pH of these solutions changes with dilution, both in the direction of neutral pH.

In contrast, at the half-equivalence point (or midpoint) of the titration, the solution is a 1:1 buffer. As long as the buffer concentration is large compared to the concentration of hydronium and hydroxide ions, the pH will be close to the pKa (and the pOH close to the pKb), irrespective of dilution.

So if you dilute the weak base with water before the titration, nothing will change along the x-axis (i.e. half-equivalence point and equivalence point will occur at the same volume of added strong acid). Along the y-axis (where you plot the pH), the curve will "shrink" toward the pH of the buffer (i.e. the starting pH will be lower - less basic - and the pH at the equivalence point will be higher - less acidic). Past the equivalence point, the pH is controlled by the excess of strong acid. Because the strong acid is diluted into a greater volume if you add water to the weak base before starting the titration, this part of the titration curve will also show higher - less acidic - pH values.