Taking the example of octahedral complexes, the theory says that since the $d_{z^2}$ and $d_{x^2-y^2}$ orbitals are closer to the ligands than the $d_{xy}$ ,$d_{yz}$ and $d_{xz}$ orbitals, they reach a higher energy level than the $d_{xy}$ ,$d_{yz}$ and $d_{xz}$ orbitals.(due to greater interaction with the ligands)
I can't understand why the $d_{z^2}$ and $d_{x^2-y^2}$ orbitals are closer to the ligands. I agree that they are along the bonding axes, but with some rotation in space I can have other d-orbitals along the bonding axes. For example, if I rotated the octahedral arrangement of ligands about the z-axis, then the $d_{xy}$ orbital could be along the bonding axis instead of the $d_{x^2-y^2}$ orbital.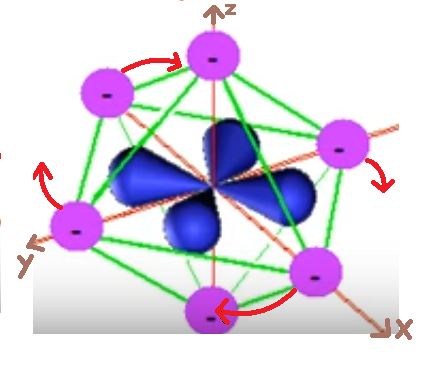
So why can't other combination of d-orbitals (like the $d_{xy}$ and $d_{z^2}$ ) reach a higher energy level instead of the $d_{z^2}$ and $d_{x^2-y^2}$ during splitiing?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ You choose the x y z however you want. If the x y z is chosen as the direction of the ligands, then you have the regular splitting scheme. If you choose different names, differently orbitals will be the high and low energy ones. This is only a naming problem $\endgroup$– GregCommented May 6, 2017 at 9:59
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
According to this theory ligands are considered point charges. These points acquire positions as those you've presented above. This leads to some d orbitals being closer to the ligands. That means that a higher amount of energy is needed for an eletron to be placed there, because it would be closer to the negative charged ligand.
However, which orbital is going to be more affected, is a matter of an "agreement" you may say. In order to be easier to explain situations, structures etc it is inclined to use a conformation such as the one you've shown. It would be much more difficult to discuss matters if everyone considered different naming and configurations.
answered May 6, 2017 at 12:41