When electrons gain energy they become more excited and move to a higher energy level, which increases the tendency of an atom to form a bond with another atom. Thus, surely bond making requires the absorbance of energy?
Equally when electrons lose energy they drop to a lower energy level, which would break a bond. Therefore, why isn't bond breaking exothermic?
Could someone point out the flaw in my reasoning, because I don't get why bond-breaking is possibly endothermic?
When electrons gain energy they become more excited and move to a higher energy level, which increases the tendency of an atom to form a bond with another atom.
Let me assure you that this statement is incorrect. I’m not fully sure where you extracted it from, but I assume it stems from the way many schools teach hybridisation at the beginning of organic chemistry classes; requiring an $\ce{s\bond{->}p}$ excitation in carbon from $\mathrm{[He]\ 2s^2\,2p^2}$ to $\mathrm{[He]\ 2s^1\,2p^3}$, after which the s- and p-orbitals can form $\mathrm{sp^3}$ hybrid orbitals. This idea is nothing more than a school level simplification used to get around teaching of more complex molecular orbital theory and symmetry.
Nothing prevents you from constructing e.g. a methane molecule without initial hybridisation, i.e. starting from an unhybridised carbon atom and four hydrogen atoms in a tetrahedric arrangement. I refer you to the following scheme posted in a different question and originally taken from Professor Klüfers’ internet scriptum for basic and inorganic chemistry at the university of Munich:
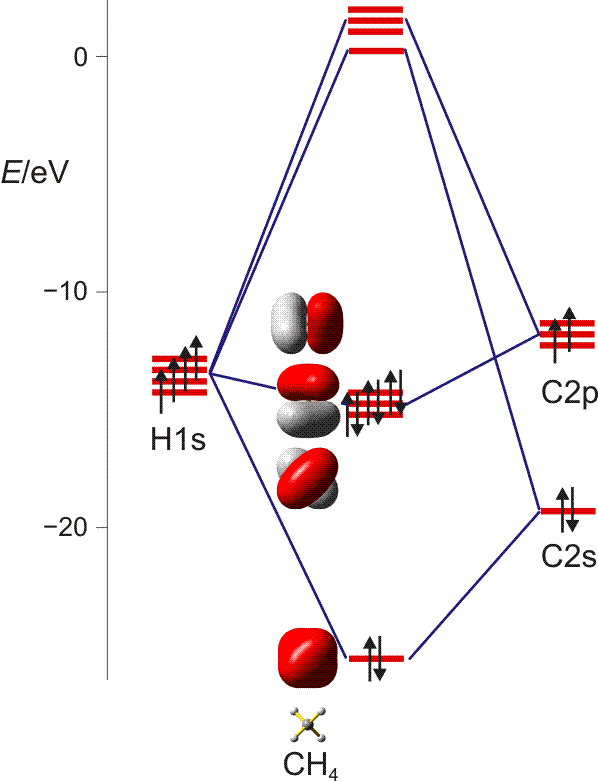
As you can see on the right, carbon enters this scheme in the unhybridised ground state. There is no need to invoke a previous hybridisation before mixing orbitals; rather, it is necessary to determine the symmetry of orbitals and thereafter combine symmetry-equivalent orbitals in a bonding-antibonding fashion. Finally, fill in electrons from bottom to top.
This method will always result in stabilisation of bonding orbitals; the trade-off always being the destabilisation of antibonding orbitals in such a way that the (actual) energy gained is lower than the (virtual) energy lost.
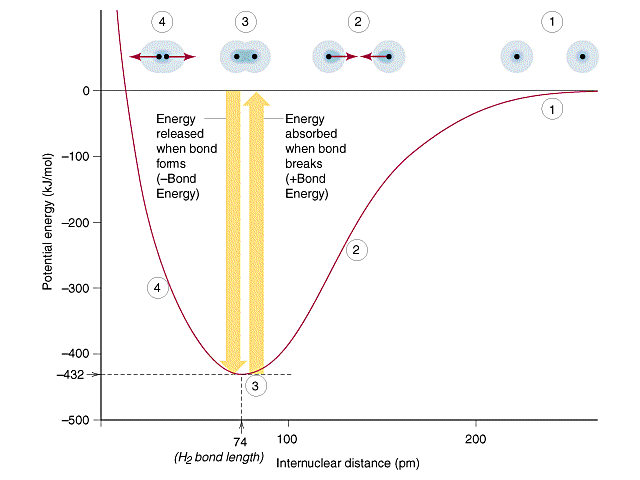
Therefore, assuming a positive bond order, forming a bond typically liberates energy while breaking one will typically require energy. I am not aware of any counterexamples, but the sentence is phrased so that it remains true when the obligatory counterexample is posted as a comment.
Sorry, your logic doesn't quite hold. Increasing the energy of electrons does make it more likely that they will do something, but the end result is frequently something that is lower in energy than the starting state.
Dropping in energy levels doesn't break a bond. Generally, bonds correspond to the lowest energy levels.
The main thing to keep in mind is that one way to define a bond is that it is the stabilization of electrons between a group of atoms. For neutral species, the stabilization is relative to the energies of the electrons in the atomic orbitals of the constituent atoms.
No matter how weak the bond is, there are always going to be some interactions between the two species involved in the bond. It is because of those interactions that the bonds were formed at the first place so energy will always be required to break those interactions and hence bond cleavage is always endothermic.
You seem to have it the wrong way round. Adding energy to an electron in a bond places it into an anti-bonding orbital making it more likely that the bond will break. Adding a second amount of energy to put two electrons into anti-bonding orbitals even more so. The normal bonded state is the lowest energy state by convention this is the most negative energy. When a bond is formed energy is released and is normally taken up by the surrounding molecules and translational, vibrational and rotational energy.
To understand this, first you have to know that energy of a system is always inversely proportional to the stability of system.
When two atoms come towards each other, the energy of the system of two atoms decreases ( Here the energy is potential energy). When atoms form bond, this energy becomes minimum ( since the system of atoms is most stable now). Notice that energy has become minimum so there must be some lost of energy, and this is the energy which is released when a bond is formed.
Now if you want to to break that bond , you have to seprate those atoms ( separating atoms means decreasing the stability of two atom system) and since stability is inversaly proportional to energy hence decreasing the stability is equivalent to increasing the energy. So when you are breaking a bond, you are seprating the atoms and this will lead to an increase in energy. An increase in energy of system is only possible if energy is provided to system. I shall let you conclude now.
You have to break the mutual electrostatic attraction between the electrons and protons of each atom.
A bond between two atoms happens because they get a more stabilized stadium of energy. In chemistry, low potential energy means more stabilization. Think to a ball at the bottom of a basin. This is an extremely stabilized system, and means you will have to put energy to move this ball. Conversely, a ball on top of a potential energy hill is not a stable system, and no energy is required move the ball down.
A bond between two atoms is the ball at the bottom of a basin - energetically speaking.
