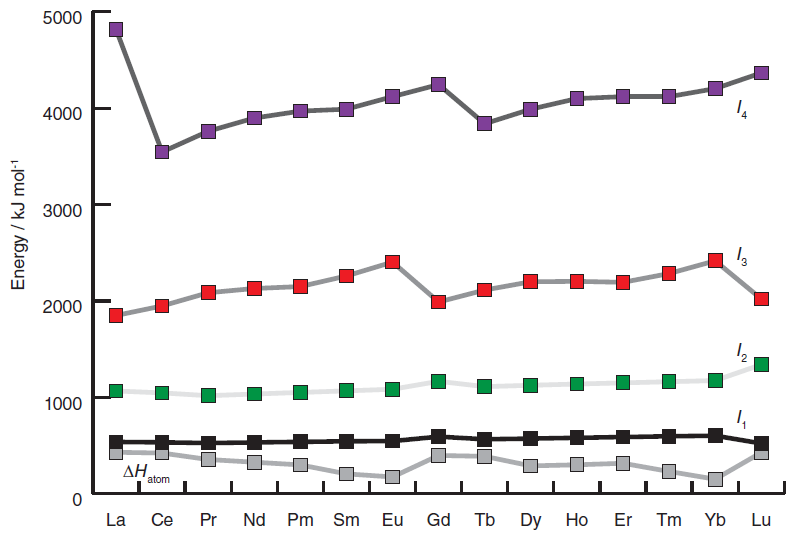
For most lanthanide metals$^{[1]}$, the stable oxidation state is III. The general electronic structure$^{[2]}$ is $$\ce{[Xe] 4f^{0}^{-14} 5s^2 5p^6 5d^{0}^{-1} 6s^2}.$$
Elements that have the d-electron are La, Ce, Gd, and Lu. Furthermore, the f-subshell is considered relatively stable in states $$f^0, f^7, \text{and} f^{14}.$$
We can conclude that La, Gd, and also Lu easily form $\ce{E^3+}$ ions. Yet, as would be predicted by this easy approach, we would also see $$\ce{Sm+, Tm+ (f^7 \ and \ f^14 ), \\ Pr^5+, Dy^5+ (f^0 \ and \ f^7)}.$$
Why is this not the case?
$^{[1]}$ Ce, Pr, and Tb also have the oxidation state IV. Eu and Tm have the additional state II.
$^{[2]}$ Ordering (relative energy) changes with the number of electrons.
I strongly recommend having a look at these questions:
- Howcome orbitals become 'core-like' when electrons are removed?
- What is meant by 'electrons of like/unlike rotation'?
- What's up with this quarter / three-quarter rule?
Original topic: Predominance of III oxidation state for lanthanides