I know that activation energy for a reaction is the extra energy given to the reactants to reach the threshold energy so that they can collide and react. But then, why is it said that the activation energy does not depend on the temperature?
If the temperature is higher, the reactant molecules have a higher kinetic energy, and thus the energy difference between the threshold energy and the energy of the molecules will decrease, that is, the activation energy will decrease.
There is a simple (some might say simplistic) way to get an intuition about this and it involves thinking at the molecular or atomic level rather than about the bulk properties of the reaction (the thermodynamic view).
For a simple reaction where molecule A has to bang into molecule B to create molecule C, the reaction will only happen if the amount of energy involved in the collision is large enough. The amount of energy involved depends on the nature of the reaction which is determined by the electronic structures of A and B. Within limits, those electronic structures don't vary with temperature and a given reaction will only occur when there is enough energy in the collision for the reaction to happen.
What changes with temperature isn't the energy required for the reaction to occur, it is the number of molecules that have enough energy to overcome the barrier. At higher temperatures more molecules have that energy, but the amount of energy required doesn't change. The amount of energy require to initiate the reaction is roughly equivalent to the activation energy. It can be calculated by looking at the way the overall rate of the reaction changes with temperature. So the rate of reaction changes with temperature but not the barrier to the reaction happening.
This view is a little simplified but it gives the right intuition about the problem.
As mentioned above, it is best to first assume that activation energy is temperature independent. The number of molecules that have sufficient energy to do the reaction, can now be calculated via the Boltzmann distribution. The textbook "Physical Organic Photochemistry" from Anslyn & Dougherty has a very good picture to visualize this.
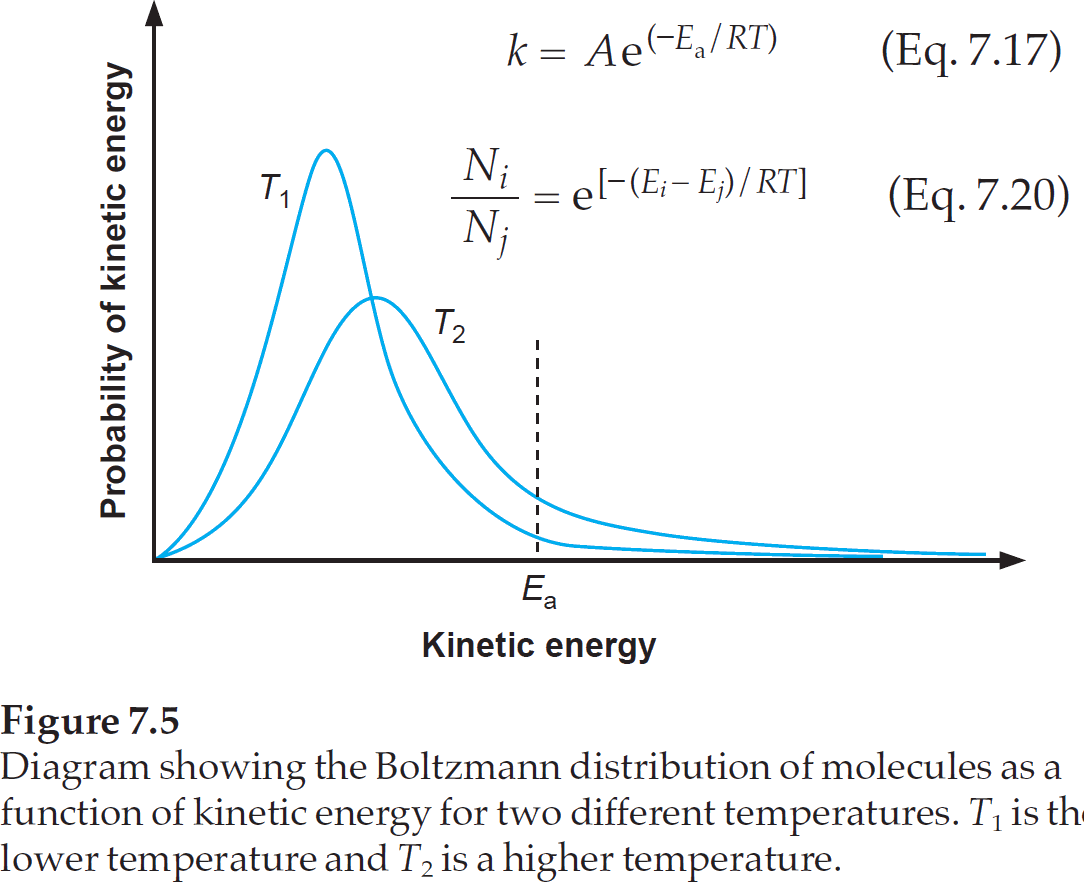
Note that Eq. 7.17 is the Arrhenius equation, and Eq. 7.20 is the Boltzmann distribution.
Another thing mentioned is the dependance of Activation energy $E_a$ on temperature $T$. This is best illustrated by Figure 7.6 of the Physical Organic Photochemistry-book; it shows that the reaction coordinate diagram (shown on the right) must actually be seen as an energy surface (shown on the left).
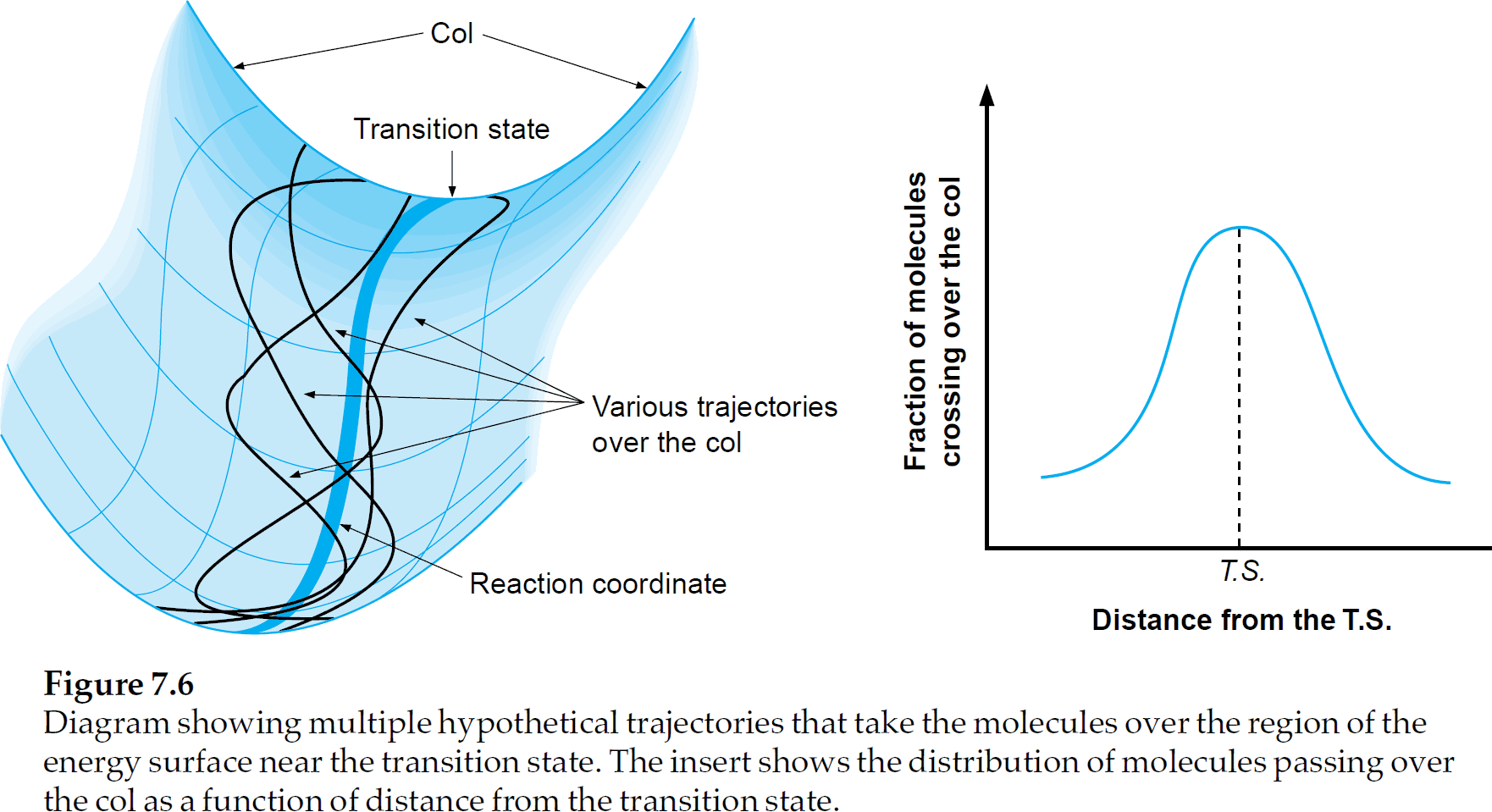
At higher temperatures, there is more vibrational/rotational/translational energy, in total there are 3N degrees of freedom. Only one of the vibrations will lead to an effective reaction; e.g. the bond of interest to be broken. This is why the length of that bond is chosen as a reaction coordinate.
Thermal energy, however, will be distributed over all degrees of freedom, according to the Maxwell–Boltzmann distributions. The point is that, at a higher temperature, there will be more paths over the transition state than just the path that walks in the valley of the energy surface.
Back to the question. Does activation energy depend on temperature? Well, the minimum activation energy, or enthalpy of activation ($\Delta H^\ddagger$) does not change, but the effective activation energy ($\langle E_a \rangle$, or actually simply $E_a$), which is essential for the macroscopic property of a rate constant, does increase, because paths other than the minimum require slightly higher activation energy.
According to page 368 of "Anslyn and Dougherty - Modern Physical Organic Chemistry", the effective activation energy ($E_a$) can be connected to enthalpy of activation ($\Delta H^\ddagger$) via an equation. For a single-step unimolecular or bimolecular reaction, the following equation holds:
$$ \text{Activation energy: } E_a = \Delta H^\ddagger + RT \qquad (eq. 1)$$
According to the internet, it may be generalized for single-step multimolecular reaction as follows:
$$ \text{Activation energy: } E_a = \Delta H^\ddagger + (1-\Delta n^\ddagger)RT \qquad (eq. 2)$$
where $\Delta n^\ddagger$ is the change in the number of molecules changed in going from the reactants to the transition state. For example, a bimolecular reaction in the gas phase would have $\Delta n^\ddagger = -1$. In most other cases, however, $\Delta n^\ddagger = 0$, so this equation becomes equal to the one given in the book of Anslyn and Dougherty.
Let's take a look at what the consequence of this $E_a$-temperature-dependence is. Let's plug it into the Arrhenius-equation!
Before I start, I want to make one final change to activation-ennergy-equation. For convenience, I define $n = (1-\Delta n^\ddagger)$, so that upcomming equations appear less scary. Note that $n$ is generally 1 for reactions in the liquid-phase, but $n$ may be 2 in the case of reactions in the gas-phase.
$$ \text{Activation energy: } E_a = \Delta H^\ddagger + nRT \qquad (eq. 3)$$
Below, I start with the original Arrhenius equation, and then substitute $E_a$ with eq. 3. I then try to simplify.
$$ \begin{align} \text{Arrhenius: } k_1 = \!\! \qquad A \!\! \qquad & e^{\frac{-E_a }{R T}}\\ \text{Arrhenius: } k_1 = \!\! \qquad A \!\! \qquad & e^{\frac{-(\Delta H^\ddagger + nRT)}{R T}}\\ ... = k_1 = \!\! \qquad A \!\! \qquad & e^{\frac{-nR T}{R T}} \times e^{\frac{-\Delta H^\ddagger}{R T}}\\ ... = k_1 = \!\! \qquad \frac{A}{e^n} \!\! \qquad & \times e^{\frac{-\Delta H^\ddagger}{R T}} \propto e^{\frac{-E_a }{R T}} \end{align}$$
Now, because $A$ is the "pre-exponential Arrhenius constant", I think we can make our life easier by including the $e^{-n}$ term in this constant. For as far as I know, changing $n$ would mean changing reaction mechanism, which is (I suppose) not so easy in practice, and generally not done in kinetics studies. Keeping this in mind, we may state the following:
$$k_1 = \frac{A_{old}}{e^n} \times e^{\frac{-\Delta H^\ddagger}{R T}} = A_{new} \times e^{\frac{-\Delta H^\ddagger}{R T}}$$
According to Arrhenius equation, we must conclude that temperature dependence of activation energy is irrelevant on the kinetics of the reaction, because it just changes the pre-exponential factor with a non-temperature-dependent constant.
If you try to determine the value of activation energy or reaction Gibbs energy, via kinetics, however, Arrhenius is far from ideal, because $E_a = - n R T \ln(\frac{k_1}{A})$, and if you go deeper on Wikipedia, you'll find out that the pre-exponential factor $A$ may also depend on temperature and other fudge factors.
I think that, if you want to determine information on the energy of a reaction, it is better to use the Eyring equation, because it has no fudge factors in it. I'll give it below, just for completeness, but I'll stop before I'll go off topic.
$$ \begin{align} \text{Arrhenius: } k_1 = \!\! \qquad A \!\! \qquad & e^{-\frac{E_a }{R T}}\\ \text{Arrhenius: } k_1 = \,\, \frac{k_\mathrm{B}T}{h} \,\, \mathrm{e}^{-{\frac {\Delta G^\ddagger}{RT}}} \end{align}$$
$$ k={\frac {k_{{\mathrm {B}}}T}{h}}{\mathrm {e}}^{{-{\frac {\Delta G^{\ddagger }}{RT}}}} $$
Activation energy ($E_\mathrm a$) is temperature independent, and activation free energy ($G_\mathrm a$) is temperature dependent. If we analyze Erying's transition state theory, or the statistical mechanical treatment of free energy it will become obvious. For a generic reaction
$$\ce{A + B <=> \{AB\}^\ddagger -> P}$$
we have
$$\begin{align} k_\mathrm{forward} &= \frac{k_\mathrm{B}T}{h}\cdot \frac{\widetilde{q}_{\ce{AB^\ddagger}}(T)}{q_\ce{A}(T)q_\ce{B}(T)}\cdot \exp\left(-\frac{E_\mathrm a}{k_\mathrm{B}T}\right) \\[6 pt] &= \frac{k_\mathrm{B}T}{h}\cdot\exp\left(-\frac{G_\mathrm a}{k_\mathrm{B}T}\right) \end{align}$$
So here we have two different contribution to free energy. One is entropic or temperature dependent contribution that comes from vibrational, rotational, and translational partition function, and temperature independent contribution from electronic partition function. Electronic contribution or activation energy is temperature independent (though all the electronic structure software use elevated temperature to find the electronic energy, but later they all extrapolate it to $\pu{0 K}$). Even zero point energy is also temperature independent. Electronic partition function is temperature independent as long as they are in ground state.
Activation energy has a term that is proportional to temperature according to transition state theory (at least). This relies on the definition of activation energy as the parameter
$$E_\mathrm a = RT^2\left(\frac{\mathrm d \ln k}{\mathrm dT}\right)$$
(see this answer).
For instance, for the bimolecular reaction $\ce{A + B -> P}$, we have $$E_\mathrm a = \Delta^{\ddagger}H^{\ominus} + nRT$$ where $\Delta^{\ddagger}H^{\ominus}$ is standard enthalpy of activation, and $n = 2$ for a gas-phase reaction and $1$ for a reaction in solution. That said, for many reactions, the activation enthalpy is much larger than $RT$ (which is ~$\pu{2.5 kJ/mol}$ at $\pu{300 K}$) and the activation energy can be approximately regarded as temperature independent.
