I understand Le Chatelier's principle and how every change to an equilibrium system causes an opposing reaction from the system. I also understand how, when pressure is increased, the equilibrium shifts to the side with the lowest number of moles of gas and vice versa.
So, why does this happen? How is it more energetically favourable - or is it something else?
Also, are these explanations of why the ration changes for the other factors right:
- Temperature - equilibrium moves to the endothermic side of the reaction as temperature increases because the activation enthalpy of the exothermic reaction increases as the overall energy of the system is less
- Concentration - ratio of products/reactants changes immediately after one part of the reaction's concentration increases, it then approaches the same ratio (assuming one whole side of the reaction is added)
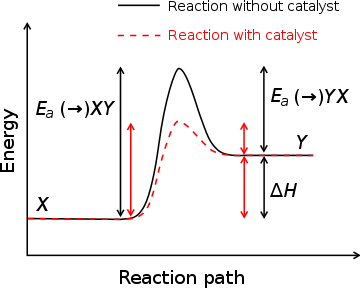
- Catalysts do nothing more than decrease the time it takes to reach equilibrium as they catalyse both sides of a reversible reaction and reduce the activation enthalpy