Is Hexafluorocyclohexane the "Most Polar" Small Molecule?

Question

Earlier this year, there was a lot of attention when all-cis 1,2,3,4,5,6-hexafluorocyclohexane was synthesized*:

Notably, C&E News quoted the lead author:

This compound is remarkable for being the most polar nonionic compound now known to exist

Now, the original paper reports a molecular dipole moment of $\pu{6.2 D}$, which is extremely high for an alkane. It also claims the:

unusual property of a facially polarized ring in organic chemistry

Certainly having one side of the cyclohexane ring completely substituted with fluorines is unusual, so I agree with the facial-polarity property being interesting.

But there are many small molecules (e.g., under 20-30 atoms) with large dipole moments, e.g., p-nitroaniline:

A quick PM7 calculation gives the predicted dipole moment of $\pu{7.93\pm1 D}$. So I dispute the claim from C&E News - nitroaniline is clearly more polar and non-ionic.

So my question is this: What's the largest dipole moment for a known non-ionic organic molecule under 30 atoms?

*: O'Hagan, et. al, "All-cis 1,2,3,4,5,6-hexafluorocyclohexane is a facially polarized cyclohexane" Nature Chemistry 7, 483–488 (2015)

Answer 1

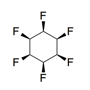
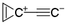At first, I thought, that those 6.2 D of O’Hagan et al. have been measured somehow but it as later described, they calculated it (1):

A molecular dipole value of 6.2 D for 1 was calculated at the M11/6-311G(2d,p) theory level using natural bond orbital (NBO) analysis with NBO 6.0.

I was using your given database for a little search, starting with nitroaniline, and came up with a few small molecules with higher dipole moments. Those seem to be PM7 calculated values and are shown in the following table:

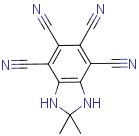
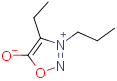
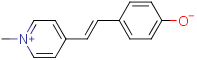
\begin{array}{lll} \hline \text{Name} & \text{Dipole moment in D} & \text{Source}\\ \hline \text{4-Nitroacetanilide} & 8.36 \pm 1.08 & (2)\\ \textit N\text,\textit N\text{-Dimethyl-4-nitroaniline} & 9.53 \pm 1.08 & (3)\\ \textit P\text ,\textit P\text{-Dimethyl-}\textit N\text{-(4-nitrophenyl)phosphinic amide} & 10.89 \pm 1.08 & (4)\\ \hline \end{array}

As there were some comments, “it seems that this level of calculation does not provide accurate estimates of dipole moments”, I did some further calculations on levels that might provide better estimates.

There is a quite new paper of Hickey and Rowley (5) which says:

CCSD, MP2, or hybrid DFT methods using the aug-cc-pVTZ basis set are all able to predict dipole moments with RMSD errors in the 0.12–0.13 D range and polarizabilities with RMSD errors in the 0.30–0.38 Å³ range.

Within the paper, there is later on a comparison between cc-pVTZ, Sadlej-pVTZ and aug-cc-pVTZ:

The Sadlej basis set yields results that are improved over the cc-pVTZ basis set and have effectively the same accuracy as the aug-cc-pVTZ basis set.

That’s why I chose Sadlej’s basis set at first … but I wanted to look if it could not be easier hence faster. Therefore I also chose the def2-SVPD and looked, what an improvement a def2-TZVPD single point calculation on this geometry would yield.

I use ORCA 3.0.3 for calculations – the command line is like the following:

! B3LYP Sadlej-pVTZ TightSCF TightOpt

Table 1: "Pure" dipole moments (in Debye) straight from the calculations \begin{array}{lllll} \hline & \text{def2-SVPD} & \text{def2-TZVPD} & \text{Sadlej-pVTZ} & \text{PM6}\\ \hline \text{4-Nitroacetanilide} & 9.18261 & 9.00380 & 9.07922 & 8.6738\\ \textit N\text ,\textit N\text{-Dimethyl…} & 7.39418 & 7.29188 & 7.30862 & 9.4209\\ \textit P\text ,\textit P\text{-Dimethyl…} & 8.58913 & 8.44430 & 8.51591 & 11.8352\\ \text{all-}\textit{cis }\ce{C6H6F6} & 6.17422 & 5.97013 & 6.04794 & 5.6298\\ \hline \end{array}

What I did then, was to think of how to produce values that could be properly compared to the experimental values from the paper. As they said, with their methods they can yield RMSDs of around 0.12 D. So I took their values for the Sadlej basis set and made my own calculations on their test molecules for the two def2 basis sets (and also for PM6 with Gaussian 09 Rev. A.02).

Table 2: Some analytical values to compare with the publication values; the units are in Debye \begin{array}{lllll} \hline & \text{def2-SVPD} & \text{def2-TZVPD} & \text{Sadlej-pVTZ} & \text{PM6}\\ \hline \text{MAE} & 0.08 & 0.08 & 0.08 & 0.40\\ \text{RMSD} & 0.13 & 0.12 & 0.12 & 0.61\\ \hline \end{array}

So for my two ways, the MAE and the RMSD are not too far away from the triple zeta values but are calculated way faster. Now I have made a linear regression between the experimental and the calculated dipole moments from Hickey and Rowley’s test molecules to be able – as said before – to calculate proper error ranges at a given probability.

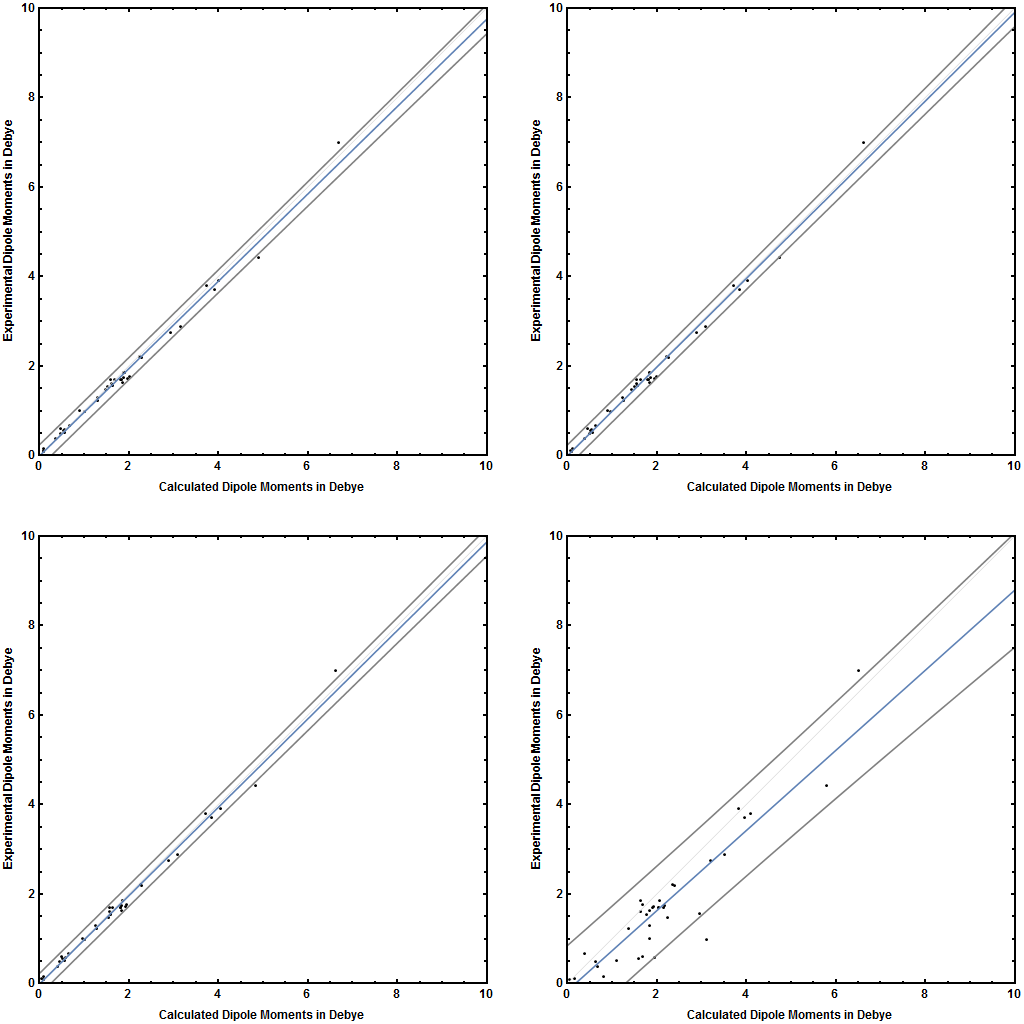
Figure 1: Regression functions for the four tested methods (blue), prediction intervals at 95% (gray); from top left to bottom right: def2-SVPD, def2-TZVPD//def2-SVPD, Sadlej-pVTZ, PM6

Now let’s turn the “pure” values from Table 1 into somehow more real values:

\begin{align} \text{def2-SVPD} \to \text{exp}&: -0.0097439128 + 0.9763275\ x\\ \text{def2-TZVPD//def2-SVPD} \to \text{exp}&: -0.0045050428 + 0.99095691\ x\\ \text{Sadlej-pVTZ} \to \text{exp}&: -0.012922547 + 0.98812009\ x\\ \text{PM6} \to \text{exp}&: -0.16057241 + 0.89545049\ x\\ \end{align}

Prediction Intervals (for the error):

$$\small\begin{align} \text{def2-SVPD} \to \text{exp}&: 0.24473369 \sqrt{1.0217391 + 0.010348758 (x-1.4590217)^2}\\ \text{def2-TZVPD//def2-SVPD} \to \text{exp}&: 0.23719452 \sqrt{1.0217391 + 0.010656662 (x-1.4321957)^2}\\ \text{Sadlej-pVTZ} \to \text{exp}&: 0.23333979 \sqrt{1.0217391 + 0.010593477 (x-1.4448261)^2}\\ \text{PM6} \to \text{exp}&: 0.98446665 \sqrt{1.0217391 + 0.0097476423 (x-1.7592391)^2} \end{align}$$

Table 3: Converted dipole moments (in Debye) from Table 1 including error ranges at 95% probability

\begin{array}{llll} \hline & \text{def2-SVPD} & \text{def2-TZVPD} & \text{Sadlej-pVTZ} & \text{PM6}\\ \hline \text{4-Nitroacetanilide} & 8.96 \pm 0.31 & 8.92 \pm 0.30 & 8.96 \pm 0.30 & 7.61 \pm 1.20 \\ \textit N\text ,\textit N\text{-Dimethyl…} & 7.21 \pm 0.29 & 7.22 \pm 0.28 & 7.21 \pm 0.27 & 8.28 \pm 1.24 \\ \textit P\text ,\textit P\text{-Dimethyl…} & 8.38 \pm 0.30 & 8.36 \pm 0.29 & 8.40 \pm 0.29 & 10.44 \pm 1.40 \\ \text{all-}\textit{cis }\ce{C6H6F6} & 6.02 \pm 0.27 & 5.91 \pm 0.26 & 5.96 \pm 0.26 & 4.88 \pm 1.06 \\ \hline \end{array}

What can be seen from this now? The smaller B3LYP/def2-SVPD method yields nearly the same values as the more expensive Sadlej version. Putting the def2-TZVPD single point on top of them, yields slightly deeper results with actually no accuracy improvements. PM6 is no real good option as the error ranges are way higher, with values of about $1-1.4$ D.

In the end, the dipole moment of all-cis 1,2,3,4,5,6-hexafluorocyclohexane is probably somewhere around $6$ D but there are probably many other molecules with higher dipole moments … “my” three molecules shown above go at least up to somewhere around $9$ D.

As long as no experimental values are available, everything here is only a good guess – but you will know this too good.

---

Sources:

1. O’Hagan et al., Nature Chemistry, 2015, 7, 483–488
2. http://pqr.pitt.edu/mol/NQRLPDFELNCFHW-UHFFFAOYSA-N
3. http://pqr.pitt.edu/mol/QJAIOCKFIORVFU-UHFFFAOYSA-N
4. http://pqr.pitt.edu/mol/FICBIFYYTQXSEY-UHFFFAOYSA-N
5. A. L. Hickey, C. N. Rowley, J. Phys. Chem. A, 2014, 118 (20), 3678–3687

Answer 2

At first I checked common organic compounds and solvents in CRC Handbook [1, 9-59] that have dipole moment above $\pu{4.5 D}$:

\begin{array}{llrr} \hline \text{Name} & \text{Formula} & \text{Atom count} & \mu, \pu{D} \\ \hline \text{Thiophenecarbonitrile} & \ce{C5H3NS} & 10 & 4.59(2) \\ \text{3-Methyl-2-butenenitrile} & \ce{C5H7N} & 13 & 4.61(13)\\ \text{Ethyl cyanate} & \ce{C3H5NO} & 10 & 4.72(9) \\ \text{Ethylene carbonate liq.} & \ce{C3H4O3} & 10 & 4.9 \\ \text{Propylene carbonate liq.} & \ce{C4H6O3} & 13 & 4.9 \\ \text{2-Nitroanisole liq.} & \ce{C7H7NO3} & 18 & 5.0 \\ \text{Hexamethylphosphoric triamide liq.} & \ce{C6H18N3OP} & 29 & 5.5 \\ \text{2-Pyridinecarbonitrile} & \ce{C6H4N2} & 12 & 5.78(11) \\ \text{Hydrogen cyanide trimer} & \ce{C3H3N3} & 9 & 10.6 \\ \hline \end{array}

Nothing special or really interesting except for $\ce{HCN}$ trimer, which is not really organic. Time to look at something more exotic.

---

Conjugated double bonds

German paper "Spektroskopische Untersuchungen an Merocyaninen I.) UV- Und NMR-Spektren von Malodinitril-Substituierten Vinylogen Säureamiden" [2] presents a series of malodinitrile-substituted vinylogous acidic amides of the following formula

$$\ce{Me2N-[CH=CH]_n-CH=C(CN)2} \qquad (n \in [0, 4])$$

and the following experimentally established ground-state ($S_0$) dipole moments:

\begin{array}{rlrr} \hline n & \text{Formula} & \text{Atom count} & \mu, \pu{D} \\ \hline 0 & \ce{C6H7N3} & 16 & 8.6 \\ 1 & \ce{C8H9N3} & 20 & 11.4 \\ 2 & \ce{C10H11N3} & 24 & 13.5 \\ 3 & \ce{C12H13N3} & 28 & 15.1 \\ 4 & \ce{C14H15N3} & 32 & 16.3 \\ \hline \end{array}

---

Aromatic compounds

From the recent paper "Hexasubstituted Benzenes with Ultrastrong Dipole Moments" [3] there are a few more candidates:

• 2,2‐diheptyl‐1,3‐dihydro‐1,3‐benzodiazole‐4,5,6,7‐tetracarbonitrile
  $\ce{C25H32N6}$ (63 atoms).
  $\mu_\mathrm{calcd} = \pu{12.7 D}$ (level-of-theory: DFT-B3LYP; basis set: 6-311 ++G(d,p));
  $\mu_\mathrm{exp} = \pu{12.2\pm0.3 D}$ (dielectric permittivity measurements in DMAc).
  

• 2,2‐dimethyl‐1,3‐dihydro‐1,3‐benzodiazole‐4,5,6,7‐tetracarbonitrile
  $\ce{C13H8N6}$ (27 atoms).
  $\mu_\mathrm{calcd} = \pu{12.3 D}$ (level-of-theory: DFT-B3LYP; basis set: aug-cc-pVTZ);
  $\mu_\mathrm{exp} = \pu{10.9\pm0.3 D}$ (dielectric permittivity measurements in THF).
  

---

Zwitterions

As stated in a handbook "Solvents and Solvent Effects in Organic Chemistry" [4, p. 79], the highest dipole moments among solvents found to date are probably zwitterionic 3-alkyl-1,2,-oxadiazolium-5-olates (sydnones). For example, 4-ethyl-3-(1-propyl)sydnone $\ce{C7H12N2O2}$ (23 atoms) has a dipole moment of $\pu{10.7 D}$ [5].

Another zwitterion and multifunctional dye, Brooker's merocyanine $\ce{C14H13NO}$ (29 atoms), has ground state dipole moment of $\pu{26 D}$ in trichloromethane, and which overall greatly depends on solvent polarity [4, 6].

In 2021 Henry Rzepa proposed another potential candidate in his blog, cycloprop‐2‐en‐1‐ylium‐1‐ylethyne (7 atoms), with the calculated dipole moment $\pu{11.9 D}$ in chloroform.

Bibliography

1. Haynes, W. M.; Lide, D. R.; Bruno, T. J. CRC Handbook of Chemistry and Physics: A Ready-Reference Book of Chemical and Physical Data.; 2017; Vol. 97. ISBN 978-1-4987-5429-3.
2. Scheibe, P.; Schneider, S.; Dörr, F.; Daltrozzo, E. Berichte der Bunsengesellschaft für physikalische Chemie 1976, 80 (7), 630–638 DOI: 10.1002/bbpc.19760800711.
3. Wudarczyk, J.; Papamokos, G.; Margaritis, V.; Schollmeyer, D.; Hinkel, F.; Baumgarten, M.; Floudas, G.; Müllen, K. Angewandte Chemie International Edition 2016, 55 (9), 3220–3223 DOI: 10.1002/anie.201508249.
4. Reichardt, C.; Welton, T. Solvents and Solvent Effects in Organic Chemistry, 4th ed.; Wiley-VCH: Weinheim, Germany, 2011.
5. Handa, M.; Kataoka, M.; Wakaumi, M.; Sasaki, Y. BCSJ 1997, 70 (2), 315–320 DOI: 10.1246/bcsj.70.315.
6. Morley, J. O.; Morley, R. M.; Docherty, R.; Charlton, M. H. J. Am. Chem. Soc. 1997, 119 (42), 10192–10202 DOI: 10.1021/ja971477m.

Answer 3

Calicene ($4.66~\pu{D}$, 14 atoms) and its derivatives are also good candidates.

For Hexaphenylcalicene, a hydrocarbon with 74 atoms, a dipole moment of $6.3~\pu{D}$ has been reported.^[1] 2,3-dicyano-5,6-diphenylcalicene (34 atoms) has a dipole moment of $14.3~\pu{D}$.^[2]

It would be interesting to see how two conjugate double bonds between the rings would influence the dipole moment

---

References

1. E.D. Bergman, I. Agranat, Chem. Commun, 512 (1965).
2. Houben-Weyl, Methods of Organic Chemistry, Vol. E 17d, 4th Edition, p. 2967.