I think the key to this question lies in the understanding of the difference between thermodynamic and kinetic stability. In this post, I find Thomij's answer the most rigorous and enlightening.
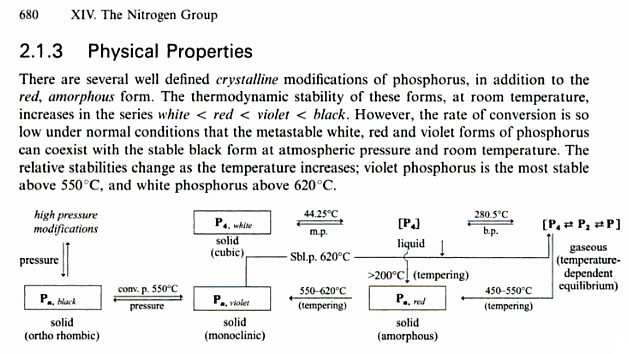
From this you should learn, that the thermodynamically most stable allotrope corresponds to the global minimum on the potential energy surface, while a kinetically stable allotrope, i.e. hindered through an activation barrier, corresponds to local minimum. From this it can easily be seen, that the thermodynamically most stable allotrope is also the kinetically most stable allotrope. This is due to the fact, that the barriers for interconversion of the different allotropes always have to be higher for the most stable one.
In the following two dimensional case you see that the thermodynamically most stable, i.e. the global minimum, is surrounded by two local minima. It must always be true, that $E_\mathrm{a}(1\to2)<E_\mathrm{a}(2\to1)$ if $1$ is a local and $2$ is a global minimum. The same applies to the other case. If this was not true, then $2$ cannot be the global minimum and hence not the thermodynamically most stable modification.
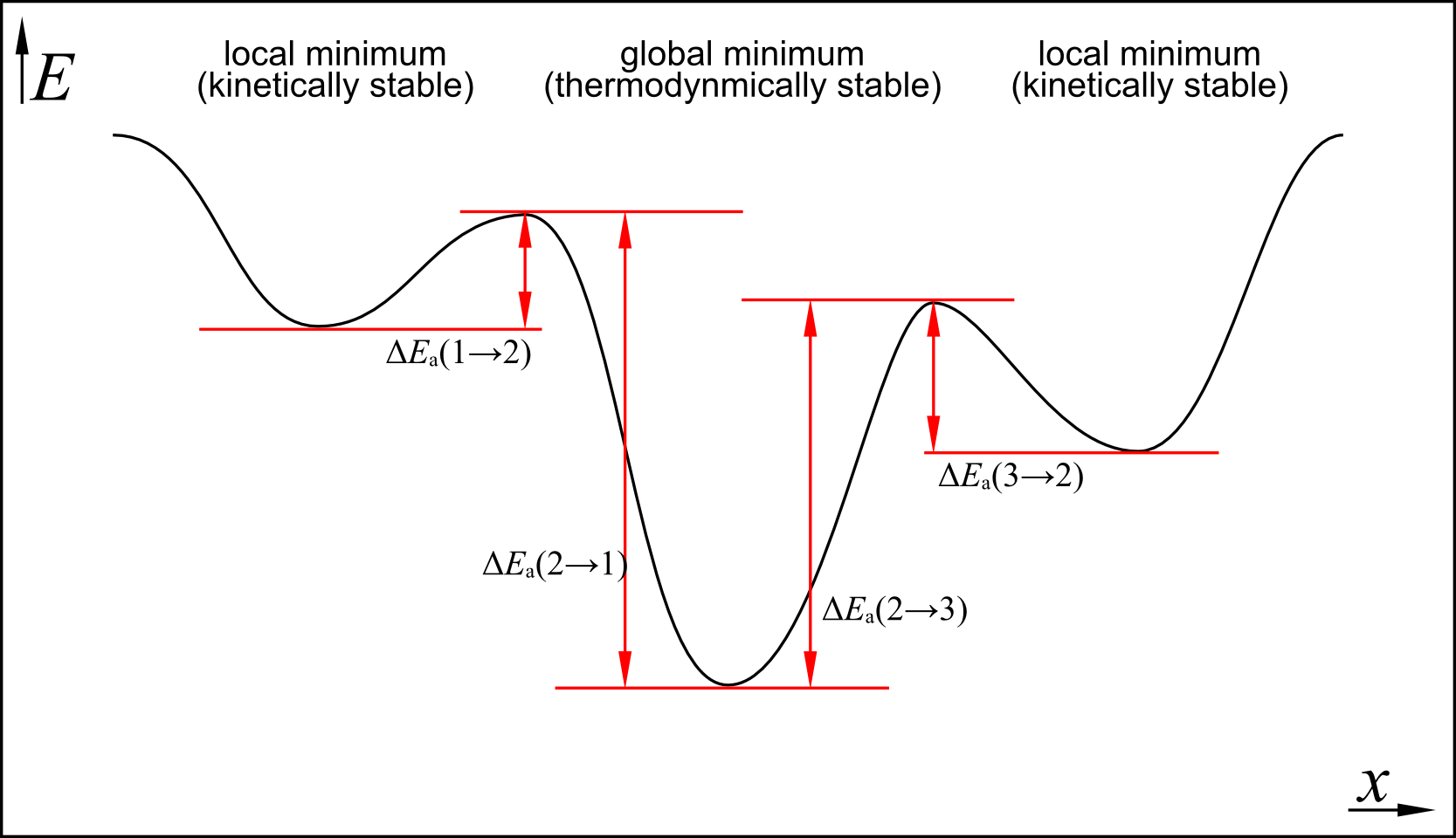
Having established that, it is therefore true, that black phosphorus is the thermodynamically and kinetically most stable form, see Hollemann-Wiberg, p. 680.
However, looking at a different reaction, the case might not be so simple any more. This is even true for different temperatures, as the following graphic will show (quoted from the linked book).