The change in enthalpy of carbon in diamond form to graphite is negative. This suggests that graphite should be more stable than diamond. Is it true?
What I thought was that graphite has van der Waals forces while diamond has covalent forces so diamond should be more stable. But the melting point of graphite is more than diamond which implies that it is thermodynamically more stable. I am thoroughly confused. So which is more stable?
At normal room temperature and pressure graphite is (slightly) more stable than diamond. But the melting point is not a good indicator of this.
Melting points are determined by the bonding structure of the solid and any potential liquid (indeed there may not be a liquid phase at some pressures). They don't necessarily tell you whether a different bonding structure is more thermodynamically stable.
The thermodynamic stability of different carbon structures depends on subtle tradeoffs among several factors in the bonding of the various solids (allotropes) than can be formed with different bonding arrangements. There is no easy way to tell which is the most thermodynamically stable other than by measurement or some very high-powered calculations about the quantum mechanics of the bonding.
The different allotropes have radically different structures (tetrahedral in diamond, a ball of hexagons and pentagons in buckminsterfullerene and flat plates of hexagons in graphite). Changing one into another requires a lot of bond breaking which isn't going to happen much at room temperature. Interconversion is possible at high pressures and temperatures when carbon is dissolved in liquid rock or metal and the carbon allotrope that forms is more likely to be whatever is stable at that whatever the pressure is (diamond is more stable at higher pressure which is why the earth or industry needs very high pressures to create diamond).
So, for most practical purposes, it doesn't matter than graphite is the more stable allotrope: your diamonds are not going to spontaneously turn to ash during your, or the universe's, lifetime.
This questions calls for an answer from thermodynamics.
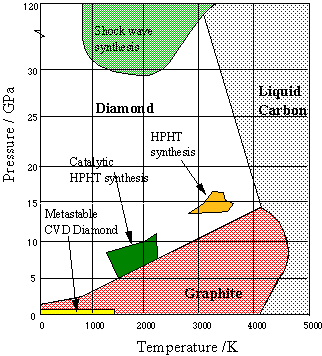
The figure provided above, lifted from here, is what we call a phase diagram. On the abscissa is temperature in units of Kelvin and on the ordinate is the pressure given in units of Gigapascals. (For your own reference, 1 GPa is nearly 10,000 times the pressure we live under, the earth's core is estimated to be over 300 GPa!) This diagram represents the input of experimental work and extrapolation with mathematical tools provided by thermodynamics. What this figure shows very clearly is that for a given temperature and pressure which phase is thermodynamically preferred.
A line between the diamond and graphite phases represents a phase boundary, and tell us precisely what temperature and pressure are required for these two phases to be in equilibrium. The phase boundary between liquid carbon and graphite represents the melting temperature for graphite; the same can be said for the diamond/carbon line, this again is the melting temperature of diamond.
Our melting temperature therefore depends on phase and pressure. So if you only have two melting points, does that really tell you which is thermodynamically preferred at room pressure (0.0001 GPa)? No, it does not. It is more subtle than that.
So how would we determine the relative stability of graphite and diamond at room temperature and pressure? For that we compute the standard Gibbs energy of reaction (at STP), this requires we use some values from a table: $\Delta G_f^0 (diamond) = 2.90 (kJ/mol)$ and $\Delta G_f^0 (graphite) = 0 (kJ/mol)$.
The reaction we want to calculate is: $C(diamond) \rightarrow C(graphite)$
Now we know that $\Delta G_{rxn} = \Sigma_{products} \Delta G_{f} - \Sigma_{reactants} \Delta G_{f} = 0 - 2.90 = -2.90 (kJ/mol)$. So $\Delta G_{rxn} < 0$ tells us at 298 K and 1 atm of pressure, diamond would spontaneously form graphite if it could; however, for this transition carbon atoms would have to change their location within a lattice and the rate at which this happens in a solid tends to be very slow at low temperatures. Hence, we call diamond a metastable phase under STP.
Now if you provide a sufficient energy to get over this activation barrier, then you will be able to see what thermodynamics prefers. Personally, I find this video very compelling.
