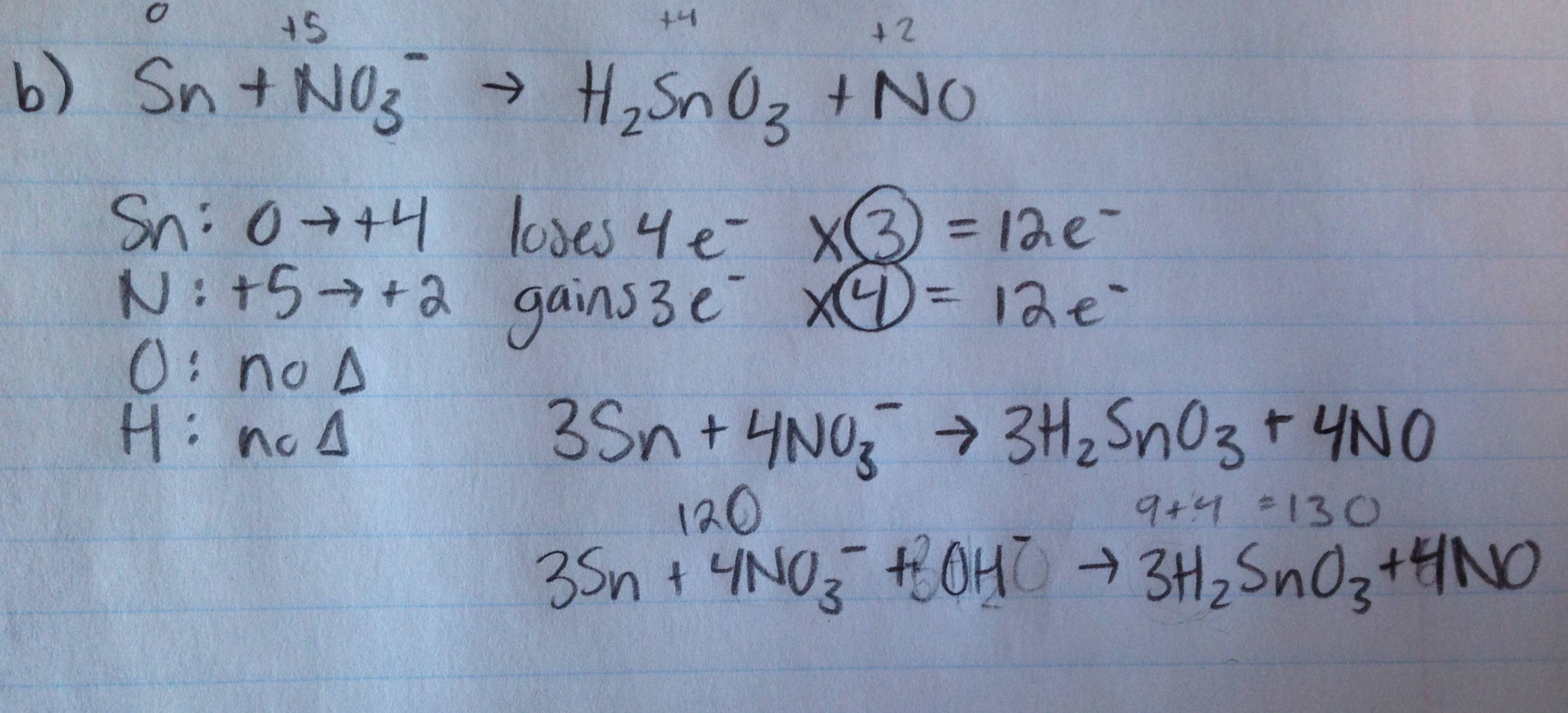I am trying to balance the REDOX equation shown below, but I got stuck after balancing the major atoms. Where can I go from here? I know I need to balance the H and O atoms, and make sure the charges are balanced.
Is this correct?
Whenever you balance a redox reaction in acidic or basic solution, you have to account for interactions with the solution itself. The easiest way of doing this is by the half-reaction method.
This is a key detail that I find a lot of students miss - you cannot balance a reaction like this without considering the solution, because the solution itself is participating. Your clue that this is happening is the phrase "in acidic (or basic) solution" - any time you see that in a redox problem, you should be thinking "half-reaction method." Another clue is that H and O appear on only one side of the equation. If you are familiar with the algebraic method of balancing equations, another clue is that the system of equations would be unsolvable.
At any rate, once you know that you need to use the half-reaction method, the next step is to use it!
This tutorial has a good description of the method, your book probably does as well, and I'll give a brief description here for good measure.
These are the major steps:
I'll use an example from my copy of Brown, LeMay, Bursten and Murphy's "Chemistry: The Central Science" to demonstrate (all phases are aqueous):
Balance the following reaction in acidic solution:
$\ce{MnO4^- + C2O4^2- -> Mn^2+ + CO2}$
Break the reaction into two half-reactions:
$\ce{MnO4^- -> Mn^2+}$
$\ce{C2O4^2- ->CO2}$
It's not always easy to see how to do this. The quick way is to make sure that the elements that are not O and H are in their own equations. The proper way is to figure out what is being reduced and what is being oxidized, then split them so that the oxidation happens in one reaction and the reduction happens in the other. In this case, Mn is reduced and C is oxidized.
Balance everything except for H and O:
$\ce{MnO4^- -> Mn^2+}$
$\ce{C2O4^2- -> 2CO2}$
This step is usually pretty easy.
Balance O by adding $\ce{H2O}$:
$\ce{MnO4^- -> Mn^2+ + 4H2O }$
$\ce{C2O4^2- -> 2CO2}$
We start with O because we are using water to balance it. Each time we add a water, we also add two H, so it doesn't make sense to start with H.
Balance H by adding $\ce{H^+}$:
$\ce{8H^+ + MnO4^- -> Mn^2+ + 4H2O }$
$\ce{C2O4^2- -> 2CO2}$
Remember that we can do this because it's in acidic solution. You are probably wondering what we do for basic solution. The answer is - use protons! There is a trick we can do at the end to "fix" it for basic solutions, and this is much easier than iterating between $\ce{OH^-}$ and $\ce{H2O}$
Balance charge by adding electrons:
$\ce{5e^- + 8H^+ + MnO4^- -> Mn^2+ + 4H2O }$
$\ce{C2O4^2- -> 2CO2 + 2e^-}$
While this is easy to understand, I have found that in practice, a majority of mistakes happen here. Be careful when counting charges - remember to multiply by the coefficients, and remember that the charges don't have to add up to zero yet - they just have to have the same sum on both sides.
Multiply the half-reactions by the appropriate coefficients so that when they are added together, the charges (electrons) cancel:
$\ce{10e^- + 16H^+ + 2MnO4^- -> 2Mn^2+ + 8H2O }$
$\ce{5C2O4^2- -> 10CO2 + 10e^-}$
This part is also prone to error - be careful and remember to multiply every species by the coefficent.
Add the equations together
$\ce{16H^+ + 2MnO4^- + 5C2O4^2- -> 2Mn^2+ + 8H2O + 10CO2 }$
If the electrons don't cancel out here, then you made a mistake - go back and check each step.
If the solution is basic, at this point you would add $\ce{OH-}$ to both sides of the equation until the 16 protons were eliminated. We can add anything we want as long as it's to both sides (since this is, after all, just algebra). Each $\ce{OH^-}$ will react with a proton to form one water molecule, and so the waters on the right would cancel out some of the waters on the left. The result would be a similar equation, but with $\ce{OH^-}$ instead of $\ce{H^+}$.
Try out your problem using these steps, and if you get stuck, update your question.
1- Determining oxidation number, Oxidation and Reduction: $$ \ce{ \overset{\color{red}{(0)}}{Sn}+ \overset{\color{green}{(+5)}}{N} \overset{\color{ black }{(-2)}}{~O3^-} -> \overset{\color{black}{(+1)}}{H2} \overset{\color{red}{(+4)}}{Sn}\overset{\color{ black }{(-2)}}{O3} +\overset{\color{green}{(+2)}}{N} \overset{\color{ black }{(-2)}}{O} }$$ The atom $\ce{N}$: went from $\color{green}{(+5)}$ to $\color{green} {(+2)}$, it gains electrons, so it is reduced.
The atom $\ce{Sn}$: went from $\color{red}{(0)}$ to $\color{red}{(+4)}$, it lost electrons, so it is oxidized.
2- Separate the redox reaction into two half-reactions: one for the oxidation, and one for the reduction
$$\text {Oxidation half}: \ce{ \overset{\color{red}{(0)}}{Sn} -> \overset{\color{black}{(+1)}}{H2} \overset{\color{red}{(+4)}}{Sn}\overset{\color{ black }{(-2)}}{O3} }$$ $$\text {Reduction half}: \ce{ \overset{\color{green}{(+5)}}{N} \overset{\color{ black }{(-2)}}{O3^-} -> \overset{\color{green}{(+2)}}{N} \overset{\color{ black }{(-2)}}{O} }$$ 3- Balance all elements except oxygen and hydrogen in both half equations
$$\text {Oxidation half}: \ce{ \overset{\color{red}{(0)}}{Sn} -> \overset{\color{black}{(+1)}}{H2} \overset{\color{red}{(+4)}}{Sn}\overset{\color{ black }{(-2)}}{O3} }$$ $$\text {Reduction half}: \ce{ \overset{\color{green}{(+5)}}{N} \overset{\color{ black }{(-2)}}{O3^-} -> \overset{\color{green}{(+2)}}{N} \overset{\color{ black }{(-2)}}{O} }$$ 4- Add the appropriate number of water molecules to that side of the equation required to balance the oxygen atoms :
$$\text {Oxidation half}: \ce{ \overset{\color{red}{(0)}}{Sn} + 3{H2O}-> \overset{\color{black}{(+1)}}{H2} \overset{\color{red}{(+4)}}{Sn}\overset{\color{ black }{(-2)}}{O3} }$$ $$\text {Reduction half}: \ce{ \overset{\color{green}{(+5)}}{N} \overset{\color{ black }{(-2)}}{O3^-} -> \overset{\color{green}{(+2)}}{N} \overset{\color{ black }{(-2)}}{O}+2{H_2O} }$$ 5- Add the appropriate number of hydrogen ions to that side of the equation required to balance the hydrogen atoms: $$\text {Oxidation half}: \ce{ \overset{\color{red}{(0)}}{Sn} + 3{H_2O}-> \overset{\color{black}{(+1)}}{H2} \overset{\color{red}{(+4)}}{Sn}\overset{\color{ black }{(-2)}}{O3}+4{H^+} }$$ $$\text {Reduction half}: \ce{ \overset{\color{green}{(+5)}}{N} \overset{\color{ black }{(-2)}}{O3^-}+4{H^+} -> \overset{\color{green}{(+2)}}{N} \overset{\color{ black }{(-2)}}{O}+2{H_2O} }$$ 6- Use electrons ($\ce{e^-}$) to equalize the net charge on both sides of the equation: $$\text {Oxidation half}: \ce{ \overset{\color{red}{(0)}}{Sn} + 3{H_2O}-> \overset{\color{black}{(+1)}}{H2} \overset{\color{red}{(+4)}}{Sn}\overset{\color{ black }{(-2)}}{O3}+4{H^+} + 4{e}^- }$$ $$\text {Reduction half}: \ce{ \overset{\color{green}{(+5)}}{N} \overset{\color{ black }{(-2)}}{O3^-}+4{H^+} +3{e}^- -> \overset{\color{green}{(+2)}}{N} \overset{\color{ black }{(-2)}}{O}+2{H_2O} }$$ 7- Make the electrons gained equal to the electrons lost: $$\text {Oxidation half}: \ce{ 3\overset{\color{red}{(0)}}{Sn} + 9{H_2O}-> 3\overset{\color{black}{(+1)}}{H2} \overset{\color{red}{(+4)}}{Sn}\overset{\color{ black }{(-2)}}{O3}+12{H^+} + 12{e}^- }$$ $$\text {Reduction half}: \ce{ 4\overset{\color{green}{(+5)}}{N} \overset{\color{ black }{(-2)}}{O3^-}+16{H^+} +12{e}^- -> 4\overset{\color{green}{(+2)}}{N} \overset{\color{ black }{(-2)}}{O}+8{H_2O} }$$ 8- Combine the two half-reactions:
$\ce{3\overset{\color{red}{(0)}}{Sn} + 9{H_2O} +4\overset{\color{green}{(+5)}}{N} \overset{\color{ black }{(-2)}}{O3^-}+16{H^+} +12{e}^-->3\overset{\color{black}{(+1)}}{H2}\overset{\color{red}{(+4)}}{Sn}\overset{\color{ black }{(-2)}}{O3}+12{H^+} + 12{e}^- +4\overset{\color{green}{(+2)}}{N} \overset{\color{ black }{(-2)}}{O}+8{H_2O} } $
9- Cancele out the electrons and anything that is the same on both sides then collect the things that is the same on the same side to form one balanced redox equation: $\ce{3\overset{\color{red}{(0)}}{Sn} + {H_2O} +4\overset{\color{green}{(+5)}}{N}{O_3^-} +4{H^+} ->3{H_2}\overset{\color{red}{(+4)}}{Sn}{O_3} +4\overset{\color{green}{(+2)}}{N} {O} } $
