Fill a pressure vessel with water, no airspace. Heat it past 374 Degrees C, so that the water goes supercritical but can't expand. What happens to the pressure at that point? Does it continue to increase linearly as the temperature continues to increase, or would the graph form a hockey stick there, as the rate of increase (pressure) suddenly increased, or does it increase exponentially (curved graph) or????
Put another way, is the pressure at 700 Kelvin twice that at 350 K, or???
Typically, isochores in a $p-T$ diagram are straight lines. This is evident in the ideal gas law, but also a prediction from the simple van der Waals' equation of state.
We put this to test using a more sophisticated equation of state, the Peng Robinson's equation of state \begin{equation} p = \frac{RT}{V - b} - \frac{a}{[V + (1 - \sqrt{2})b)][V + (1 + \sqrt{2})b]} \tag{1} \end{equation} Even though it still has two parameters, the one related to the attraction forces has a dependency with temperature \begin{equation} a = 0.45724\,\frac{\alpha(T_\mathrm{r},\omega)R^2T_c^2}{p_\mathrm{c}} \tag{2} \end{equation} in which the accentric factor is taken into account via the correlation established by Peng and Robinson \begin{equation} \alpha(T_\mathrm{r},\omega) = [1 + (0.37464 + 1.54226\omega - 0.26992\omega^2)(1 - \sqrt{T_r})]^2 \tag{3} \end{equation}
The results are shown below:
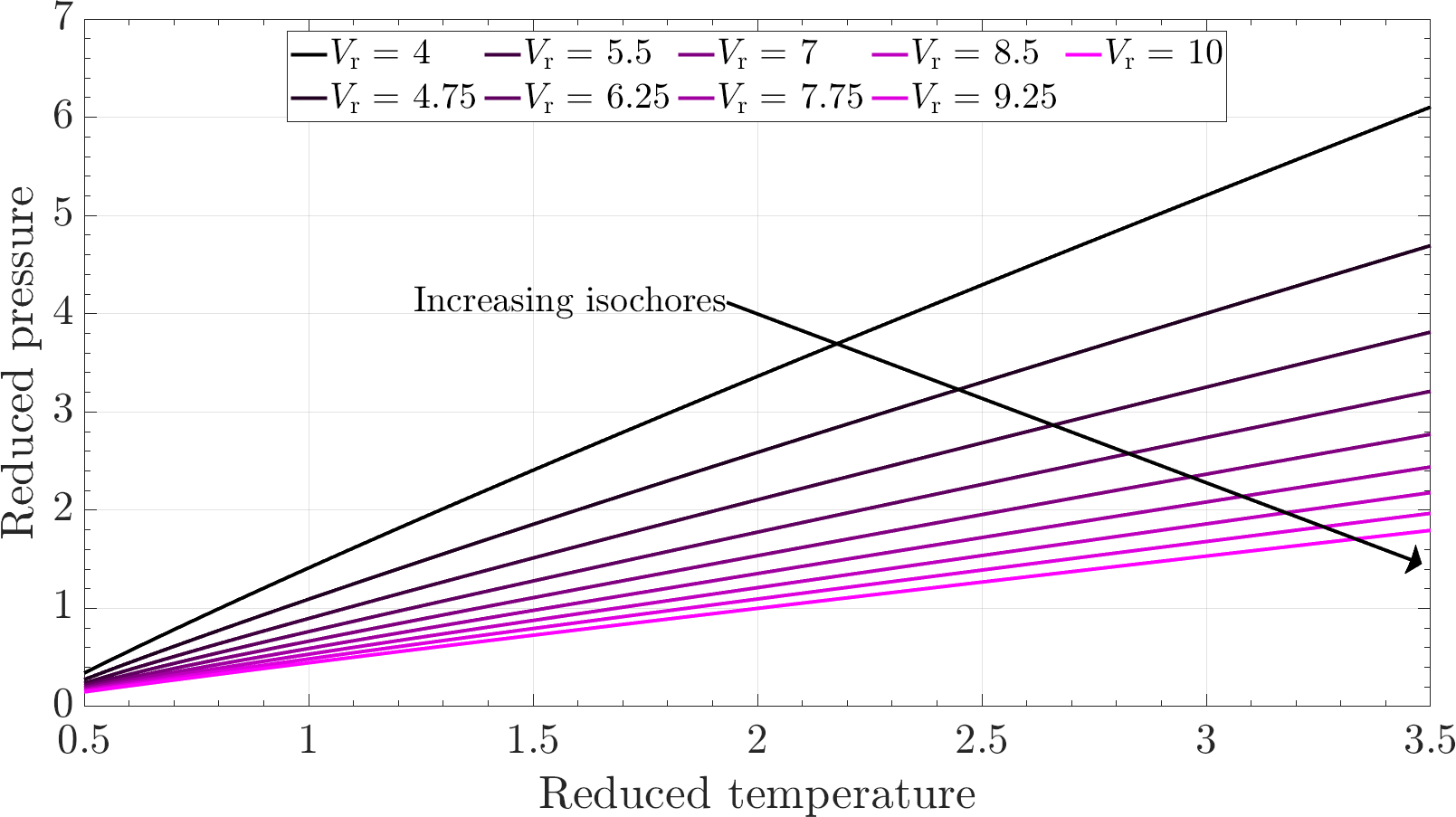
This is a $p - T$ diagram but in reduced coordinates. There are ten isochores which are also displayed in reduced form. The supercritical region that interests you lies in the rectangle for which $p_\mathrm{r} > 1$ and $T_\mathrm{r} > 1 $. When $T_\mathrm{r}$ is rather low, there seems to be some curvature in all the isochores due to that both terms are important in the equation. However, for the supercritical region we have essentially straight lines. This agrees with the explanation given by Oscar Lanzi.
References
\begin{array}{|c|c|c|c|} \hline T_\mathrm{c} \; \pu{(K)} & p_\mathrm{c} \; \pu{(bar)} & V_\mathrm{c} \; \pu{(dm3/mol)} & \text{Accentric factor} \\ \hline 647.1 & 220.55 & 0.0559 & 0.345 \\ \hline \end{array}
Assuming that the vessel does not blow up, the "gas" pressure ultimately becomes proportional to absolute temperature, but the proportionality constant may be greater than that derived from the ideal gas law.
Illustrating with the Redlich-Kwong equation:
$P=\dfrac{RT}{V-b}-\dfrac{a}{\sqrt{T}V(V+b)},$
where P is pressure, V is molar volume, T is absolute temperature and $a,b$ are deemed constants for a given gas. Under the conditions assumed in the problem $V$ is also constant as the temperature is raised and therefore the first term becomes mathematically dominant. Thus
$P\approx\dfrac{RT}{V-b},$
which exceeds the ideal gas law through the "excluded volume" from the rigid structure in the gas molecules. The excluded volume effect is also present in ordinary gases, but the relative effect would be much larger in the pressure-vessel situation considered here.
Mostly way over my head--it would really help if you would define your variables--but I gather that pressure is proportional to temperature, and that crossing the supercritical point doesn't change that, so that twice the temperature (in Kelvins) means twice the pressure. Interesting that twice the pressure means half again the velocity in firearms, and twice the temperature seems to equal about half again the efficiency in Rankine-cycle power plants. Must be another law of thermodynamics in there ... . I'm thinking of the differences between pressurized water nuclear power plants and molten salt reactors, and if I got this right I got what I needed, so thanx, guyz.
