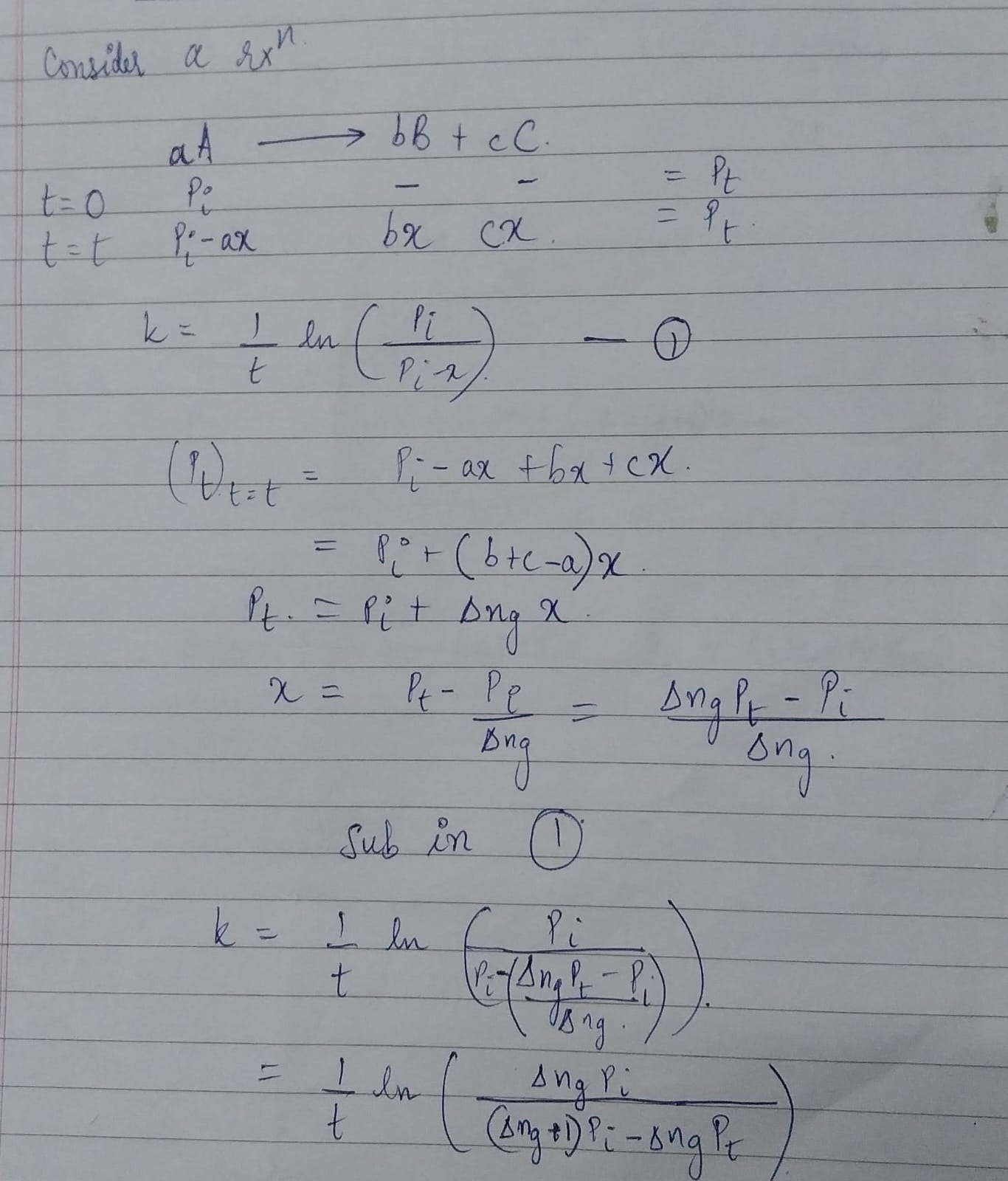0
$\begingroup$

There is a specific example cited in our NCERT Class 12 textbook, to find out the rate law equation for a general gaseous reaction, $$\ce{A->B + C}$$

It describes the entire procedure as shown:

Let us consider a typical first order gas phase reaction $\ce{A(g) -> B(g) + C(g)}$. Let $\pu{p_i}$ be the initial pressure of A and $\pu{p_t}$, the total pressure at time 'ť'. Integrated rate equation for such a reaction can be derived as $$\mathrm{Total \space pressure}\space\pu{p_t = p_A + p_B + p_C}\space \mathrm{(pressure\space units)}$$ If $\pu{x atm}$ be the decrease in pressure of A at time $t$ and one mole each of B and C is being formed, the increase in pressure of B and C will also be $\pu{x atm}$ each.
\begin{array}{|c|c|c|c|} \hline & \ce{A} & \ce{B} & \ce{C} \\ \hline \text{At } t = 0 & \pu{p_i} & 0 & 0 \\ \hline \text{At time } t & \pu{p_i - x} & x & x \\ \hline \end{array} where, $\pu{p_i}$ is the initial pressure at $t= 0$. $$\pu{p_t=(p_i-x)+x+x=p_i+x}$$ $$\pu{x=p_t-p_i}$$ where, $$\pu{p_A=p_i-x=p_i-(p_t-p_i)= 2p_i-p_t}$$ Finally, $$\pu{k=\frac{2.303}{t}log(\frac{p_i}{2p_i-p_t})}$$

Now I am trying to derive something for a general equation. The final formula would look like:

$$\pu{\frac{2.303}{t}log(\frac{(z-1)p_i}{zp_i-p_t})}$$ where z is number of gaseous products from one mole of gaseous reactant. Fine! But one thing: I want it with respect to difference in gas moles.

Considering the reaction $\ce{aA->bB + cC}$, I did try it, and got something like this.

enter image description here

Does anyone know any alternative method?

Improve this question
$\endgroup$
7
  • $\begingroup$ $\Delta n_g$ is the difference in gas moles, to clarify. $\endgroup$
    – Harikrishnan M
    Commented Jan 30 at 9:10
  • $\begingroup$ There seems to be an error in the LCM taking. You finally get $$\pu{k=\frac{2.303}{t}log(\frac{zp_i}{(z+1)p_i-p_t})}$$ but again that does not match the expression given. $\endgroup$
    – Harikrishnan M
    Commented Jan 30 at 9:14
  • $\begingroup$ The above comments are for V-1 of the question. $\endgroup$
    – Harikrishnan M
    Commented Jan 30 at 11:10
  • 1
    $\begingroup$ Note that using photos/screenshots of text instead of typing text itself is highly discouraged. The image text content cannot be indexed nor searched for, nor can be reused in answers. Specifically handwritten scripts can be difficult to decipher. Consider copy/pasting or rewriting of essential parts. // Optional: Formatting guides for texts and formulas/equations/expressions. $\endgroup$
    – Poutnik
    Commented Jan 30 at 11:22
  • $\begingroup$ @Poutnik the Mathjax of the same is quite cumbersome and the user is new. Also I feel that the crux of the question is covered in the typing itself. $\endgroup$
    – Harikrishnan M
    Commented Jan 30 at 12:03

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.