2
$\begingroup$

This doesn't look like a traditional inversion of configuration:

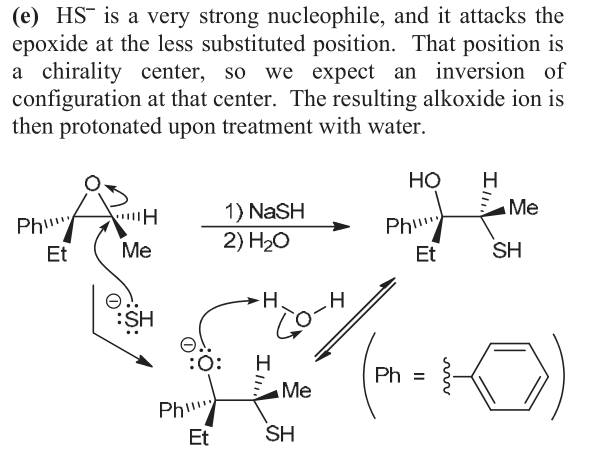
enter image description here

At first I dismissed the textbook author as making a typo. But then I realized that this "typo" was repeated throughout the solutions manual.

So I tried drawing a Sawhorse projection of the textbook's answer and my answer, and I found that both are equivalent.

So would my answer also be acceptable? In my answer I change the methyl from a wedge to dashes and the hydrogen from dashes to wedge and I place the SH group appropriately.

Improve this question
$\endgroup$
5
  • $\begingroup$ Do you mean adjacent to the OH? or opposite (like the drawing above)? It would really help to show what you have. $\endgroup$
    – LDC3
    Commented Aug 4, 2014 at 5:45
  • $\begingroup$ I have found that it can be difficult to recognize inversion when the leaving group and incoming nucleophile are both in the plane of the paper. The inversion in this case is really that the hydrogen and methyl are initially oriented "down" and are oriented "up" in the product. $\endgroup$
    – jerepierre
    Commented Aug 4, 2014 at 17:14
  • $\begingroup$ Also, in your answer, without wedges and dashes, it's impossible to say if you are correct. $\endgroup$
    – jerepierre
    Commented Aug 4, 2014 at 17:15
  • $\begingroup$ Well, the wedges and dashes aren't needed in a Sawhorse projection, because it's a pseudo-3D view of the molecule. Any substituent that looks like it's coming toward you is on a wedge in a flat, 2D representation. $\endgroup$
    – Dissenter
    Commented Aug 4, 2014 at 17:33
  • $\begingroup$ Sorry, I see what you're doing there. I was looking at it as a "line-wedge" drawing. $\endgroup$
    – jerepierre
    Commented Aug 5, 2014 at 18:00

1 Answer 1

Reset to default
1
$\begingroup$

It's slightly difficult for me to see clearly, but your drawing appears to be correct, and it corresponds to a $180^{\circ}$ rotation about the $\ce{C_1-C_2}$ bond axis relative to the textbook rendering ($\ce{C_1}$ being the carbon bearing the oxygen atom and $\ce{C_2}$ the sulfur atom, respectively). An unambiguous method of determining whether the two representations are equivalent is to assign the absolute configuration for the chiral carbon(s) in question. It may also help to use other projections (Fischer or Newman) if visualization of bond rotation and stereochemistry in the skeletal formula becomes difficult, as you did with the Sawhorse projection.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.