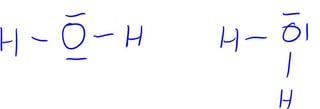Why is the molecular structure of water bent?

Question

Water has the formula $\ce{H2O}$ and we can draw a Lewis structure with two lone pairs on the central oxygen. As a physics student and not a chemist, I think to myself, "Okay, there are two lone pairs, they will repel each other" and so we arrive at the conclusion of bent shape.

The issue in my mind is that I don't see why the lone electron pairs have to exist on the same side of the atom. Also, wouldn't the Schrödinger equation provide an equally plausible structure for water with the lone pairs on the opposite side of the oxygen from what we assume (imaging the electrons on the top or on the bottom of the oxygen in the Lewis structure)? If that were true, then there would be a resonance structure between the two states and we would get a linear geometry. Clearly I'm running around in circles here, please someone enlighten me!

Answer 1

I mean, there is a time and place for VSEPR, and this is probably as good a time as any, because all beginning chemistry students go through it. The actual model has already been explained multiple times, so I will only briefly say that according to this theory, there are four pairs of electrons around the central oxygen. In order to minimise electron-electron repulsions, these pairs adopt a tetrahedral arrangement around the oxygen. It does not matter which two are lone pairs and which two are connected to hydrogen atoms; the resulting shape is always bent.

What's worth bearing in mind (and hasn't been explained very carefully so far) is that VSEPR is a model that chemists use to predict the shape of a molecule. The truth is that there is no real way to predict the shape of a molecule, apart from solving the Schrodinger equation, which is not analytically possible for water. Everything else is an approximation to the truth. Some of these approximations are pretty accurate, such as the use of density functional theory. Some of them are extremely crude, and VSEPR falls into this category: it essentially treats electrons as classical point charges, and seeks to minimise the electrostatic repulsion between these point charges. As a physics student you should know better than to do this. Thus, while it predicts the correct result in this case, it is more in spite of the model rather than because of the model. And you should not be surprised to hear that in some slightly more complicated cases, VSEPR can predict entirely wrong outcomes.

As you learn more chemistry you will find that there are increasingly sophisticated ways of explaining molecular geometry. Most revolve around molecular orbital theory. For a qualitative method, you have Walsh diagrams which have been explained at Why does bond angle decrease in the order H2O, H2S, H2Se?. For a more rigorous method you would likely have to run some quantum chemical computations, e.g. Are the lone pairs in water equivalent?. Of course, the drawback of this is that it becomes more and more difficult to extract true chemical understanding from the numbers. Although it should also be said that you cannot extract any true chemical understanding from the VSEPR model.

What interests me more is the followup question:

Also, wouldn't the Schrödinger equation provide an equally plausible structure for water with the lone pairs on the opposite side of the oxygen from what we assume (imaging the electrons on the top or on the bottom of the oxygen in the Lewis structure)?

Because the Hamiltonian of the water molecule is invariant upon rotation, this means that indeed, any orientation of the water molecule is equally likely. However, this only refers to the orientation of the water molecule as a whole. It does not say anything about the internal degrees of freedom, such as the bond angle.

In the absence of any external force, the molecule is free to bend in whichever direction it likes, and most water molecules indeed do do this as they float through space or swim in a lake. But it will always be bent.

If that were true, then there would be a resonance structure between the two states and we would get a linear geometry.

If you were to think of a single particle in a double-well potential, say something with

$$V = \begin{cases} \infty & x < -b \\ 0 & -b \le x \le -a \\ \infty & -a < x < a \\ 0 & a \le x \le b \\ \infty & x > b \end{cases}$$

then because of the symmetry of your system, in every eigenstate of your system, the expectation value of $x$ would be $\langle x \rangle = 0$. This is quite similar to your argument. In the case of water, let's set the oxygen nucleus to be at the origin. Because it can point either up or down, the expectation value of the hydrogen nucleus position along the up-down axis would be exactly level with the oxygen atom, i.e. 0. In fact, don't stop there: it can point to the left or the right, and to the front or the back. So the hydrogen nucleus has a position expectation value of exactly $(0, 0, 0)$, i.e. right inside the oxygen nucleus.

Does that mean it's actually there, though? In our contrived double-well system, it's patently impossible for the particle to be at $x = 0$, because $V = \infty$ there. If you were to measure its position, you would never find it at $x = 0$; you would only find it in the left-hand side $[-b, -a]$, or the right-hand side $[a,b]$. Just because the particle has an expectation value of $\langle x \rangle = 0$ does not mean that it is physically there, or that $x = 0$ is somehow its equilibrium state. You're confusing an expectation value with a genuine eigenstate (which is what a resonance structure is).

In exactly the same way, if you ever were to measure the properties of water (and bear in mind that practically every interaction with a water molecule is, in effect, a measurement), we would find that it is indeed always bent.

Answer 2

Lewis structures are dots around atoms on 2-dimensional paper. Although H-O-H is planar, when you throw in the lone pairs, you have to think three-dimensionally. How would oxygen be hybridized, in readiness to accept two incoming hydrogens, each with an electron?

Oxygen could hybridize as sp2p, with 120 degree angles between bonds to hydrogen and a 90 degree angle between the p2 lone pair and the sp2 lone pair. Or as sp3, with 109 degrees between all the bonds. In water, we have 104.5 degrees https://en.wikibooks.org/wiki/Structural_Biochemistry/Water. 104.5 is between 90 and 109, so there must be some accommodation between the repulsion of lone pairs, which would push toward sp3 hybridization, and perhaps some repulsion from the electrons in the O-H bonds, which would tend to spread the H-O-H angle toward 120 degrees (sp2) and push one of the lone pairs more completely into the p2 orbital.

I just can't decide which is the more powerful force; perhaps the water molecule can't either, so it just compromises.

Answer 3

Yet another way to think about the fact that $\ce{H2O}$ does not have the full symmetry theoretically possible, is that the number of electrons does not properly match the highest possible symmetry point group (which has the odd name $D_{\infty\mathrm{h}}$. In this sense it can be seen as simple example of symmetry breaking (its however not a simple first order Jahn-Teller distortion).

Answer 4

I don't see why the lone electron pairs have to exist on the same side of the atom.

Nobody says they exist "on the same side of the atom". In the picture showing Lewis structures of waters, the lone pairs are shown on opposite sides in the left panel , and on the same side on the right.

These two structures are identical. A Lewis structure does not make a statement about the geometry of a molecule.

If you look at a 3D model of water where the lone pairs are shown according to sp3-hybridization in the valence bond view of things, it depends on the orientation of the molecule whether it looks like "on opposite sides" or "on the same side" (the elongated shape - bunny ears - of the lone pairs is exaggerated; they should add up to a roughly spherical electron density). (Source: https://www.biotopics.co.uk/jsmol/watersingle.html)

In the molecular orbital view (where the molecular orbitals share the symmetry of the molecule) the two lone pairs have distinct shapes (each panel shows one lone pair, orientation of the molecule distinct from figure above).

This was calculated using molcalc.org. As these two molecular orbitals are of similar energy, you could make linear combinations of them to arrive at orbitals similar to the valence bond picture.

So what is the real picture? Looking at hydrogen bond geometries, either one describes the directions from which hydrogen acceptors on other molecules would be located, so both models are consistent with experimental data.

Answer 5

There are eight valence electrons on the oxygen that have similar energies then there is a gap and two electrons very close to oxygen core follow. These eight electrons form four spin-pairs and those point in directions that more or less minimise the repulsion. These directions are called "tetrahedral" in Chemistry language, since they point from the center (= oxygen atom core) to the vertices of an tetrahedron. Now the two protons are connected with two of those "electron pairs" and two are "alone". Hence you obtain the bent shape of H$_2$O.

(This is kind of trivialized version of the VSEPR model.)