How is the reaction rate constant affected by concentration?

Question

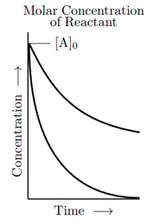
Consider the concentration-time dependence graph for two first-order reactions.

Which reaction has a larger rate constant?

1. Unable to determine
2. The reaction represented by the upper curve
3. The reaction represented by the lower curve correct

The above question is confusing me. It gives two concentrations vs. time curves, and asks which reaction has the larger rate constant. The answer is (3) the reaction represented by the lower curve.

I know that the reaction is first-order, hence $$\mathrm{rate} = k[A].$$ Thus, algebra reveals that $$[A] = \frac{\mathrm{rate}}{k}.$$ Thus, it makes sense that increasing the value of $k$ means dividing rate by a larger number, yielding a smaller concentration at any given time. This makes sense why the lower curve is the correct answer.

However, I also understand that the value of $k$, the rate constant, does not depend/is not correlated with the concentration of the reactant(s). Thus, is my logic not applicable? How else can you reason your way to the correct answer?

Answer 1

...I also understand that the value of k, the rate constant, does not depend/is not correlated with the concentration of the reactant(s).

It would be easier to represent this in calculus, but I assume you have not gotten there yet.

Anyway, you are correct, $k$ is a constant.

$$\mathrm{rate} = k [A]^x \implies \mathrm{rate} \propto k$$

thus as $k$ increases, the rate will increase for a given concentration of $\ce A$. The question did provide that $x = 1$ but this is not needed to solve the problem. Think of rate as: $$\mathrm{rate} = -\frac{\Delta [A]}{\Delta t}$$ (the reaction is removing $\ce A$) which is in the form of a slope. thus the larger the rate value, the lower the slope of the curve. Rate is dependend on concentration, but for the same concentration, a reaction with a higher rate constant will proceed faster, thus remove $\ce A$ fast and have a more negative slope.

Lets compare for the two $k$ values, $k_{upper}$ and $k_{lower}$

$$\mathrm{rate}_{upper} = k_{upper}[\ce A]= -\frac{\Delta [A]}{\Delta t} \\ \text{and }\\ \mathrm{rate}_{lower} = k_{lower}[\ce A]= -\frac{\Delta [A]}{\Delta t} $$

Lets say arbitrarily that two reaction rates are: $k_{1} = \pu{2 s-1}$ and $k_{2} = \pu{4 s-1}$ and the instantaneous concentration is $[\ce A] = \pu{0.6M}$ then the reaction rates would be as follows:

$$\mathrm{rate}_{1} = \pu{2 s-1}\times \pu{0.6M} = \pu{1.2 M s-1} = -\frac{\Delta [A]}{\Delta t} \implies \frac{\Delta [A]}{\Delta t} = -\pu{1.2 M s-1}\\ \text{and }\\ \mathrm{rate}_{2} = \pu{4 s-1}\times \pu{0.6M} = \pu{2.4 M s-1} = -\frac{\Delta [A]}{\Delta t} \implies \frac{\Delta [A]}{\Delta t} = -\pu{2.4 M s-1}$$

Since a reaction of $\mathrm{rate}_2$ has a more negative slope, it must correspond to a curve that decreases faster than a reaction of $\mathrm{rate}_1$ and the concentration would be lower sooner, thus $\mathrm{rate}_2$ is the lower curve and $\mathrm{rate}_1$ is the upper curve.