4
$\begingroup$

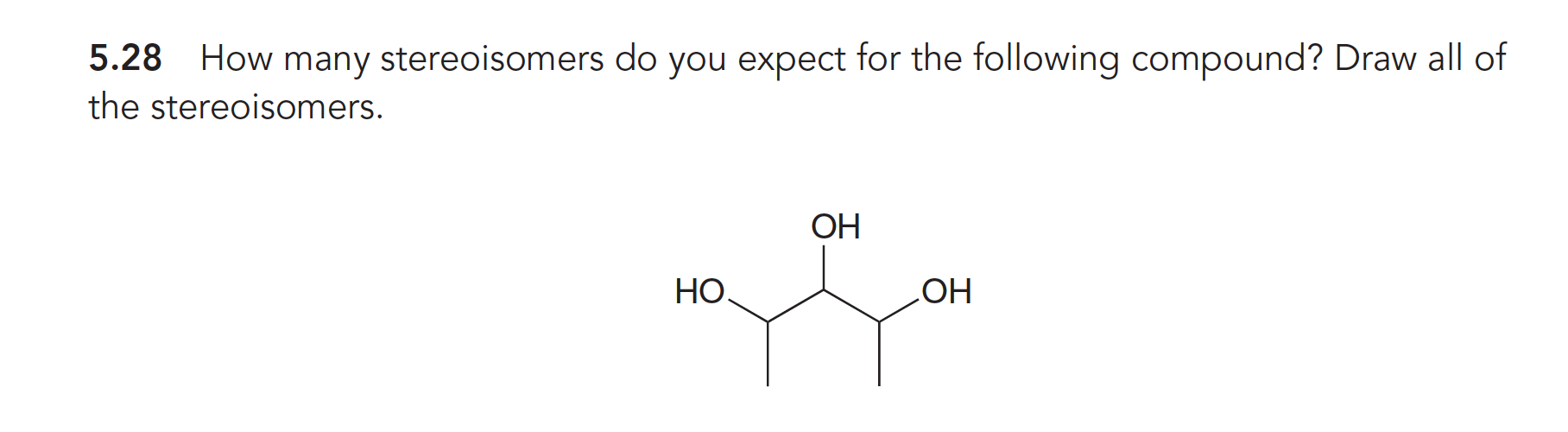
enter image description here

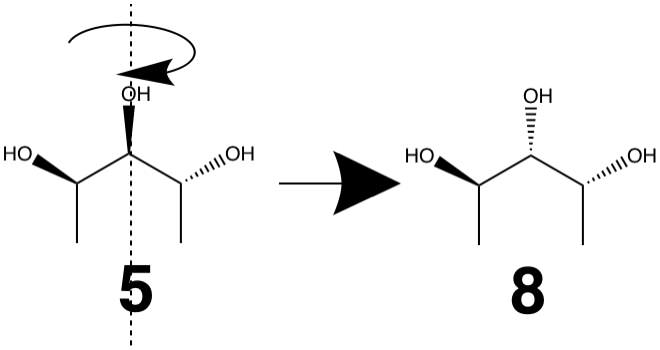
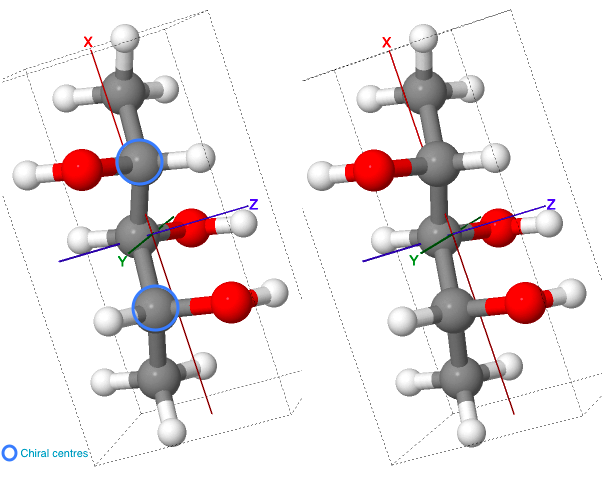
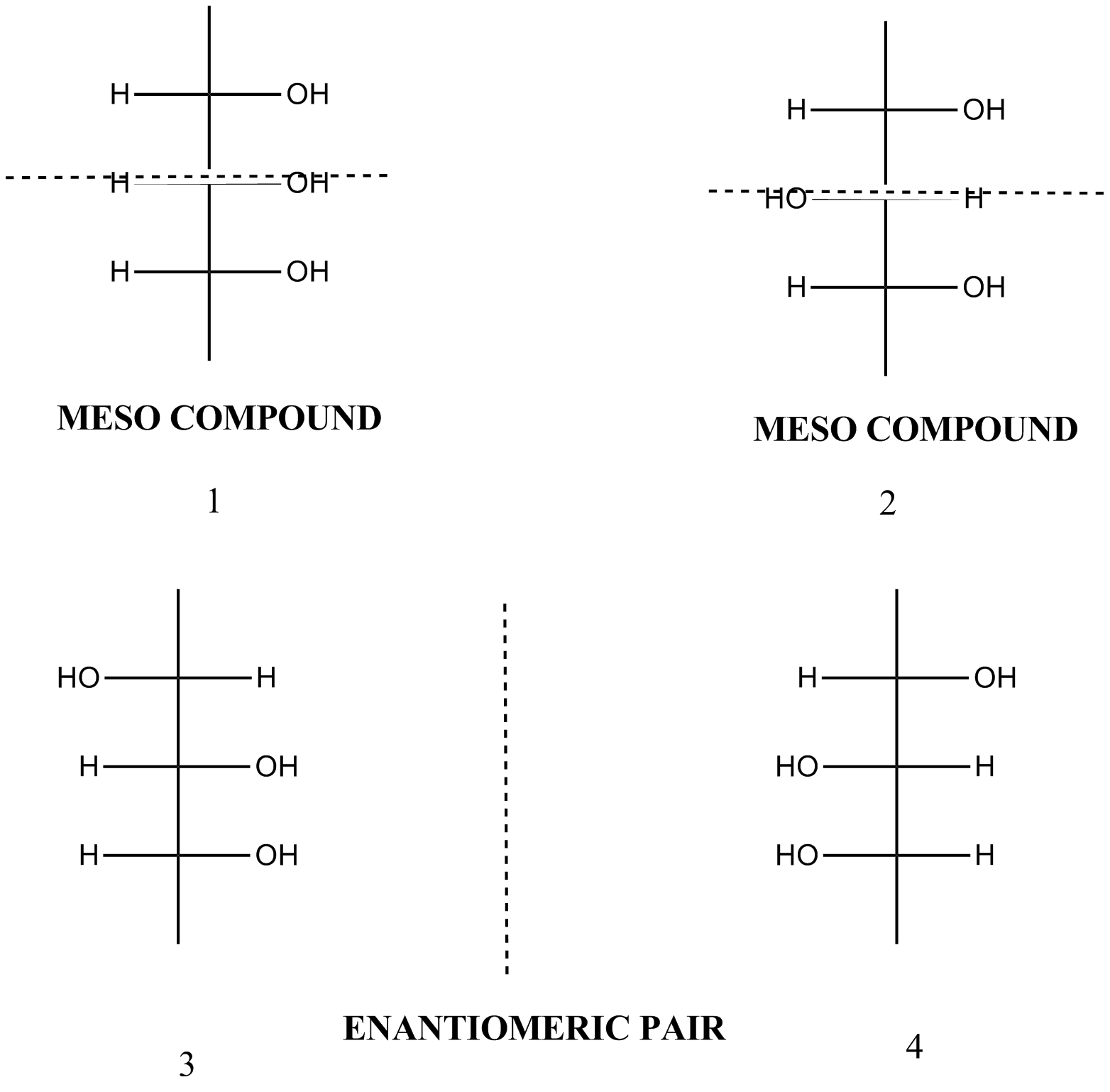
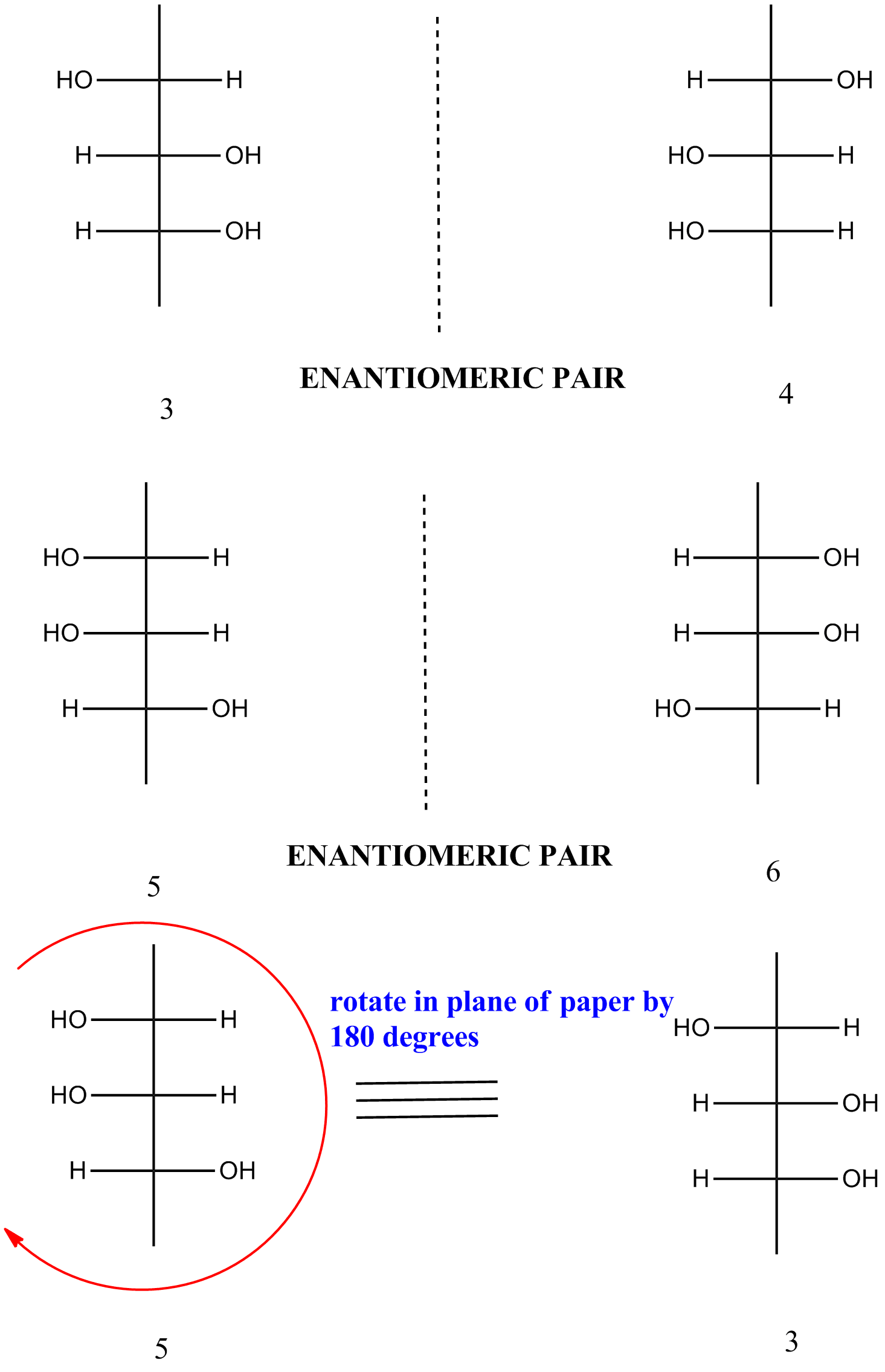
The answer is apparently 4, but the solution does not make sense.

I can only identify 2 chirality centers (the central carbon cannot be a chirality center) so I thought 2^2 = 4 stereoisomer possibilities, but because of the existence of a plane of symmetry, there is a meso compound: thus there should only be 3 stereoisomers.

However, the solution claims that initially, we can deduce 3 chirality centers and claim 8 stereoisomers, then eliminate some of them through the observation of plains of symmetry (there is only one as far as I can see either way). This solution does not make sense, but when I checked by looking at all of the structures, it seemed true. Can someone help me what I am missing?

enter image description here

Improve this question
$\endgroup$
7
  • 5
    $\begingroup$ Central C is chiral when the configuration around the external ones is not the same. $\endgroup$
    – Alchimista
    Commented Jul 26, 2017 at 23:48
  • $\begingroup$ Why someone did downvoted my comment? It is obvious that a C atom between two fragments with different configurations is a chiral center. Obviously if the two remaining substituents differ from each other. I really do not see the reason for such complicated answers with such a simple structure. Especially considering that the question is based on assuming that the molecule has only two chirality centers when indeed, as I explained in my comment, there are three. $\endgroup$
    – Alchimista
    Commented Jul 27, 2017 at 14:06
  • 5
    $\begingroup$ @Alchimista it's not possible to downvote comments, what are you talking about? The central carbon can also be a pseudoasymmetric centre - search on here or look for it in the IUPAC gold book $\endgroup$
    – orthocresol
    Commented Jul 27, 2017 at 14:43
  • $\begingroup$ Not a real problem @Orthocresol.Does not matter much... :) I thought that is not possible but I was convinced that I read that and I was wondering why. I wanted to made clear to the self-teaching student why he got stuck with the max number of stereocenters and consequently the max number of stereisomers. It did not seemed to have problems with identifying symmetry elements, as he said that after seeing the structure he agreed with the exercise book. $\endgroup$
    – Alchimista
    Commented Jul 27, 2017 at 14:51
  • $\begingroup$ Related: Number of diastereomer pairs of 1,3-dichloro-1,2,3-triphenylpropane $\endgroup$
    – user7951
    Commented Jul 27, 2017 at 15:36

4 Answers 4

Reset to default
8
$\begingroup$

It is easier to compare molecules is you rotate them all into a comparable position. Say 1 with the central C-3 hydroxyl group out of the plane of the screen/page like in 1, 4, 5 and 7.

So when we rotate a molecule by 180 degrees along a vertical axis in the plane of the paper, something going into the plane of the page on one end off the molecule rotates to become something coming out of the plane of the page on the other end of the molecule. Also central group flips from into to out of the page and vice-versa.

Hence we can tell that actually pairs (1,2), (3,4), (5,8) and (6,7) are the same molecule, just rotated by 180 degrees.

The absolute positions of the three centres doesn't matter, only the relative orientation of the end two to the central site.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Also, adding to ans of @user213305, molecule number (5,7) and (6,8) are enantiomeric. Hope it helps $\endgroup$
    – user206007
    Commented May 26, 2019 at 10:25
4
$\begingroup$

It seems to me that your question is motivated by considering the central C atom as having two identical substituents. However, the fact that the two substituents have their own R or S makes them not identical, at least not always (only conformational differences do not reflect to the optical ones).

When the two sides of the molecule are not in the same configuration, it easy to see that the central atom is a stereocenter, too.

From here you proceed with max number of enantiomers = 2^n and so on, in which the previous answer might help you in how to identify symmetry elements - if you do not see them at a glance.

In this situation, the carbon is referred to as a pseudoasymmetric centre.

Improve this answer
$\endgroup$
3
$\begingroup$

Stereoisomerism comes by the atoms making up the isomers, joined in the same order, but still manage to have a different spatial arrangement.

Here is the molecule projected in space with 3D axis and are you able to see now if there's a plane of symmetry or not? NOTE: this is not the mirror image. Rotation of its mirror image does not generate the original structure. To superimpose the mirror images, bonds must be broken and reformed.

And you can see if you do spatial arrangements for all other structures in your question, they do have mirror images that are superimposable.

enter image description here

EDIT

I was trying to get a mirror image of the molecule using the application, but it doesn't turn out well. So I leave just the molecule here.

enter image description here

Improve this answer
$\endgroup$
0
2
$\begingroup$

enter image description here

The above drawn structures are 4 stereoisomers of pent 2,3,4,triol.

Note that structure 1 and 2 are meso compounds and therefore do not possess mirror images.

Structures 3 and 4 are an enentiomeric pair . Now you have 4 stereoisomers.

I am quoting from http://www.chem.ucalgary.ca/courses/351/Carey5th/Ch07/ch7-7.html.

"When relating one Fischer projection to another, it's important to realise that it may only be manipulated within the 2D plane in which it is drawn (that is, it may not be arbitrarily rotated within 3D space), and even then, it can only rotated in the plane it is drawn (2D) by 180 degrees ".

If structure 5 is rotated by 180 degrees in the plane of paper then we get structure 3.

Similarly if structure 6 is rotated by 180 degrees in the plane of paper then we get structure 4.

Therefore we get on;y 4 isomers.

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.