Is this what an orbital means?

Question

In this picture, there is a $1s$ and a $2s$ orbital. Are the orbitals only the dark gray circles ? Or are the light gray circles (which are surrounding the dark gray ones on both sides) also included in the orbitals? Other than these two types of circles , there are the nodes which never include any electrons. And since people define an orbital as a region where electrons are most likely to be found, this must mean that the orbitals are only the gray circles because if they included the light gray circles too, then the probability of finding electrons in an orbital would be 100%.

Answer 1

This picture is quite bad, since there are patterns inside the gray areas which shouldn't be there but the gray areas are the orbitals. You could think of it like writing down the position of an electron over and over again and every dot is a position where you found the electron. More dots means it was in that area more often (=higher probability of finding it there).

An electron, theoretically, can be everywhere except the nodes. So an electron from an atom right in front of you could be lightyears away. The chance is very, very, very low for that to happen but it is possible. So for having a 100% chance of finding the electron you have to look in the whole universe, of course it has to be somewhere. That's not very useful at all, since every electrons can be found somewhere in the universe so we don't work with 100% chance but rather with 95% ( or 99% or 99.9%,..) since that's already a probability of finding the electron very, very close to the core of the atom and it is there most of the time.

Answer 2

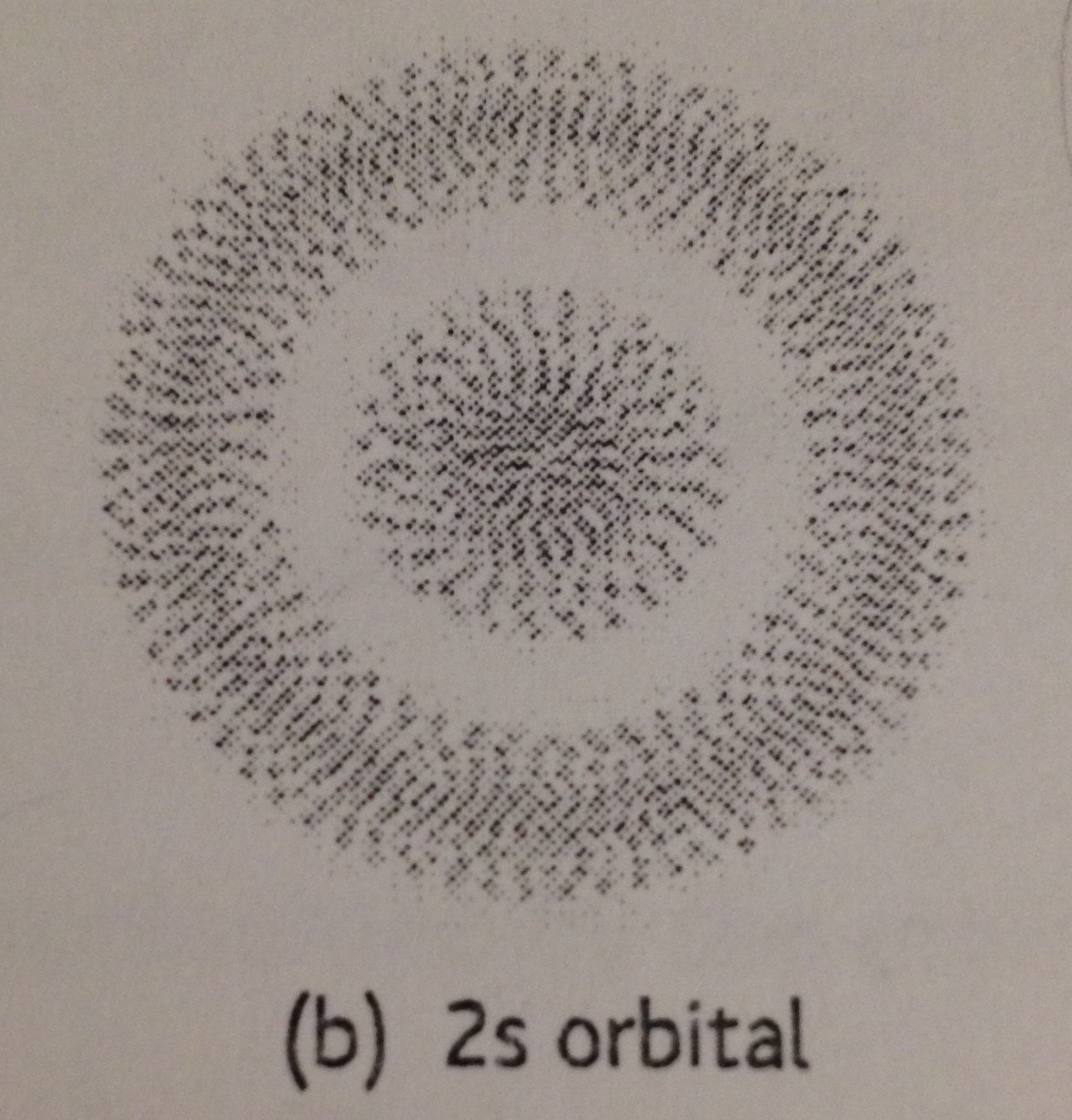
That is not a $1s$ orbital and a $2s$ orbital, the two together make up the $2s$ orbital. If you take a look at the radial distribution function versus atomic distance for a $2s$ orbital, you'll see that there are two peaks and a node between them.

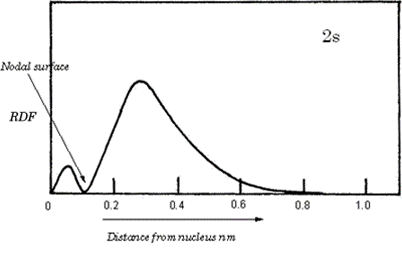
The graphic you presented is (rather poorly) illustrating the above graph of the RDF, with darkness corresponding to the magnitude of the RDF at a given radius. It's useless to try and cut it up so cleanly, though. Electrons don't orbit the nucleus in a well-defined path like the planets orbit the sun—they can be literally anywhere except the node. The only 100% chance of finding an electron in an orbital is by integrating over all space (from center of the nucleus to infinity, in all directions).

---

The orbital is not where the electron is most likely to be found, it is the region of space that it occupies as described by a mathematical function (its wave equation). In order to understand why we treat quantum mechanics the way we do, I would strongly recommend reading up on the the uncertainty principle as well as wave-particle duality (though work through the math you'll need integral calculus, linear algebra, differential equations, and some probability theory). In essence, we can't really know everything about electrons for sure, but what we can know is the probability that they are a certain way at any given time.