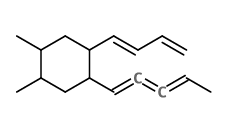
How many stereoisomers are possible for the compound in picture?

Question

How many stereoisomers are possible for the compound in picture? I'm getting 8. Is that correct?

My approach was as follows: The left hand methyl groups may be both up or one up and one down. For each of these cases the groups on the right may be in $(E{,}Z'),(E'{,}Z),(E{,}E'),(Z{,}Z')$ configurations. So $4\cdot2=8$.

I think it is quite evident that carbon having 2 π bonds must be linear. I apologize for the non-linearity in the picture. I hope you will be able to understand the question otherwise.

Answer 1

Follow these steps:

1. Identify all possible sources of stereochemistry. You got pretty far.

2. Determine how many isomers can derive from each. I think you went wrong here.

3. Do you have symmetry? Reduce accordingly.

4. Multiply all the numbers together.

In your molecule:

1. Double bonds:

   • the internal double bond in the residue on the top right
   • the cumulene, which also shows isomerism (E/Z for uneven double bond counts, aR/aS for even ones).

   Asymmetric carbons:

   • the top methyl group
   • the bottom methyl group
   • the cumulene-containing side-chain
   • the other unsaturated side-chain.

2. Every one of these can be either R/S or E/Z.

3. The molecule does not have any elements of symmetry, so we cannot reduce.

4. All we have are factors of 2; two from the double bonds, another four from the asymmetric carbons. This leads us to: $$n(\text{isomers}) = 2^{(2 + 4)} = 2^6 = 64$$

Answer 2

The cyclohexane ring contains 4 chiral centers, one for each carbon in the ring bearing a substituent. Also, the ring shows no plane of symmetry.

The diene moiety (upper right) contains 2 double bonds which could each be E or Z, but the terminal double bond has only one substituent, in other words it contains a $\ce{CH2}$ group, so there is no stereoisomer.

Now the fun part. The lower right moiety (which is quite unusual, btw) also has 2 stereoisomers. The $\ce{CH=C=C=C}$ is linear but its bonds cannot rotate freely. In fact, we could consider it as a simple double bond and the terminal methyl group can be E or Z compared to the cyclohexane ring.

There are 6 stereogenic centers so there are $2^6=64$ stereoisomers.