d-orbital splittings in WS2 monolayer

Question

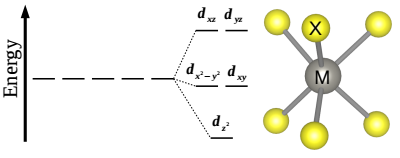
The formerly degenerate $d$ orbitals of the tungsten atoms in the $\ce{WS2}$ monolayer are split into three groups: (1) $\mathrm{d}_{z^2}$, (2) $\mathrm{d}_{x^2-y^2}, \mathrm{d}_{xy}$ and (3) $\mathrm{d}_{xz}, \mathrm{d}_{yz}$ in the order of increasing energy with the large band gap between the first and the second groups.

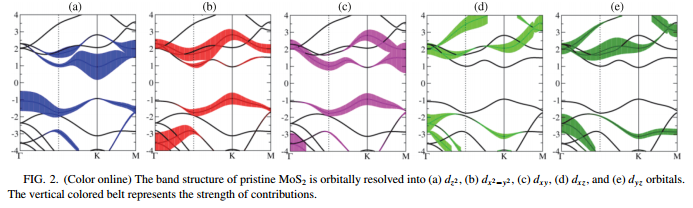
Now the valence band maximum consists dominantly of $\mathrm{d}_{x^2-y^2}, \mathrm{d}_{xy}$ orbitals, whereas the conduction band minimum is composed mainly of $\mathrm{d}_{z^2}$ metal orbital.

So, the order of $\mathrm{d}$ orbitals in the first two splitting groups now is reversed, since the valence band is lower in energy than the conduction band. Where is the correspondence between these two facts?

Answer 1

One vague explanation I came up with is the following.

A prismatic arrangement of atoms (first figure) does not capture fully all symmetries of a hexagonal arrangement in a crystal lattice (which is the case of the second figure). However, the most overlap (in terms of the number of symmetries) between the two occurs at the $\Gamma$ point, since it is the point of the highest symmetry. Thus, the first figure resembles mostly the orbitals at the $\Gamma$ point in the first Brillouin zone of the crystal lattice. Please, if I am thinking in a right direction, refine my answer to make it more strict and rigid.