At equilibrium, the mass of reactants and products are equal. Does that mean the reaction stops?

Question

This one is troubling me, and I even got it wrong in my exam:

at equilibrium the mass of reactants and products are equal, does that mean the reaction stops?

Please explain this one.

Answer 1

At equilibrium, the reaction continues, but the rate of the forward reaction is equal to the rate of the backward reaction.

Let's consider the first order reaction $\ce{A <=>[k_f][k_b] B}$

So, the rate of the forward reaction is $k_f[A]$, and the rate of the backward reaction is $k_b[B]$. Initially, $[B]=0$, and $[A]$ is high, so the forward reaction is very fast, and the backwards reaction doesn't occur at all. As time passes, $[A]$ decreases and $[B]$ increases, so the forward reaction becomes slower and slower, and the backwards reaction speeds up. At one point, both reactions have equal rates ($k_f[A]=k_b[B]$). Here, both reactions take place, but for every time when an $A$ becomes $B$, another $B$ becomes $A$. The reaction is taking place, just that you don;t notice it since the net effect is zero.

Here's a diagram for how the rates of the reaction proceed for the formation of ammonia. Note that the rates never reach 0:

Answer 2

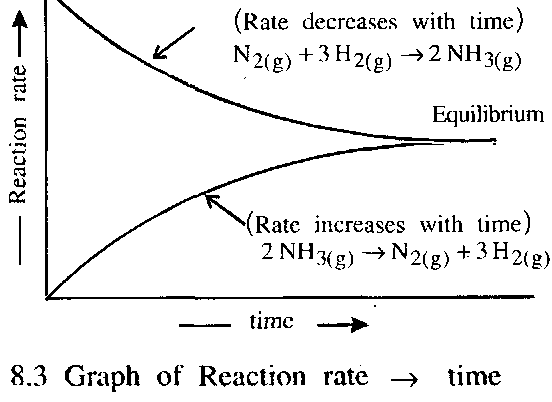
A reaction at equilibrium never stops (by itself), it rather remains in a dynamic equilibrium where forwards and reverse reactions occur at the same rate. However, macroscopically you cannot differentiate between a reaction that stopped and a dynamic equilibrium.

Also another note: It is wrong to say that the masses of reactants and products be equal at equilibrium. You cannot even say that the amounts, concentrations or any other variable is equal for reactants and products. Rather, for each reaction there is a specific ratio of concentrations that is reached at equilibrium, commonly known as the equilibrium constant.

$$\ce{$a$A + $b$B <=> $c$C + $d$D}$$

$$k_\mathrm{eq} = \frac{[\ce{C}]^c [\ce{D}]^d}{[\ce{A}]^a [\ce{B}]^b}$$

The mass ratio at an equilibrium can be anything. Most notably, it can be both $1\,:\,1$ and $10^{99}\,:\,1$ and there is no rule as to which mass ratio would be present at a given equilibrium.

Answer 3

Because at equilibrium state forward and reverse reactions occur at same place but they occur in opposite directions so reaction don't stops..